The-value-of-cosA-cos2A-cos2-2-A-cos-2-n-1-A-where-A-R-may-be-1-1-2-2-3-1-4-sin-2-n-A-2-n-sin-A-

Question Number 18003 by Tinkutara last updated on 13/Jul/17

The value of \cos A \cdot \cos 2 A \cdot {\cos 2}^{2} A \dots . . \cos (2^{n - 1} A),

where A \in R may be

(1) 1

(2) 2

(3) - 1

(4) \frac{\sin 2^{n} A}{2^{n} \sin A}

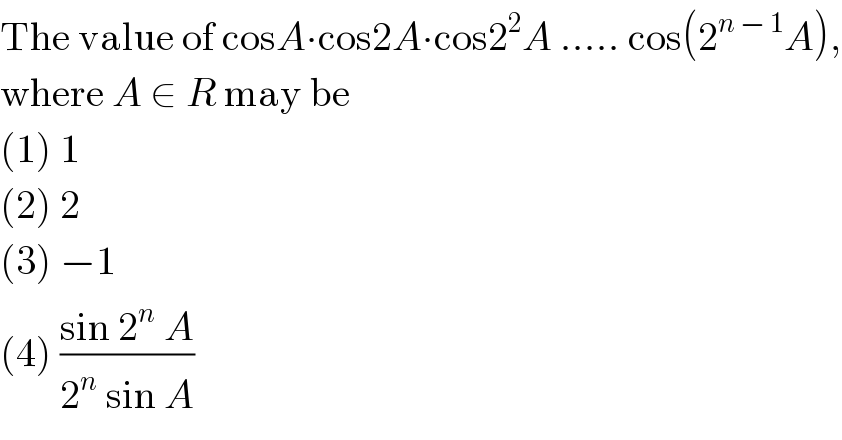
Answered by alex041103 last updated on 13/Jul/17

L e t E = c o s (A) c o s (2 A) \dots = \underset{k = 0}{\prod^{n - 1}} c o s (2^{k} A)

B e c a u s e s i n (2^{k + 1} A) = 2 s i n (2^{k} A) c o s (2^{k} A)

\Rightarrow c o s (2^{k} A) = \frac{s i n (2^{k + 1} A)}{2 s i n (2^{k} A)}

\Rightarrow E = \underset{k = 0}{\prod^{n - 1}} \frac{s i n (2^{k + 1} A)}{2 s i n (2^{k} A)} =

= [\underset{k = 0}{\prod^{n - 1}} s i n (2^{k + 1} A)] \times [\underset{k = 0}{\prod^{n - 1}} \frac{1}{2 s i n (2^{k} A)}]

= \frac{s i n (2^{n} A)}{2 s i n (A)} [\underset{k = 0}{\prod^{n - 2}} s i n (2^{k + 1} A)] [\underset{k = 1}{\prod^{n - 1}} \frac{1}{2 s i n (2^{k} A)}]

= \frac{s i n (2^{n} A)}{2 s i n (A)} [\underset{k = 1}{\prod^{n - 1}} s i n (2^{k} A)] [\underset{k = 1}{\prod^{n - 1}} \frac{1}{2 s i n (2^{k} A)}]

= \frac{s i n (2^{n} A)}{2 s i n (A)} [\underset{k = 1}{\prod^{n - 1}} \frac{s i n (2^{k} A)}{2 s i n (2^{k} A)}]

= \frac{s i n (2^{n} A)}{2 s i n (A)} [\underset{k = 1}{\prod^{n - 1}} \frac{1}{2}]

= \frac{s i n (2^{n} A)}{2 s i n (A)} \times \frac{1}{2^{n - 1}}

= \frac{s u n (2^{n} A)}{2^{n} s i n (A)} = c o s (A) c o s (2 A) \dots

Commented by Tinkutara last updated on 13/Jul/17

But which options are correct ? It can

have more than one option (s) .

Commented by alex041103 last updated on 13/Jul/17

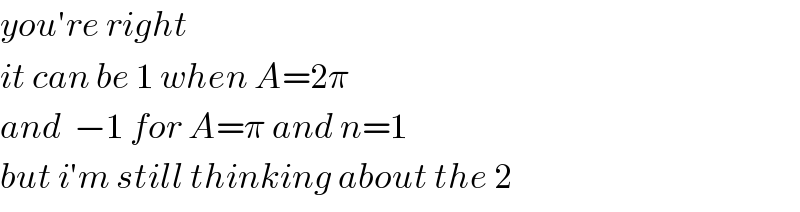
{y o u}^{'} r e r i g h t

i t c a n b e 1 w h e n A = 2 π

a n d - 1 f o r A = π a n d n = 1

b u t i^{'} m s t i l l t h i n k i n g a b o u t t h e 2

Commented by Tinkutara last updated on 13/Jul/17

Thanks Sir!