The-values-of-a-for-which-y-ax-2-ax-1-24-and-x-ay-2-ay-1-24-touch-each-other-are-1-2-3-2-3-2-3-13-601-12-4-13-601-12-

Question Number 39384 by rahul 19 last updated on 05/Jul/18

The values of a for which y = a x^{2} + a x + \frac{1}{24}

a n d x = {a y}^{2} + a y + \frac{1}{24} t o u c h e a c h o t h e r

a r e

1) \frac{2}{3} 2) \frac{3}{2}

3) \frac{13 + \sqrt{601}}{12} 4) \frac{13 - \sqrt{601}}{12} .

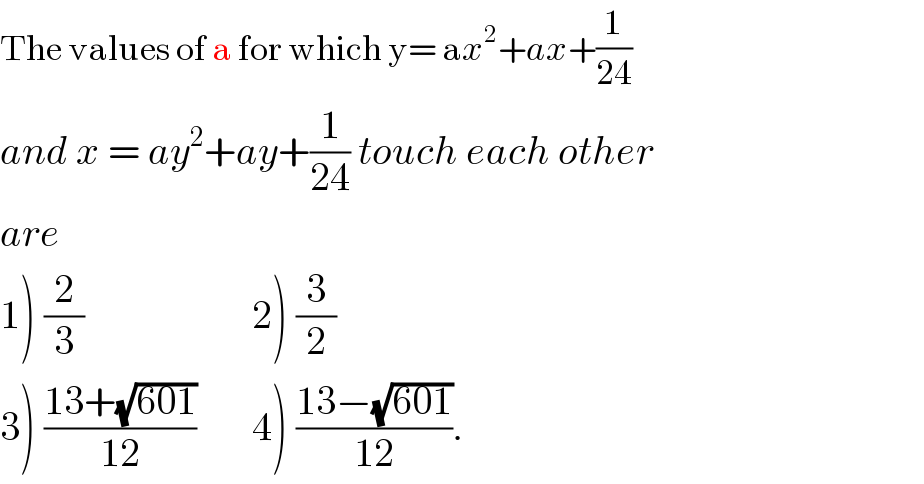
Answered by ajfour last updated on 05/Jul/18

t h e y w i l l t o u c h o n y = x

S o y = x w i l l b e t a n g e n t t o b o t h .

\Rightarrow x = {a x}^{2} + a x + \frac{1}{24} h a s a d o u b l e

r o o t .

\Rightarrow 6 {(a - 1)}^{2} = a

\Rightarrow 6 a^{2} - 13 a + 6 = 0

a = \frac{13 \pm \sqrt{169 - 144}}{12} = \frac{3}{2}, \frac{2}{3} .

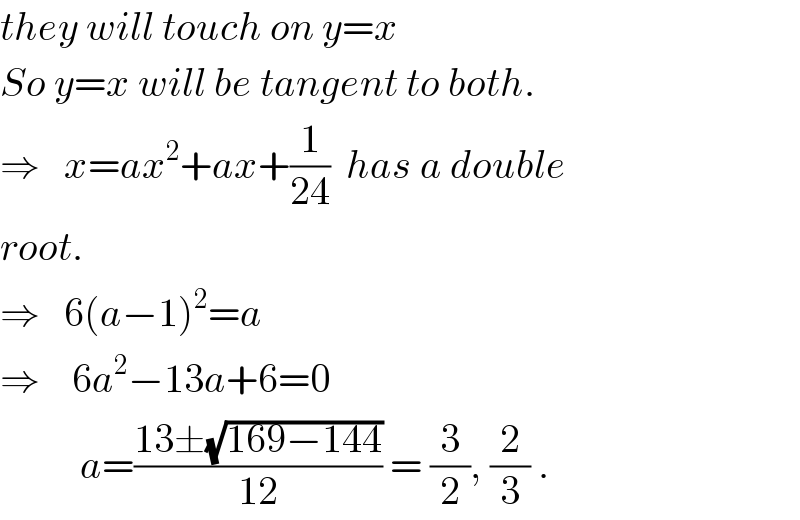
Commented by MJS last updated on 05/Jul/18

master {you}^{'} ve been faster \dots

Commented by rahul 19 last updated on 06/Jul/18

Thank you sir!

Answered by MJS last updated on 05/Jul/18

“ {touching}^{″} means only 1 intersection point

f (x) = {a x}^{2} + a x + \frac{1}{24}

f^{- 1} (x) : x = {a y}^{2} + a y + \frac{1}{24}

so they must have the common tangent y = x

{a x}^{2} + (a - 1) x + \frac{1}{24} = 0 must have exactly 1 solution \Rightarrow

\Rightarrow B^{2} - 4 A C = 0 \Rightarrow {(a - 1)}^{2} - \frac{1}{6} a = 0

a^{2} - \frac{13}{6} a + 1 = 0

o = \frac{13}{12} \pm \frac{5}{12}

a_{1} = \frac{2}{3}; a_{2} = \frac{3}{2}

Commented by rahul 19 last updated on 06/Jul/18

Thank you sir!