Question Number 157739 by gsk2684 last updated on 27/Oct/21
$${two}\:{parallel}\:{paths}\:\mathrm{26}{m}\:{apart}\:{run} \\ $$$${east}−{west}\:{through}\:{the}\:{woods}. \\ $$$${one}\:{person}\:{east}\:{on}\:{one}\:{path}\:{at}\: \\ $$$$\mathrm{3}\:{kmph}\:{and}\:{another}\:{west}\:{on}\:{other}\: \\ $$$${path}\:{at}\:\mathrm{4}\:{kmph}.\:{if}\:{they}\:{pass}\:{each}\: \\ $$$${other}\:{at}\:{time}\:{t}\:=\mathrm{0}\:,\: \\ $$$$\left.{i}\right){how}\:{far}\:{apart}\:{are}\:{they} \\ $$$$\mathrm{8}\:{sec}\:{later}?\: \\ $$$$\left.{ii}\right)\:{how}\:{fast}\:{is}\:{the}\: \\ $$$${distance}\:{between}\:{them}\:{changing}\: \\ $$$${at}\:{that}\:{mlment}?\: \\ $$$$\left.{iii}\right){find}\:{the}\:{distance}\:{L} \\ $$$${between}\:{them}\:{at}\:\mathrm{8}\:{sec}\:{and}\:\frac{{dL}}{{dt}}? \\ $$
Commented by gsk2684 last updated on 27/Oct/21
$${could}\:{you}\:{pls}\:{answer}\:{this}. \\ $$
Answered by Kunal12588 last updated on 27/Oct/21
$${a}\:{time}\:=\:{t} \\ $$$${position}\:{of}\:{first}\:{person}=\mathrm{3}{t}\:\hat {{i}} \\ $$$${position}\:{of}\:{second}\:{person}=−\mathrm{4}{t}\:\hat {{i}} \\ $$$${distance}\:{between}\:{them}\:=\:\mid\mathrm{3}{t}−\left(−\mathrm{4}{t}\right)\mid=\mathrm{7}{t} \\ $$$$\left({assuming}\:'{t}'\:{is}\:{positive}\right) \\ $$$$ \\ $$$$\left({i}\right)\:{at}\:{t}=\mathrm{8} \\ $$$${distance}\:{between}\:{them}=\:\mathrm{7}×\mathrm{8}=\mathrm{56}\:{km} \\ $$$$\left({ii}\right)\:\frac{{d}}{{dt}}\left(\mathrm{7}{t}\right)=\mathrm{7}\:{kmph}.\:{irrespective}\:{of}\:{the}\:{time} \\ $$$$\left({iii}\right){L}=\mathrm{56}\:{km}\:{and}\:\frac{{dL}}{{dt}}=\mathrm{7}{kmph} \\ $$
Commented by gsk2684 last updated on 28/Oct/21
$${thanks}\: \\ $$$${and}\:{it}\:{is}\:{guven}\:{that}\:{the}\:{paths}\:{are} \\ $$$${parallel}\:{not}\:{on}\:{the}\:{same}\:{line}. \\ $$$${and}\:{they}\:{apart}\:\mathrm{26}{m}. \\ $$
Commented by Kunal12588 last updated on 29/Oct/21
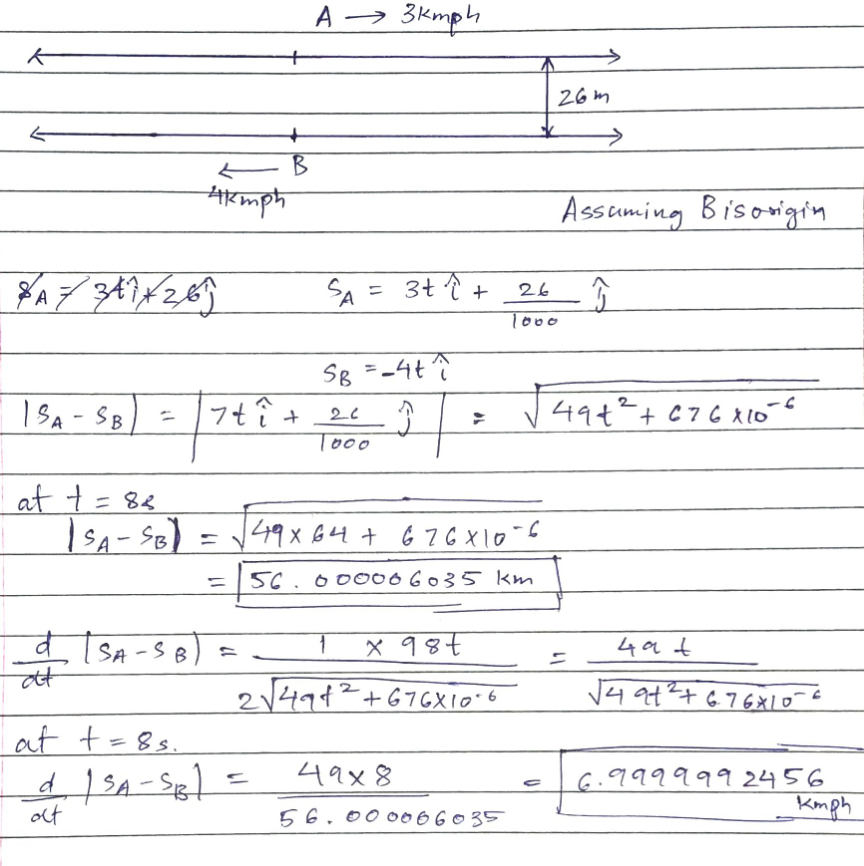
Commented by gsk2684 last updated on 30/Oct/21
$${thanks}\:\left(°\:\smile°\right) \\ $$