Question Number 37945 by NECx last updated on 19/Jun/18

Commented by MrW3 last updated on 20/Jun/18
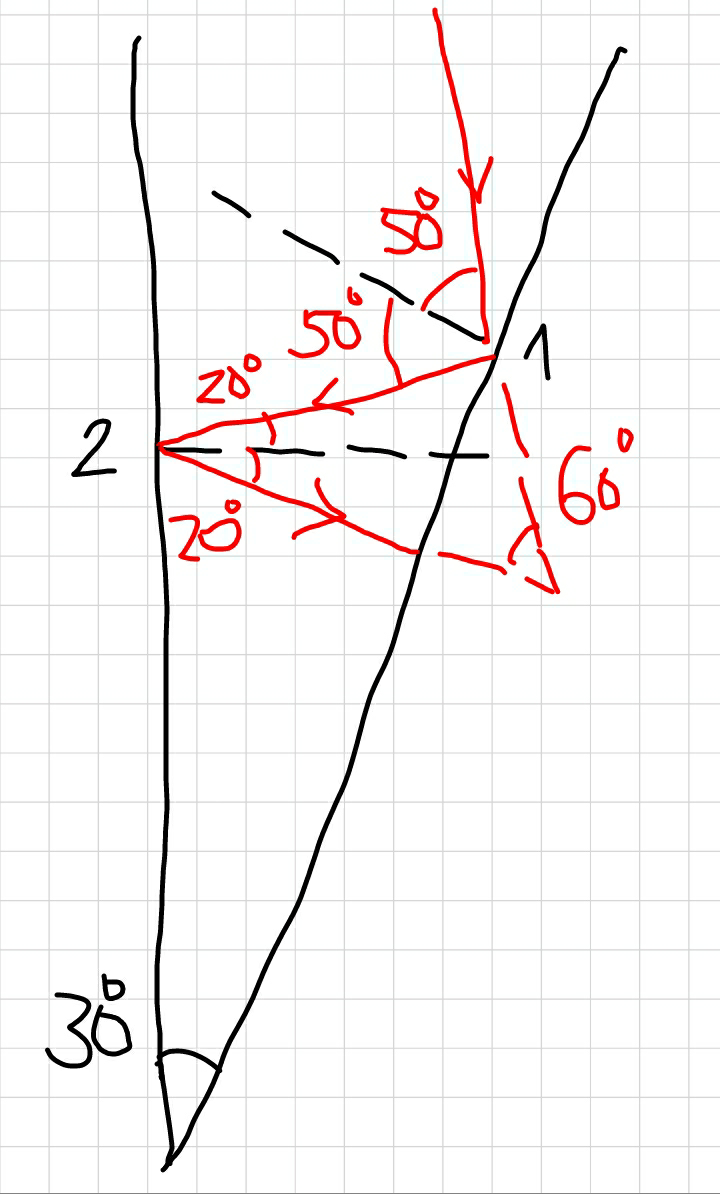
Commented by MrW3 last updated on 20/Jun/18
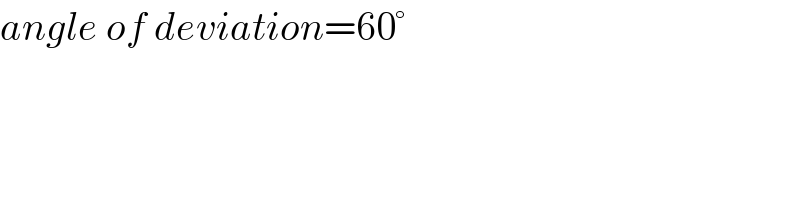
Commented by MrW3 last updated on 20/Jun/18

Commented by MrW3 last updated on 20/Jun/18
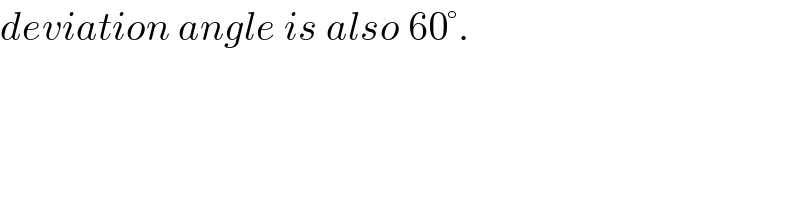
Commented by NECx last updated on 20/Jun/18
Commented by NECx last updated on 20/Jun/18
Commented by MrW3 last updated on 21/Jun/18

Commented by MrW3 last updated on 21/Jun/18

Commented by NECx last updated on 22/Jun/18

Commented by MrW3 last updated on 23/Jun/18