Question Number 56838 by necx1 last updated on 25/Mar/19

$${What}\:{would}\:{be}\:{the}\:{diameter}\:{of}\:{a}\:{circle} \\ $$$${having}\:{a}\:{heptagon}\:{of}\:{sides}\:\mathrm{45}{m},\mathrm{60}{m}, \\ $$$$\mathrm{60}{m},\mathrm{50}{m},\mathrm{40}{m},\mathrm{45}{m}\:{and}\:\mathrm{50}{m}\:{inscribed} \\ $$$${in}\:{it}? \\ $$
Answered by MJS last updated on 25/Mar/19
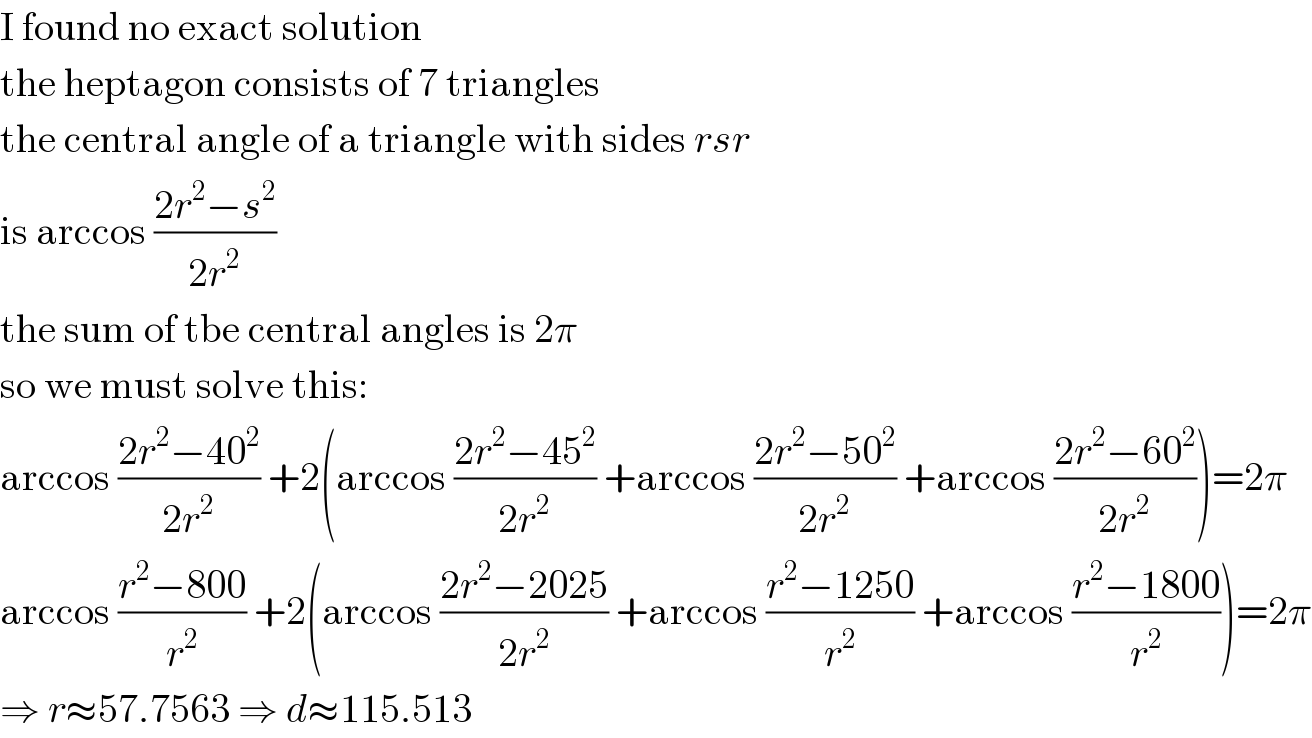
$$\mathrm{I}\:\mathrm{found}\:\mathrm{no}\:\mathrm{exact}\:\mathrm{solution} \\ $$$$\mathrm{the}\:\mathrm{heptagon}\:\mathrm{consists}\:\mathrm{of}\:\mathrm{7}\:\mathrm{triangles} \\ $$$$\mathrm{the}\:\mathrm{central}\:\mathrm{angle}\:\mathrm{of}\:\mathrm{a}\:\mathrm{triangle}\:\mathrm{with}\:\mathrm{sides}\:{rsr} \\ $$$$\mathrm{is}\:\mathrm{arccos}\:\frac{\mathrm{2}{r}^{\mathrm{2}} −{s}^{\mathrm{2}} }{\mathrm{2}{r}^{\mathrm{2}} } \\ $$$$\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{tbe}\:\mathrm{central}\:\mathrm{angles}\:\mathrm{is}\:\mathrm{2}\pi \\ $$$$\mathrm{so}\:\mathrm{we}\:\mathrm{must}\:\mathrm{solve}\:\mathrm{this}: \\ $$$$\mathrm{arccos}\:\frac{\mathrm{2}{r}^{\mathrm{2}} −\mathrm{40}^{\mathrm{2}} }{\mathrm{2}{r}^{\mathrm{2}} }\:+\mathrm{2}\left(\mathrm{arccos}\:\frac{\mathrm{2}{r}^{\mathrm{2}} −\mathrm{45}^{\mathrm{2}} }{\mathrm{2}{r}^{\mathrm{2}} }\:+\mathrm{arccos}\:\frac{\mathrm{2}{r}^{\mathrm{2}} −\mathrm{50}^{\mathrm{2}} }{\mathrm{2}{r}^{\mathrm{2}} }\:+\mathrm{arccos}\:\frac{\mathrm{2}{r}^{\mathrm{2}} −\mathrm{60}^{\mathrm{2}} }{\mathrm{2}{r}^{\mathrm{2}} }\right)=\mathrm{2}\pi \\ $$$$\mathrm{arccos}\:\frac{{r}^{\mathrm{2}} −\mathrm{800}}{{r}^{\mathrm{2}} }\:+\mathrm{2}\left(\mathrm{arccos}\:\frac{\mathrm{2}{r}^{\mathrm{2}} −\mathrm{2025}}{\mathrm{2}{r}^{\mathrm{2}} }\:+\mathrm{arccos}\:\frac{{r}^{\mathrm{2}} −\mathrm{1250}}{{r}^{\mathrm{2}} }\:+\mathrm{arccos}\:\frac{{r}^{\mathrm{2}} −\mathrm{1800}}{{r}^{\mathrm{2}} }\right)=\mathrm{2}\pi \\ $$$$\Rightarrow\:{r}\approx\mathrm{57}.\mathrm{7563}\:\Rightarrow\:{d}\approx\mathrm{115}.\mathrm{513} \\ $$
Commented by necx1 last updated on 27/Mar/19

$${hmmm} \\ $$
