Question Number 95424 by john santu last updated on 25/May/20

$$\mathrm{without}\:\mathrm{calculator}\: \\ $$$$\mathrm{tan}\:^{\mathrm{2}} \mathrm{36}^{\mathrm{o}} \:×\:\mathrm{tan}\:^{\mathrm{2}} \mathrm{72}^{\mathrm{o}} \:? \\ $$
Commented by PRITHWISH SEN 2 last updated on 26/May/20

$$\mathrm{tan}\:\mathrm{2Atan}\:\mathrm{A}=\mathrm{sec}\:\mathrm{2A}−\mathrm{1} \\ $$$$\mathrm{tan}\:\mathrm{72tan}\:\mathrm{36}=\mathrm{sec}\:\mathrm{72}−\mathrm{1}=\frac{\mathrm{1}}{\mathrm{sin}\:\mathrm{18}}−\mathrm{1}=\sqrt{\mathrm{5}}+\mathrm{1}−\mathrm{1}=\sqrt{\mathrm{5}} \\ $$
Answered by john santu last updated on 25/May/20
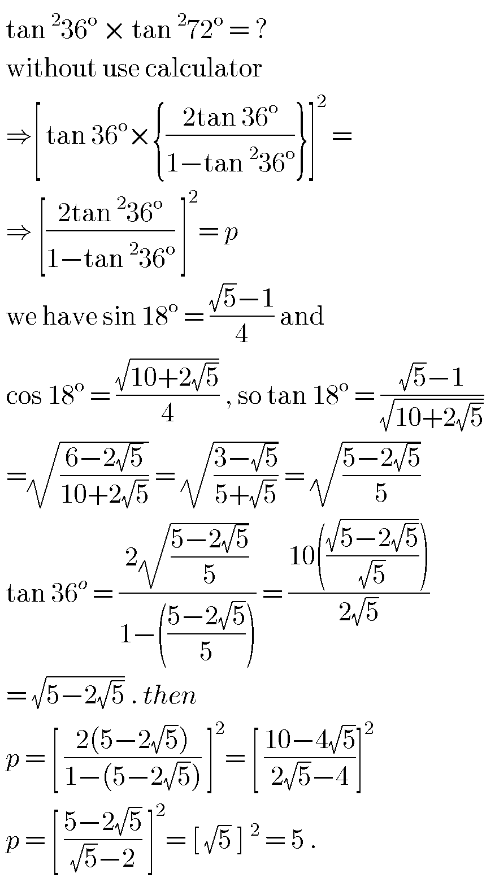
Commented by john santu last updated on 25/May/20

$$\mathrm{very}\:\mathrm{length}\:\mathrm{hahaha}… \\ $$
Answered by bobhans last updated on 25/May/20
![in my way tan 36^o ×tan 72^o = ((2sin^2 36^o cos 36^o )/(cos 36^o cos 72^o )) = ((2−2cos^2 36^o )/(2cos^2 36^o −1)) = (1/(2cos^2 36^o −1))−1 = (1/(2((((√5)+1)/4))^2 −1)) −1 = (√5) so tan^2 36 × tan^2 72^o = ((√5) )^2 = 5 [ we know cos 36^o = (((√5)+1)/4) ]](https://www.tinkutara.com/question/Q95430.png)
$$\mathrm{in}\:\mathrm{my}\:\mathrm{way}\: \\ $$$$\mathrm{tan}\:\mathrm{36}^{\mathrm{o}} ×\mathrm{tan}\:\mathrm{72}^{\mathrm{o}} \:=\:\frac{\mathrm{2sin}\:^{\mathrm{2}} \mathrm{36}^{\mathrm{o}} \:\mathrm{cos}\:\mathrm{36}^{\mathrm{o}} }{\mathrm{cos}\:\mathrm{36}^{\mathrm{o}} \:\mathrm{cos}\:\mathrm{72}^{\mathrm{o}} } \\ $$$$=\:\frac{\mathrm{2}−\mathrm{2cos}\:^{\mathrm{2}} \mathrm{36}^{\mathrm{o}} }{\mathrm{2cos}\:^{\mathrm{2}} \mathrm{36}^{\mathrm{o}} −\mathrm{1}}\:\:=\:\frac{\mathrm{1}}{\mathrm{2cos}\:^{\mathrm{2}} \mathrm{36}^{\mathrm{o}} −\mathrm{1}}−\mathrm{1} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}\left(\frac{\sqrt{\mathrm{5}}+\mathrm{1}}{\mathrm{4}}\right)^{\mathrm{2}} −\mathrm{1}}\:−\mathrm{1}\:=\:\sqrt{\mathrm{5}}\: \\ $$$$\mathrm{so}\:\mathrm{tan}\:^{\mathrm{2}} \mathrm{36}\:×\:\mathrm{tan}\:^{\mathrm{2}} \mathrm{72}^{\mathrm{o}} \:=\:\left(\sqrt{\mathrm{5}}\:\right)^{\mathrm{2}} \:=\:\mathrm{5} \\ $$$$\left[\:\mathrm{we}\:\mathrm{know}\:\mathrm{cos}\:\mathrm{36}^{\mathrm{o}} \:=\:\frac{\sqrt{\mathrm{5}}+\mathrm{1}}{\mathrm{4}}\:\right]\: \\ $$
Commented by john santu last updated on 25/May/20

$$\mathrm{waw}……\mathrm{joosss} \\ $$
