Question Number 98952 by Ar Brandon last updated on 17/Jun/20
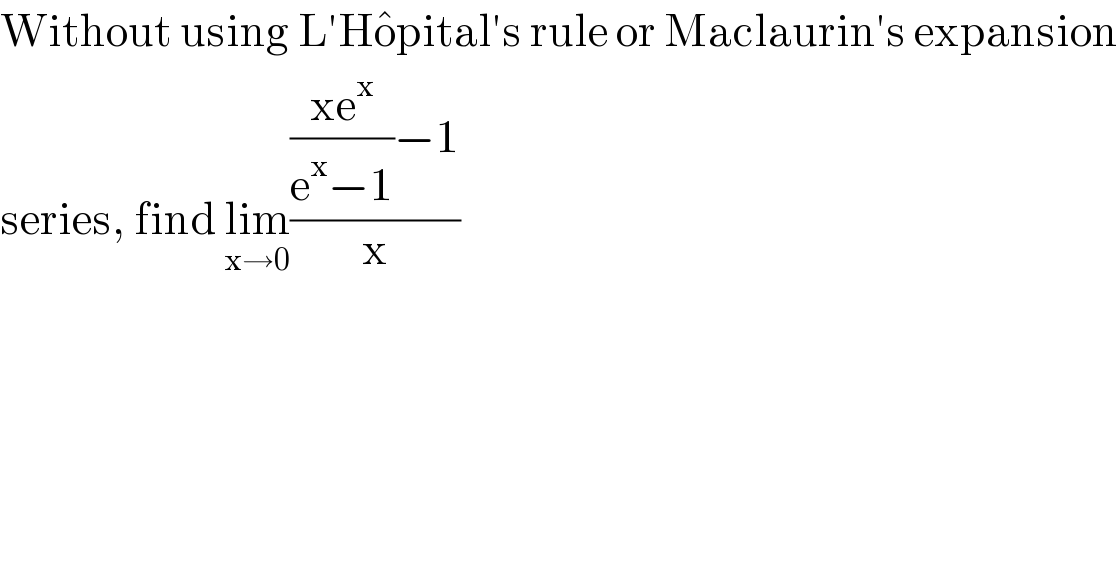
$$\mathrm{Without}\:\mathrm{using}\:\mathrm{L}'\mathrm{H}\hat {\mathrm{o}pital}'\mathrm{s}\:\mathrm{rule}\:\mathrm{or}\:\mathrm{Maclaurin}'\mathrm{s}\:\mathrm{expansion} \\ $$$$\mathrm{series},\:\mathrm{find}\:\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\frac{\mathrm{xe}^{\mathrm{x}} }{\mathrm{e}^{\mathrm{x}} −\mathrm{1}}−\mathrm{1}}{\mathrm{x}} \\ $$
Commented by 675480065 last updated on 17/Jun/20
![let f(x)=((xe^x )/(e^x −1))−1 and g(x)=x from taylor series expansion e^x =1+x+(x^2 /2)+0(x^2 )⇒xe^x =x+x^2 +(x^3 /2)+0(x^3 ) e^x −1=1+x+(x^2 /2)+0(x^2 )−1=x+(x^2 /2)+0(x^2 ) ⇒f(x)=[((x(1+x+(x^2 /2)+0(x^2 )))/(x(1+(x/2)+0(x))))]−1 ⇒f(x)=[((1+x+(x^2 /2)−1−(x/2)+0(x^2 ))/((1+(x/2)+0(x))))]=[((x+x^2 +0(x^2 ))/((2+x+0(x))))] lim_(x→0) (((f(x))/(g(x))))=lim_(x→0) [((1+x+0(x))/(2+x+0(x)))]=(1/2)](https://www.tinkutara.com/question/Q98960.png)
$$\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{xe}^{\mathrm{x}} }{\mathrm{e}^{\mathrm{x}} −\mathrm{1}}−\mathrm{1}\:\mathrm{and}\:\mathrm{g}\left(\mathrm{x}\right)=\mathrm{x} \\ $$$$\mathrm{from}\:\mathrm{taylor}\:\mathrm{series}\:\mathrm{expansion} \\ $$$$\mathrm{e}^{\mathrm{x}} =\mathrm{1}+\mathrm{x}+\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}+\mathrm{0}\left(\mathrm{x}^{\mathrm{2}} \right)\Rightarrow\mathrm{xe}^{\mathrm{x}} =\mathrm{x}+\mathrm{x}^{\mathrm{2}} +\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{2}}+\mathrm{0}\left(\mathrm{x}^{\mathrm{3}} \right) \\ $$$$\mathrm{e}^{\mathrm{x}} −\mathrm{1}=\mathrm{1}+\mathrm{x}+\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}+\mathrm{0}\left(\mathrm{x}^{\mathrm{2}} \right)−\mathrm{1}=\mathrm{x}+\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}+\mathrm{0}\left(\mathrm{x}^{\mathrm{2}} \right) \\ $$$$\Rightarrow\mathrm{f}\left(\mathrm{x}\right)=\left[\frac{\mathrm{x}\left(\mathrm{1}+\mathrm{x}+\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}+\mathrm{0}\left(\mathrm{x}^{\mathrm{2}} \right)\right)}{\mathrm{x}\left(\mathrm{1}+\frac{\mathrm{x}}{\mathrm{2}}+\mathrm{0}\left(\mathrm{x}\right)\right)}\right]−\mathrm{1} \\ $$$$\Rightarrow\mathrm{f}\left(\mathrm{x}\right)=\left[\frac{\mathrm{1}+\mathrm{x}+\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}}−\mathrm{1}−\frac{\mathrm{x}}{\mathrm{2}}+\mathrm{0}\left(\mathrm{x}^{\mathrm{2}} \right)}{\left(\mathrm{1}+\frac{\mathrm{x}}{\mathrm{2}}+\mathrm{0}\left(\mathrm{x}\right)\right)}\right]=\left[\frac{\mathrm{x}+\mathrm{x}^{\mathrm{2}} +\mathrm{0}\left(\mathrm{x}^{\mathrm{2}} \right)}{\left(\mathrm{2}+\mathrm{x}+\mathrm{0}\left(\mathrm{x}\right)\right)}\right] \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\frac{\mathrm{f}\left(\mathrm{x}\right)}{\mathrm{g}\left(\mathrm{x}\right)}\right)=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left[\frac{\mathrm{1}+\mathrm{x}+\mathrm{0}\left(\mathrm{x}\right)}{\mathrm{2}+\mathrm{x}+\mathrm{0}\left(\mathrm{x}\right)}\right]=\frac{\mathrm{1}}{\mathrm{2}} \\ $$
