Question Number 169396 by Mastermind last updated on 29/Apr/22

Commented by mr W last updated on 29/Apr/22

Commented by infinityaction last updated on 29/Apr/22

Commented by mr W last updated on 29/Apr/22

Commented by Mastermind last updated on 29/Apr/22

Commented by Mastermind last updated on 29/Apr/22

Commented by Mastermind last updated on 29/Apr/22

Commented by Mastermind last updated on 29/Apr/22

Commented by mr W last updated on 30/Apr/22
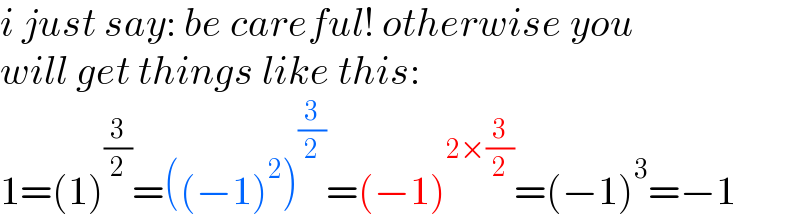
Commented by infinityaction last updated on 30/Apr/22

Commented by mr W last updated on 30/Apr/22
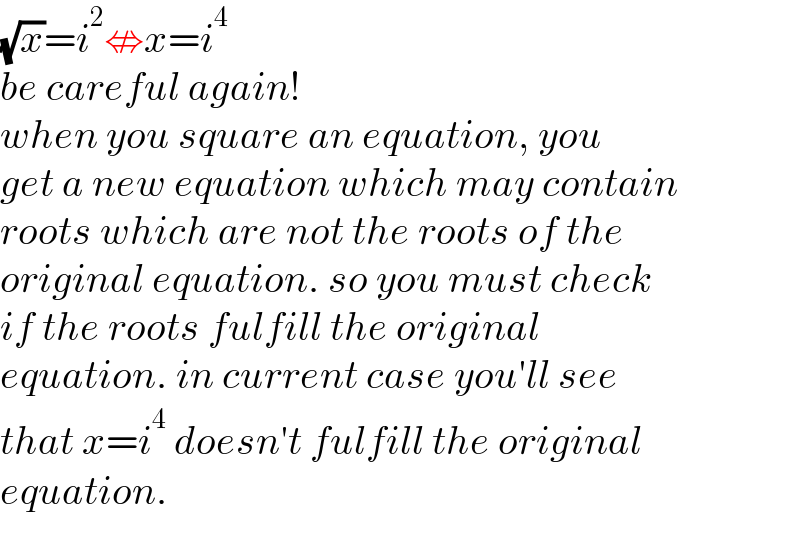
Commented by mr W last updated on 30/Apr/22
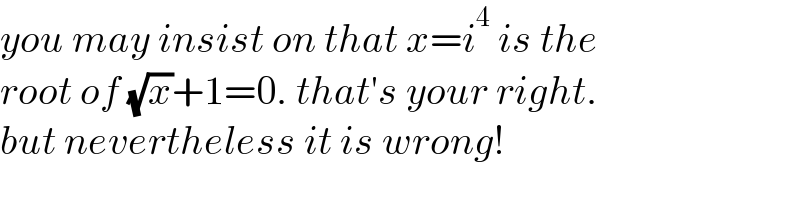
Commented by mr W last updated on 30/Apr/22
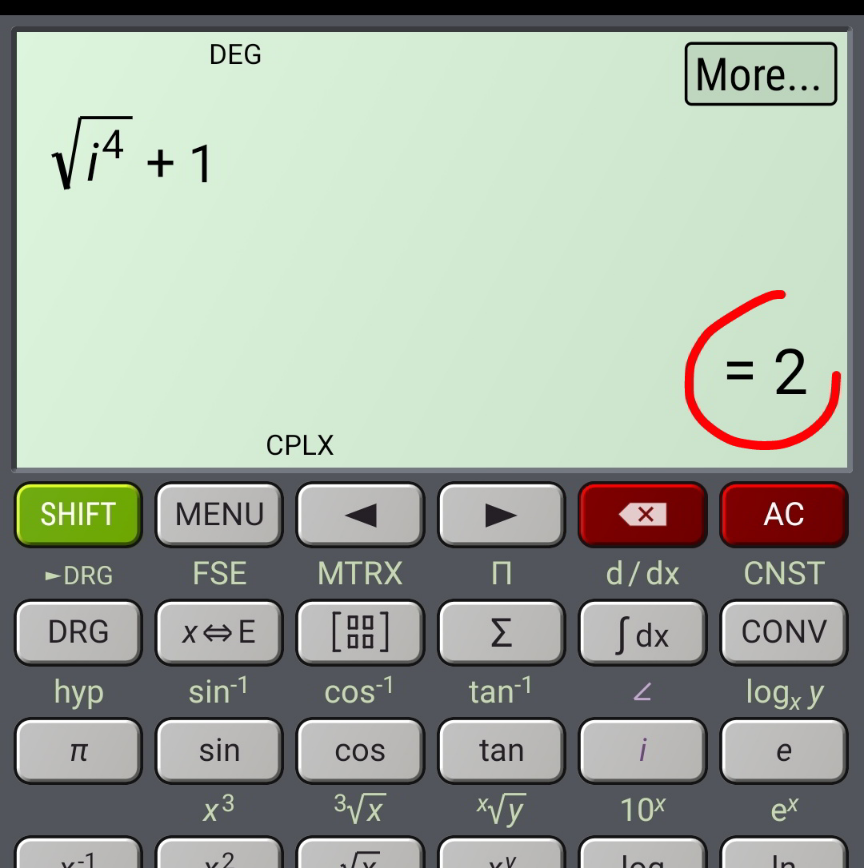
Commented by infinityaction last updated on 30/Apr/22

Commented by MJS_new last updated on 30/Apr/22

