Menu Close

x-1-x-1-x-1-x-1-dx-

Tinku Tara 0 Comments
Pin




Question Number 65015 by aliesam last updated on 24/Jul/19
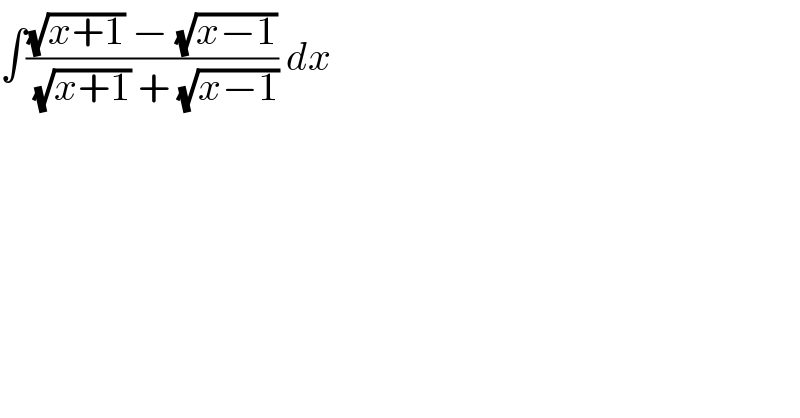
∫(((√(x+1)) − (√(x−1)))/( (√(x+1)) + (√(x−1)))) dx
∫x+1−x−1x+1+x−1dx
Commented by mathmax by abdo last updated on 24/Jul/19
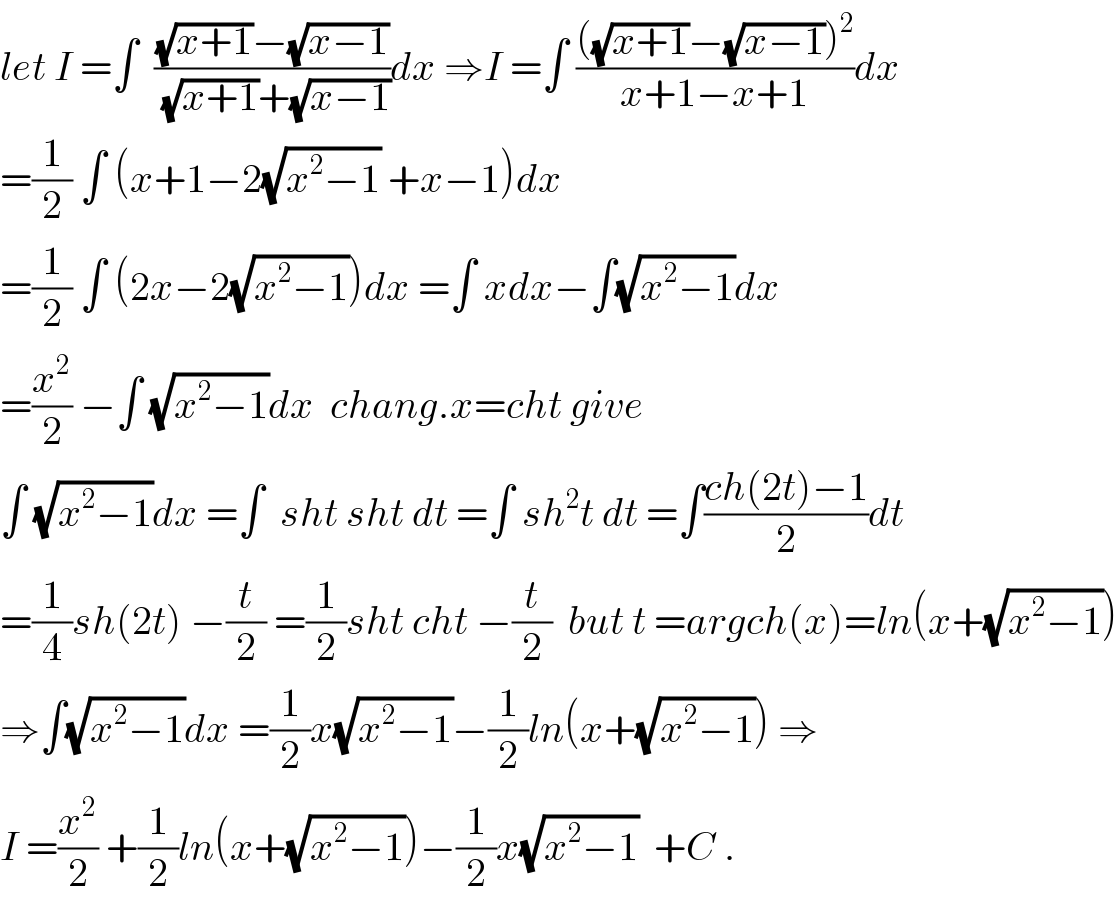
let I =∫ (((√(x+1))−(√(x−1)))/( (√(x+1))+(√(x−1))))dx ⇒I =∫ ((((√(x+1))−(√(x−1)))^2 )/(x+1−x+1))dx =(1/2) ∫ (x+1−2(√(x^2 −1)) +x−1)dx =(1/2) ∫ (2x−2(√(x^2 −1)))dx =∫ xdx−∫(√(x^2 −1))dx =(x^2 /2) −∫ (√(x^2 −1))dx chang.x=cht give ∫ (√(x^2 −1))dx =∫ sht sht dt =∫ sh^2 t dt =∫((ch(2t)−1)/2)dt =(1/4)sh(2t) −(t/2) =(1/2)sht cht −(t/2) but t =argch(x)=ln(x+(√(x^2 −1))) ⇒∫(√(x^2 −1))dx =(1/2)x(√(x^2 −1))−(1/2)ln(x+(√(x^2 −1))) ⇒ I =(x^2 /2) +(1/2)ln(x+(√(x^2 −1)))−(1/2)x(√(x^2 −1)) +C .
letI=∫x+1−x−1x+1+x−1dx⇒I=∫(x+1−x−1)2x+1−x+1dx=12∫(x+1−2x2−1+x−1)dx=12∫(2x−2x2−1)dx=∫xdx−∫x2−1dx=x22−∫x2−1dxchang.x=chtgive∫x2−1dx=∫shtshtdt=∫sh2tdt=∫ch(2t)−12dt=14sh(2t)−t2=12shtcht−t2butt=argch(x)=ln(x+x2−1)⇒∫x2−1dx=12xx2−1−12ln(x+x2−1)⇒I=x22+12ln(x+x2−1)−12xx2−1+C.
Commented by aliesam last updated on 24/Jul/19
thank you sir
thankyousir
Commented by mathmax by abdo last updated on 24/Jul/19
you are welcome.
youarewelcome.
Answered by Tanmay chaudhury last updated on 24/Jul/19
∫((x+1−2(√(x^2 −1)) +x−1)/(x+1−x+1))dx ∫x−(√(x^2 −1)) dx (x^2 /2)−(((x(√(x^2 −1)))/2)−(1/2)ln(x+(√(x^2 −1)) )+c
∫x+1−2x2−1+x−1x+1−x+1dx∫x−x2−1dxx22−(xx2−12−12ln(x+x2−1)+c
Commented by aliesam last updated on 24/Jul/19
thank you sir
thankyousir
Commented by Tanmay chaudhury last updated on 24/Jul/19
most welcome sir
mostwelcomesir

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *