x-1-x-2-x-1-x-2-2-x-

Question Number 79147 by jagoll last updated on 23/Jan/20

\sqrt{x + \frac{1}{x^{2}}} + \sqrt{x - \frac{1}{x^{2}}} ⩽ \frac{2}{x}

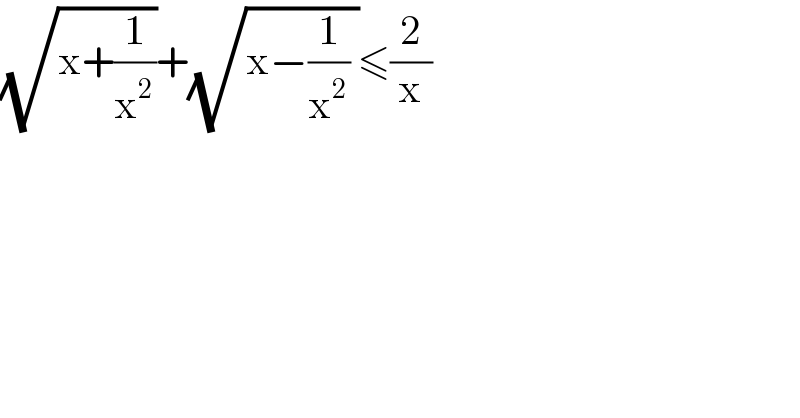
Answered by john santu last updated on 23/Jan/20

\sqrt{\frac{x^{3} + 1}{x^{2}}} + \sqrt{\frac{x^{3} - 1}{x^{2}}} ⩽ \frac{2}{x}

(i) x ⩾ - 1 \land x ⩾ 1 \land x \neq 0

(i i) \frac{\sqrt{x^{3} + 1} + \sqrt{x^{3} - 1}}{x} ⩽ \frac{2}{x}

\Rightarrow \sqrt{x^{3} + 1} + \sqrt{x^{3} - 1} ⩽ 2

l e t x^{3} = t \Rightarrow \sqrt{t + 1} + \sqrt{t - 1} ⩽ 2

2 t + 2 \sqrt{t^{2} - 1} ⩽ 4

\sqrt{t^{2} - 1} ⩽ 2 - t \Rightarrow t^{2} - 1 ⩽ 4 - 4 t + t^{2}

4 t ⩽ 5 \Rightarrow t ⩽ \frac{5}{4} \Rightarrow x^{3} ⩽ \frac{5}{4}

x ⩽ \sqrt[3]{\frac{5}{4}} . n o w w e g e t s o l u t i o n

x ⩾ 1 \land x ⩽ \sqrt[3]{\frac{5}{4}} \Rightarrow x \in [1, \sqrt[3]{\frac{5}{4}}]

Commented by jagoll last updated on 23/Jan/20

thank you sir . your solution is

fantastic

Commented by jagoll last updated on 23/Jan/20

good sir

Commented by MJS last updated on 23/Jan/20

nice and right, but (just saying) :

(i) x ⩾ - 1 \land x ⩾ 1 \land x \neq 0 \Rightarrow x ⩾ 1

squaring without substituting t {isn}^{'} t any harder :

0 ⩽ \sqrt{x^{3} + 1} + \sqrt{x^{3} - 1} ⩽ 2 ∣^{2}

0 ⩽ 2 x^{3} + 2 \sqrt{x^{3} + 1} \sqrt{x^{3} - 1} ⩽ 4

- x^{3} ⩽ \sqrt{x^{3} + 1} \sqrt{x^{3} - 1} ⩽ 2 - x^{3}

but we know that 0 ⩽ \sqrt{x^{3} + 1} \sqrt{x^{3} - 1}

\Rightarrow 0 ⩽ 4 - x^{3} \Rightarrow x ⩽ \sqrt[3]{2}

0 ⩽ \sqrt{x^{3} + 1} \sqrt{x^{3} - 1} ⩽ 2 - x^{3} ∣^{2}

0 ⩽ x^{6} - 1 ⩽ x^{6} - 4 x^{3} + 4

0 ⩽ x^{3} ⩽ \frac{5}{4}

0 ⩽ x ⩽ \sqrt[3]{\frac{5}{4}}

but x ⩾ 1

\Rightarrow

1 ⩽ x ⩽ \sqrt[3]{\frac{5}{4}}

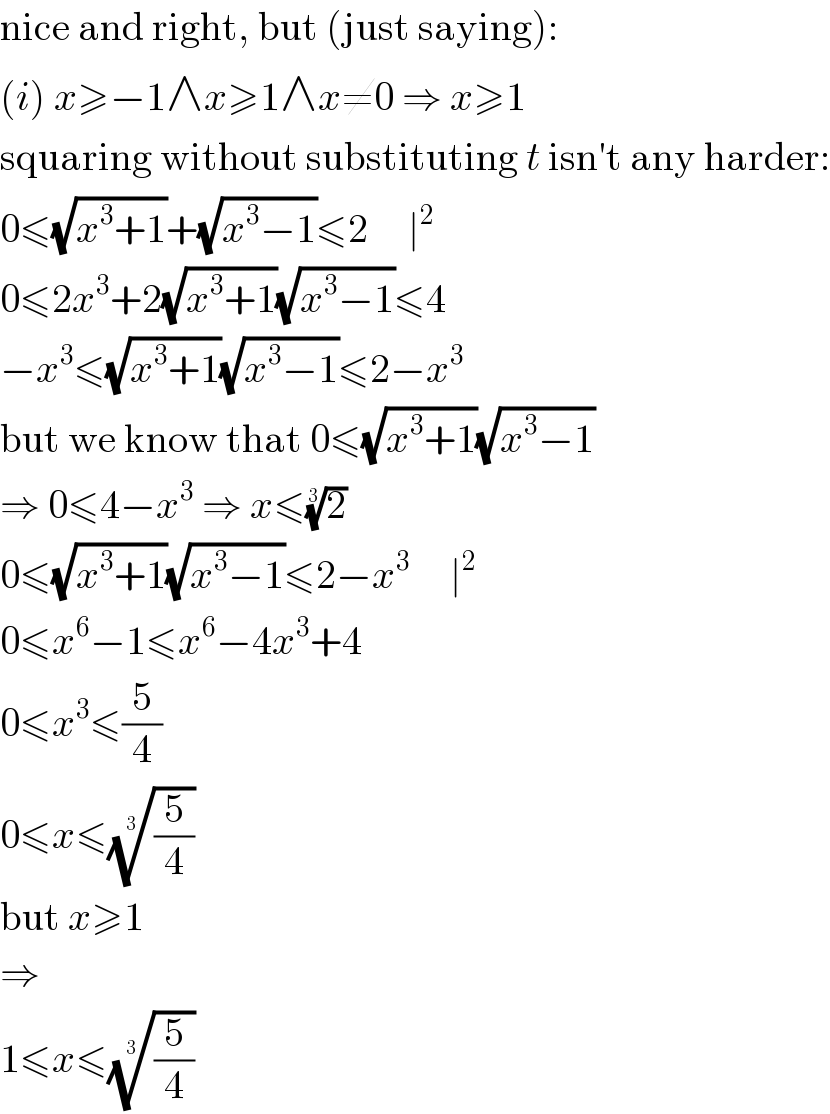
Commented by john santu last updated on 23/Jan/20

y e s d e p e n d i n g o n t h e d i r e c t i o n

o f o u r v i e w s i r