Menu Close

x-1-y-2-y-1-z-2-z-1-x-2-x-y-z-

Tinku Tara 0 Comments
Pin




Question Number 149588 by mathdanisur last updated on 06/Aug/21
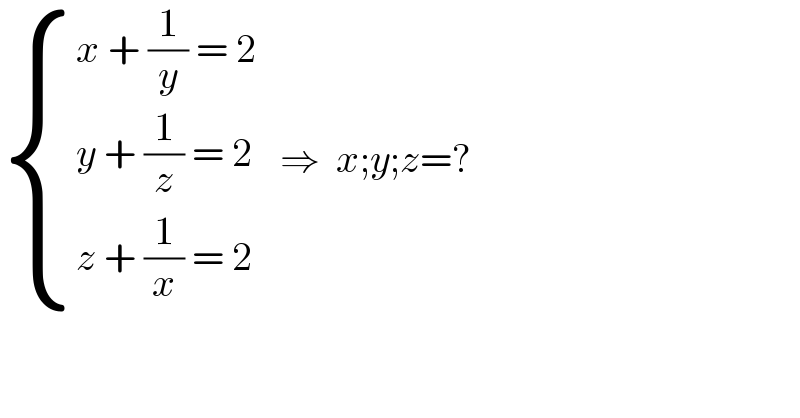
{ ((x + (1/y) = 2)),((y + (1/z) = 2)),((z + (1/x) = 2)) :} ⇒ x;y;z=?
$$\begin{cases}{{x}\:+\:\frac{\mathrm{1}}{{y}}\:=\:\mathrm{2}}\\{{y}\:+\:\frac{\mathrm{1}}{{z}}\:=\:\mathrm{2}}\\{{z}\:+\:\frac{\mathrm{1}}{{x}}\:=\:\mathrm{2}}\end{cases}\:\:\:\Rightarrow\:\:{x};{y};{z}=? \\ $$
Commented by Ar Brandon last updated on 06/Aug/21
1;1;1
$$\mathrm{1};\mathrm{1};\mathrm{1} \\ $$
Commented by mathdanisur last updated on 06/Aug/21
Thankyou Ser, solution
$$\mathrm{Thankyou}\:\boldsymbol{\mathrm{S}}\mathrm{er},\:\mathrm{solution} \\ $$
Answered by iloveisrael last updated on 06/Aug/21
If { ((x+(1/y)=2)),((y+(1/z)=2)),((z+(1/x)=2 )) :} then find the value of (x,y,z). let { ((y=mx)),((z=nx)) :} ⇒ { ((x+(1/(mx))=2⇒mx^2 −2mx+1=0)),((nx+(1/x)=2⇒nx^2 −2x+1=0)) :} it follows that m=n so we get { ((y=mx)),((z=mx)) :} consider eq(3)⇒mx+(1/(mx))=2 ⇒m^2 x^2 −2mx+1=0 ⇒(mx−1)^2 =0 ⇒mx=1 ⇒m=(1/x) then { ((y=mx=1)),((z=mx=1)) :} and x+1 = 2 ⇒x = 1. solution (x,y,z)=(1,1,1)
$$\:\mathrm{If}\:\begin{cases}{\mathrm{x}+\frac{\mathrm{1}}{\mathrm{y}}=\mathrm{2}}\\{\mathrm{y}+\frac{\mathrm{1}}{\mathrm{z}}=\mathrm{2}}\\{\mathrm{z}+\frac{\mathrm{1}}{\mathrm{x}}=\mathrm{2}\:}\end{cases}\:\mathrm{then}\:\mathrm{find}\:\mathrm{the}\:\mathrm{value} \\ $$$$\mathrm{of}\:\left(\mathrm{x},\mathrm{y},\mathrm{z}\right).\: \\ $$$$\mathrm{let}\:\begin{cases}{\mathrm{y}=\mathrm{mx}}\\{\mathrm{z}=\mathrm{nx}}\end{cases}\:\Rightarrow\begin{cases}{\mathrm{x}+\frac{\mathrm{1}}{\mathrm{mx}}=\mathrm{2}\Rightarrow\mathrm{mx}^{\mathrm{2}} −\mathrm{2mx}+\mathrm{1}=\mathrm{0}}\\{\mathrm{nx}+\frac{\mathrm{1}}{\mathrm{x}}=\mathrm{2}\Rightarrow\mathrm{nx}^{\mathrm{2}} −\mathrm{2x}+\mathrm{1}=\mathrm{0}}\end{cases} \\ $$$$\mathrm{it}\:\mathrm{follows}\:\mathrm{that}\:\mathrm{m}=\mathrm{n}\:\mathrm{so}\:\mathrm{we}\:\mathrm{get} \\ $$$$\begin{cases}{\mathrm{y}=\mathrm{mx}}\\{\mathrm{z}=\mathrm{mx}}\end{cases}\:\mathrm{consider}\:\mathrm{eq}\left(\mathrm{3}\right)\Rightarrow\mathrm{mx}+\frac{\mathrm{1}}{\mathrm{mx}}=\mathrm{2} \\ $$$$\Rightarrow\mathrm{m}^{\mathrm{2}} \mathrm{x}^{\mathrm{2}} −\mathrm{2mx}+\mathrm{1}=\mathrm{0} \\ $$$$\Rightarrow\left(\mathrm{mx}−\mathrm{1}\right)^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow\mathrm{mx}=\mathrm{1}\:\Rightarrow\mathrm{m}=\frac{\mathrm{1}}{\mathrm{x}}\:\mathrm{then}\:\begin{cases}{\mathrm{y}=\mathrm{mx}=\mathrm{1}}\\{\mathrm{z}=\mathrm{mx}=\mathrm{1}}\end{cases} \\ $$$$\mathrm{and}\:\mathrm{x}+\mathrm{1}\:=\:\mathrm{2}\:\Rightarrow\mathrm{x}\:=\:\mathrm{1}.\: \\ $$$$\mathrm{solution}\:\left(\mathrm{x},\mathrm{y},\mathrm{z}\right)=\left(\mathrm{1},\mathrm{1},\mathrm{1}\right) \\ $$
Commented by Rasheed.Sindhi last updated on 07/Aug/21
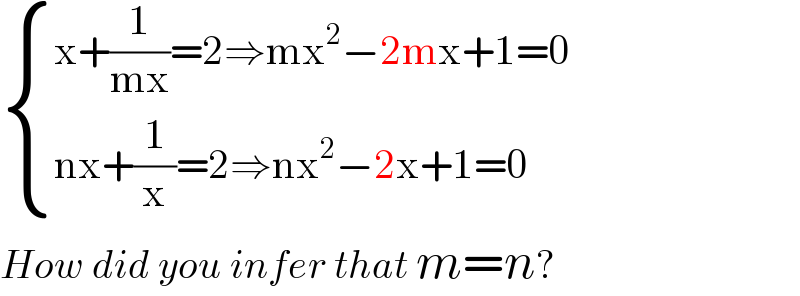
{ ((x+(1/(mx))=2⇒mx^2 −2mx+1=0)),((nx+(1/x)=2⇒nx^2 −2x+1=0)) :} How did you infer that m=n?
$$\begin{cases}{\mathrm{x}+\frac{\mathrm{1}}{\mathrm{mx}}=\mathrm{2}\Rightarrow\mathrm{mx}^{\mathrm{2}} −\mathrm{2mx}+\mathrm{1}=\mathrm{0}}\\{\mathrm{nx}+\frac{\mathrm{1}}{\mathrm{x}}=\mathrm{2}\Rightarrow\mathrm{nx}^{\mathrm{2}} −\mathrm{2x}+\mathrm{1}=\mathrm{0}}\end{cases} \\ $$$${How}\:{did}\:{you}\:{infer}\:{that}\:{m}={n}? \\ $$
Commented by Rasheed.Sindhi last updated on 07/Aug/21
Thank you!
$$\mathcal{T}{hank}\:{you}! \\ $$
Commented by iloveisrael last updated on 07/Aug/21
(m−n)x^2 +(2−2m)x=0 { ((m−n=0⇒m=n)),((2−2m=0⇒m=1)) :}
$$\left(\mathrm{m}−\mathrm{n}\right)\mathrm{x}^{\mathrm{2}} +\left(\mathrm{2}−\mathrm{2m}\right)\mathrm{x}=\mathrm{0} \\ $$$$\:\begin{cases}{\mathrm{m}−\mathrm{n}=\mathrm{0}\Rightarrow\mathrm{m}=\mathrm{n}}\\{\mathrm{2}−\mathrm{2m}=\mathrm{0}\Rightarrow\mathrm{m}=\mathrm{1}}\end{cases} \\ $$
Commented by mathdanisur last updated on 06/Aug/21
Ser, thank you
$$\boldsymbol{\mathrm{S}}\mathrm{er},\:\mathrm{thank}\:\mathrm{you} \\ $$
Answered by Rasheed.Sindhi last updated on 06/Aug/21
{ (((i):x + (1/y) = 2)),(((ii):y + (1/z) = 2)),(((iii):z + (1/x) = 2)) :} ⇒ x;y;z=? (iii)⇒x=(1/(2−z)) (ii)⇒y=2−(1/z)=((2z−1)/z) (i)⇒(1/(2−z))+(z/(2z−1))=2 2z−1+2z−z^2 =2(2−z)(2z−1) −z^2 +4z−1=2(4z−2−2z^2 +z) −z^2 +4z−1=−4z^2 +10z−4 3z^2 −6z+3=0 z^2 −2z+1=0 (z−1)^2 =0 z=1 (iii)⇒1+(1/x)=2⇒(1/x)=1⇒x=1 (ii)⇒y+(1/1)=2⇒y=1 (x,y,z)=(1,1,1)
$$\begin{cases}{\left({i}\right):{x}\:+\:\frac{\mathrm{1}}{{y}}\:=\:\mathrm{2}}\\{\left({ii}\right):{y}\:+\:\frac{\mathrm{1}}{{z}}\:=\:\mathrm{2}}\\{\left({iii}\right):{z}\:+\:\frac{\mathrm{1}}{{x}}\:=\:\mathrm{2}}\end{cases}\:\:\:\Rightarrow\:\:{x};{y};{z}=? \\ $$$$\left({iii}\right)\Rightarrow{x}=\frac{\mathrm{1}}{\mathrm{2}−{z}} \\ $$$$\left({ii}\right)\Rightarrow{y}=\mathrm{2}−\frac{\mathrm{1}}{{z}}=\frac{\mathrm{2}{z}−\mathrm{1}}{{z}} \\ $$$$\left({i}\right)\Rightarrow\frac{\mathrm{1}}{\mathrm{2}−{z}}+\frac{{z}}{\mathrm{2}{z}−\mathrm{1}}=\mathrm{2} \\ $$$$\mathrm{2}{z}−\mathrm{1}+\mathrm{2}{z}−{z}^{\mathrm{2}} =\mathrm{2}\left(\mathrm{2}−{z}\right)\left(\mathrm{2}{z}−\mathrm{1}\right) \\ $$$$−{z}^{\mathrm{2}} +\mathrm{4}{z}−\mathrm{1}=\mathrm{2}\left(\mathrm{4}{z}−\mathrm{2}−\mathrm{2}{z}^{\mathrm{2}} +{z}\right) \\ $$$$−{z}^{\mathrm{2}} +\mathrm{4}{z}−\mathrm{1}=−\mathrm{4}{z}^{\mathrm{2}} +\mathrm{10}{z}−\mathrm{4} \\ $$$$\:\:\mathrm{3}{z}^{\mathrm{2}} −\mathrm{6}{z}+\mathrm{3}=\mathrm{0} \\ $$$$\:\:{z}^{\mathrm{2}} −\mathrm{2}{z}+\mathrm{1}=\mathrm{0} \\ $$$$\:\:\:\left({z}−\mathrm{1}\right)^{\mathrm{2}} =\mathrm{0} \\ $$$$\:\:\:\:{z}=\mathrm{1} \\ $$$$\:\:\:\:\left({iii}\right)\Rightarrow\mathrm{1}+\frac{\mathrm{1}}{{x}}=\mathrm{2}\Rightarrow\frac{\mathrm{1}}{{x}}=\mathrm{1}\Rightarrow{x}=\mathrm{1} \\ $$$$\left({ii}\right)\Rightarrow{y}+\frac{\mathrm{1}}{\mathrm{1}}=\mathrm{2}\Rightarrow{y}=\mathrm{1} \\ $$$$\left({x},{y},{z}\right)=\left(\mathrm{1},\mathrm{1},\mathrm{1}\right) \\ $$
Commented by mathdanisur last updated on 07/Aug/21
Thank you Ser
$$\mathrm{Thank}\:\mathrm{you}\:\boldsymbol{\mathrm{S}}\mathrm{er} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *