Question Number 79398 by ubaydulla last updated on 24/Jan/20

$$\frac{{x}^{\mathrm{2}} −\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{1}}{dx} \\ $$
Commented by john santu last updated on 24/Jan/20
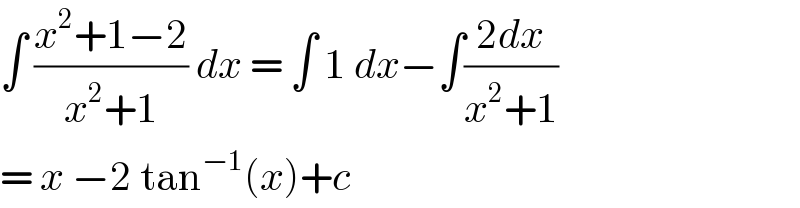
$$\int\:\frac{{x}^{\mathrm{2}} +\mathrm{1}−\mathrm{2}}{{x}^{\mathrm{2}} +\mathrm{1}}\:{dx}\:=\:\int\:\mathrm{1}\:{dx}−\int\frac{\mathrm{2}{dx}}{{x}^{\mathrm{2}} +\mathrm{1}} \\ $$$$=\:{x}\:−\mathrm{2}\:\mathrm{tan}^{−\mathrm{1}} \left({x}\right)+{c} \\ $$
