Menu Close

x-2-1-x-5-dx-

Tinku Tara 0 Comments
Pin




Question Number 87503 by M±th+et£s last updated on 04/Apr/20
∫(x^2 /(1+x^5 ))dx
$$\int\frac{{x}^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{5}} }{dx} \\ $$
Commented by MJS last updated on 04/Apr/20
x^5 +1=(x+1)(x^2 −((1−(√5))/2)x+1)(x^2 −((1+(√5))/2)+1) can you do it now?
$${x}^{\mathrm{5}} +\mathrm{1}=\left({x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} −\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} −\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}+\mathrm{1}\right) \\ $$$$\mathrm{can}\:\mathrm{you}\:\mathrm{do}\:\mathrm{it}\:\mathrm{now}? \\ $$
Commented by M±th+et£s last updated on 06/Apr/20
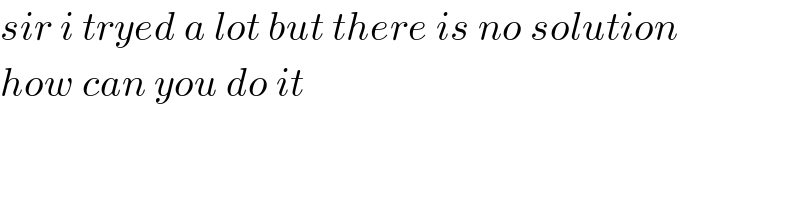
sir i tryed a lot but there is no solution how can you do it
$${sir}\:{i}\:{tryed}\:{a}\:{lot}\:{but}\:{there}\:{is}\:{no}\:{solution} \\ $$$${how}\:{can}\:{you}\:{do}\:{it} \\ $$
Commented by MJS last updated on 06/Apr/20
I will show later
$$\mathrm{I}\:\mathrm{will}\:\mathrm{show}\:\mathrm{later} \\ $$
Commented by M±th+et£s last updated on 06/Apr/20
thank you sir
$${thank}\:{you}\:{sir} \\ $$
Answered by MJS last updated on 06/Apr/20
x^5 +1=0 x_1 =−1 x_(2, 3) =e^(±i(π/5)) x_(4, 5) =e^(±i((3π)/5)) x^5 +1=(x−x_1 )((x−x_2 )(x−x_3 ))((x−x_4 )(x−x_5 ))= =(x+1)(x^2 −((1+(√5))/2)x+1)(x^2 −((1−(√5))/2)x+1) ⇒ (x^2 /(x^5 +1))=(α/(x+1))+((βx+γ)/(x^2 −((1+(√5))/2)x+1))+((δx+ε)/(x^2 −((1−(√5))/2)x+1)) ⇒ α=(1/5) β=γ=−(1/(10))+((√5)/(10)) δ=ε=−(1/(10))−((√5)/(10)) ⇒ ∫(x^2 /(x^5 +1))dx= =(1/5)∫(dx/(x+1))−((1−(√5))/(10))∫((x+1)/(x^2 −((1+(√5))/2)x+1))dx−((1+(√5))/(10))∫((x+1)/(x^2 −((1−(√5))/2)x+1))dx now use formulas
$${x}^{\mathrm{5}} +\mathrm{1}=\mathrm{0} \\ $$$${x}_{\mathrm{1}} =−\mathrm{1} \\ $$$${x}_{\mathrm{2},\:\mathrm{3}} =\mathrm{e}^{\pm\mathrm{i}\frac{\pi}{\mathrm{5}}} \\ $$$${x}_{\mathrm{4},\:\mathrm{5}} =\mathrm{e}^{\pm\mathrm{i}\frac{\mathrm{3}\pi}{\mathrm{5}}} \\ $$$${x}^{\mathrm{5}} +\mathrm{1}=\left({x}−{x}_{\mathrm{1}} \right)\left(\left({x}−{x}_{\mathrm{2}} \right)\left({x}−{x}_{\mathrm{3}} \right)\right)\left(\left({x}−{x}_{\mathrm{4}} \right)\left({x}−{x}_{\mathrm{5}} \right)\right)= \\ $$$$=\left({x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} −\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} −\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right) \\ $$$$\Rightarrow \\ $$$$\frac{{x}^{\mathrm{2}} }{{x}^{\mathrm{5}} +\mathrm{1}}=\frac{\alpha}{{x}+\mathrm{1}}+\frac{\beta{x}+\gamma}{{x}^{\mathrm{2}} −\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}}+\frac{\delta{x}+\epsilon}{{x}^{\mathrm{2}} −\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}} \\ $$$$\Rightarrow \\ $$$$\alpha=\frac{\mathrm{1}}{\mathrm{5}} \\ $$$$\beta=\gamma=−\frac{\mathrm{1}}{\mathrm{10}}+\frac{\sqrt{\mathrm{5}}}{\mathrm{10}} \\ $$$$\delta=\epsilon=−\frac{\mathrm{1}}{\mathrm{10}}−\frac{\sqrt{\mathrm{5}}}{\mathrm{10}} \\ $$$$\Rightarrow \\ $$$$\int\frac{{x}^{\mathrm{2}} }{{x}^{\mathrm{5}} +\mathrm{1}}{dx}= \\ $$$$=\frac{\mathrm{1}}{\mathrm{5}}\int\frac{{dx}}{{x}+\mathrm{1}}−\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{10}}\int\frac{{x}+\mathrm{1}}{{x}^{\mathrm{2}} −\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}}{dx}−\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{10}}\int\frac{{x}+\mathrm{1}}{{x}^{\mathrm{2}} −\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}}{dx} \\ $$$$\mathrm{now}\:\mathrm{use}\:\mathrm{formulas} \\ $$
Commented by M±th+et£s last updated on 06/Apr/20
god bless you sir
$${god}\:{bless}\:{you}\:{sir} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *