Question Number 37258 by rahul 19 last updated on 11/Jun/18

$$\int\:\frac{{x}^{\mathrm{3}} +\mathrm{1}}{\:\sqrt{{x}^{\mathrm{2}} +{x}}}\:{dx}\:=\:? \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 11/Jun/18
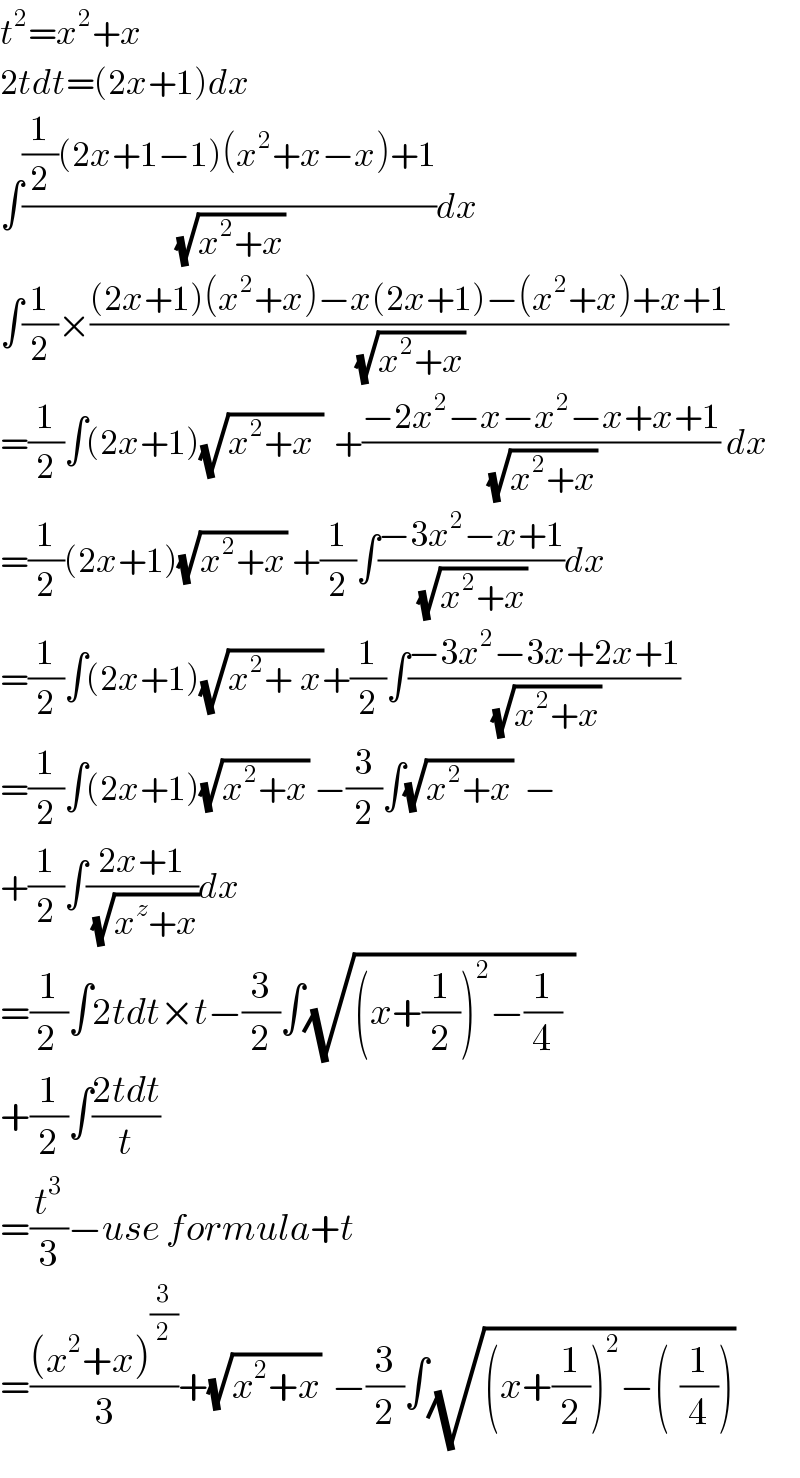
$${t}^{\mathrm{2}} ={x}^{\mathrm{2}} +{x} \\ $$$$\mathrm{2}{tdt}=\left(\mathrm{2}{x}+\mathrm{1}\right){dx} \\ $$$$\int\frac{\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{2}{x}+\mathrm{1}−\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}−{x}\right)+\mathrm{1}}{\:\sqrt{{x}^{\mathrm{2}} +{x}}}{dx} \\ $$$$\int\frac{\mathrm{1}}{\mathrm{2}}×\frac{\left(\mathrm{2}{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}\right)−{x}\left(\mathrm{2}{x}+\mathrm{1}\right)−\left({x}^{\mathrm{2}} +{x}\right)+{x}+\mathrm{1}}{\:\sqrt{{x}^{\mathrm{2}} +{x}}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\left(\mathrm{2}{x}+\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} +{x}_{} }\:\:+\frac{−\mathrm{2}{x}^{\mathrm{2}} −{x}−{x}^{\mathrm{2}} −{x}+{x}+\mathrm{1}}{\:\sqrt{{x}^{\mathrm{2}} +{x}}}\:{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{2}{x}+\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} +{x}}\:+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{−\mathrm{3}{x}^{\mathrm{2}} −{x}+\mathrm{1}}{\:\sqrt{{x}^{\mathrm{2}} +{x}}}{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\left(\mathrm{2}{x}+\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} +_{} {x}}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{−\mathrm{3}{x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}{x}+\mathrm{1}}{\:\sqrt{{x}^{\mathrm{2}} +{x}}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int\left(\mathrm{2}{x}+\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} +{x}}\:−\frac{\mathrm{3}}{\mathrm{2}}\int\sqrt{{x}^{\mathrm{2}} +{x}}\:\:− \\ $$$$+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{2}{x}+\mathrm{1}}{\:\sqrt{{x}^{{z}} +{x}}}{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}\:}\int\mathrm{2}{tdt}×{t}−\frac{\mathrm{3}}{\mathrm{2}}\int\sqrt{\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{4}}\:\:} \\ $$$$+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{2}{tdt}}{{t}} \\ $$$$=\frac{{t}^{\mathrm{3}} }{\mathrm{3}}−{use}\:{formula}+{t} \\ $$$$=\frac{\left({x}^{\mathrm{2}} +{x}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} }{\mathrm{3}}+\sqrt{{x}^{\mathrm{2}} +{x}}\:\:−\frac{\mathrm{3}}{\mathrm{2}}\int\sqrt{\left({x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} −\left(_{} \frac{\mathrm{1}}{\mathrm{4}}\right)} \\ $$
Commented by rahul 19 last updated on 13/Jun/18

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir}. \\ $$
