x-3-3x-12-0-solve-real-number-help-

Question Number 150752 by Jamshidbek last updated on 15/Aug/21

x^{3} + 3 x + 12 = 0 solve real number

help

Commented by liberty last updated on 15/Aug/21

x = root(3, - root(2, 37) - 6) - (1/root(3, - root(2, 37) - 6))

Commented by liberty last updated on 15/Aug/21

Answered by mr W last updated on 15/Aug/21

Δ = 1^{3} + 6^{2} = 37 > 0

x = \sqrt[3]{\sqrt{37} - 6} - \sqrt[3]{\sqrt{37} + 6}

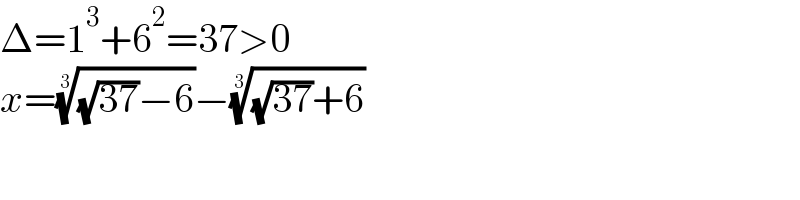
Commented by puissant last updated on 15/Aug/21

s i r i t i s t h e f o r m u l a o f T a r t a g l i a . . ?

Commented by mr W last updated on 15/Aug/21

{c a r d a n o}^{'} s

Commented by puissant last updated on 15/Aug/21

\Rightarrow x^{3} = - 3 x - 12

x = \sqrt[3]{- 6 + \sqrt{37}} - \sqrt[3]{6 + \sqrt{37}} s o r r y s i r . .

Commented by naka3546 last updated on 15/Aug/21

m a y b e :

x = \frac{\sqrt[3]{{(\sqrt{37} - 6)}^{2}} - 1}{\sqrt[3]{\sqrt{37} - 6}}

x = \sqrt[3]{\sqrt{37} - 6} - \sqrt[3]{\sqrt{37} + 6}

Commented by Tawa11 last updated on 15/Aug/21

Facebook Tweet Pin