Question Number 27419 by ayushrtet last updated on 06/Jan/18
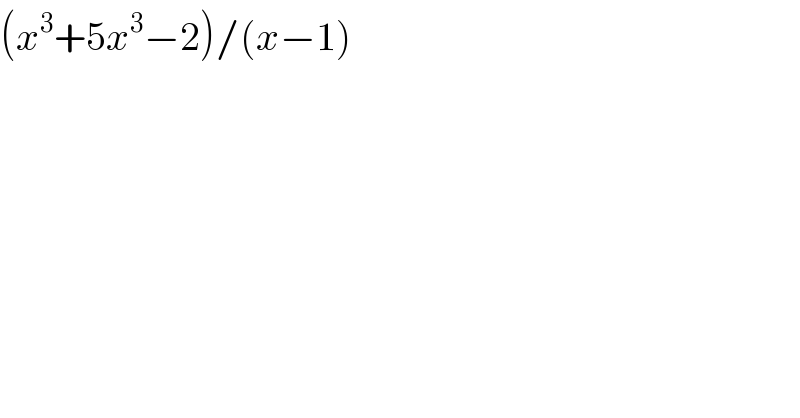
$$\left({x}^{\mathrm{3}} +\mathrm{5}{x}^{\mathrm{3}} −\mathrm{2}\right)/\left({x}−\mathrm{1}\right) \\ $$
Answered by Rasheed.Sindhi last updated on 06/Jan/18
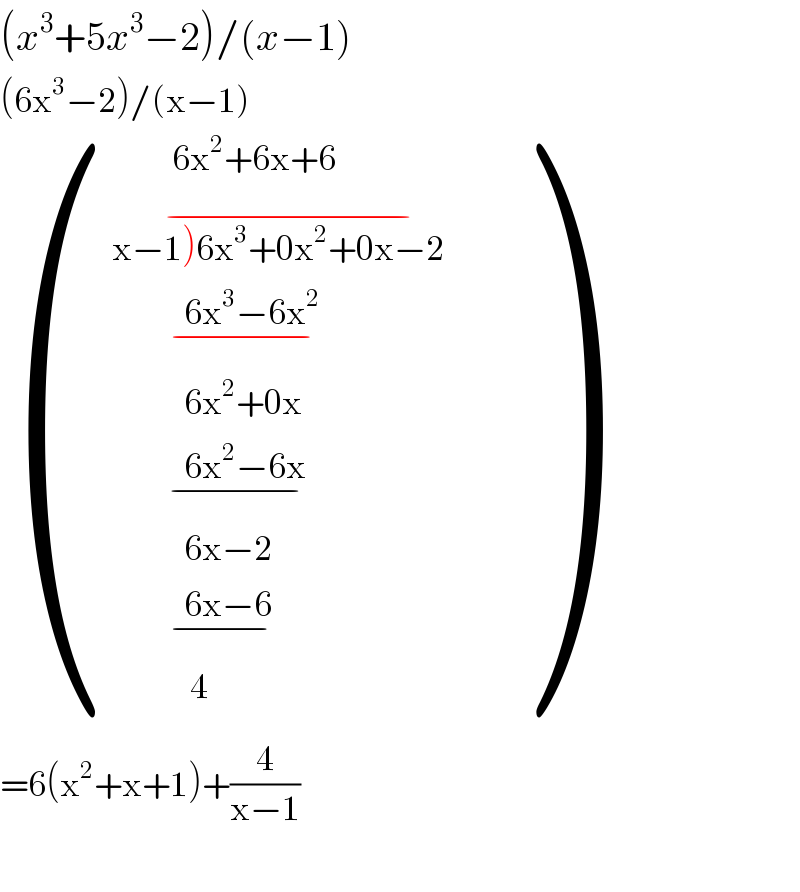
$$\left({x}^{\mathrm{3}} +\mathrm{5}{x}^{\mathrm{3}} −\mathrm{2}\right)/\left({x}−\mathrm{1}\right) \\ $$$$\left(\mathrm{6x}^{\mathrm{3}} −\mathrm{2}\right)/\left(\mathrm{x}−\mathrm{1}\right) \\ $$$$\begin{pmatrix}{\:\:\:\:\:\:\:\:\:\:\mathrm{6x}^{\mathrm{2}} +\mathrm{6x}+\mathrm{6}}\\{\left.\mathrm{x}\overline {−\mathrm{1}\right)\mathrm{6x}^{\mathrm{3}} +\mathrm{0x}^{\mathrm{2}} +\mathrm{0x}−\mathrm{2}\:\:\:\:}}\\{\:\:\:\:\:\:\:\underset{−} {\:\:\:\:\:\mathrm{6x}^{\mathrm{3}} −\mathrm{6x}^{\mathrm{2}} \:\:\:\:}}\\{\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{6x}^{\mathrm{2}} +\mathrm{0x}}\\{\:\:\:\:\:\:\:\underset{−} {\:\:\:\:\:\mathrm{6x}^{\mathrm{2}} −\mathrm{6x}\:\:\:\:}}\\{\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{6x}−\mathrm{2}}\\{\:\:\:\:\:\:\:\:\underset{−} {\:\:\:\:\mathrm{6x}−\mathrm{6}\:\:\:}}\\{\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{4}}\end{pmatrix} \\ $$$$=\mathrm{6}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)+\frac{\mathrm{4}}{\mathrm{x}−\mathrm{1}} \\ $$$$\:\:\:\:\:\:\:\:\: \\ $$
