x-3-ax-b-dx-

Question Number 123964 by mindispower last updated on 29/Nov/20

\int \sqrt{x^{3} + a x + b} d x

Commented by MJS_new last updated on 29/Nov/20

x_{1} = p

x_{2} = - \frac{p}{2} - \sqrt{q}

x_{3} = - \frac{p}{2} + \sqrt{q}

(x - x_{1}) (x - x_{2}) (x - x_{3}) = x^{3} + a x + b \Rightarrow

a = - \frac{3 p^{2}}{4} - q \land b = - \frac{p^{3}}{4} + p q

\Rightarrow we can try

\int \sqrt{x - p} \sqrt{x^{2} + p x + \frac{p^{2}}{4} - q} d x

but I found no path yet

Commented by mindispower last updated on 30/Nov/20

t h a n k y o u s i r m e t o o i h a v e n o t f o u n d s o m t h i n g

m y b e e t h e r e i s n o c l o s e] f o r m o r

s o m e s p e c i a l f u n c t i o n w e d o n t s e e y e t

h a v e a n i c e d a y s i r

Commented by MJS_new last updated on 30/Nov/20

Wolfram Alpha gives super complicated

solutions for \int \sqrt{x - p} \sqrt{x^{2} + p x + \frac{p^{2}}{4} - q} d x

and \int \sqrt{x - p} \sqrt{x + \frac{p}{2} - \sqrt{q}} \sqrt{x + \frac{p}{2} + \sqrt{q}} d x,

(for \int \sqrt{x^{3} + a x + b} d x {it}^{'} s also including the

You can't use 'macro parameter character #' in math mode

including elliptic functions E and F; but it

cannot solve \int \sqrt{x - a} \sqrt{x - b} \sqrt{x - c} at all .

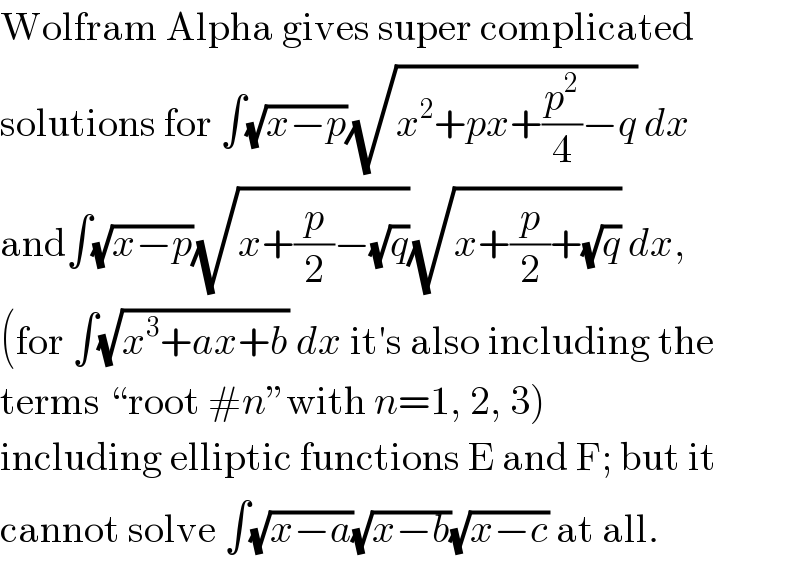
Commented by mindispower last updated on 30/Nov/20

t h a n k y o u s i r

i s e e

\int \sqrt{x - a} . \sqrt{x^{2} \underline{+} b}

c a n b e e s o l v e d b y

x = \sqrt{b} c h (t), w e h e n -

x = \sqrt{b} s h (t), w h e n + m a y b e e

t h a n x a g a i n s i r

x =