Menu Close

x-4-x-3-x-2-x-1-0-

Tinku Tara 0 Comments
Pin




Question Number 173839 by Khalmohmmad last updated on 19/Jul/22
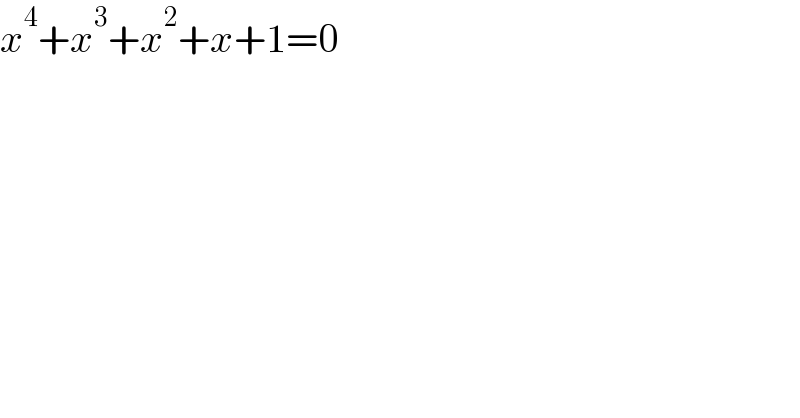
x^4 +x^3 +x^2 +x+1=0
x4+x3+x2+x+1=0
Commented by mr W last updated on 19/Jul/22
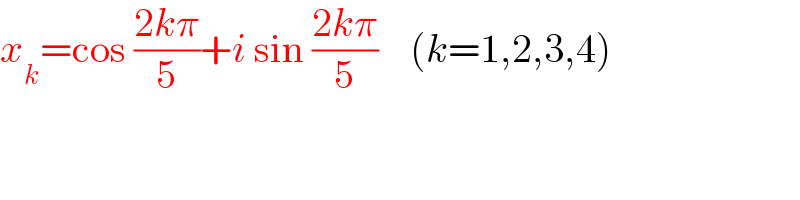
x_k =cos ((2kπ)/5)+i sin ((2kπ)/5) (k=1,2,3,4)
xk=cos2kπ5+isin2kπ5(k=1,2,3,4)
Answered by mahdipoor last updated on 19/Jul/22
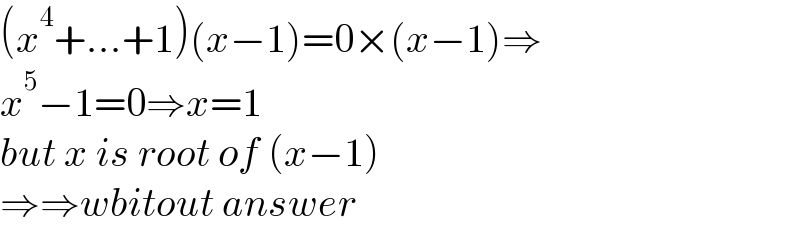
(x^4 +...+1)(x−1)=0×(x−1)⇒ x^5 −1=0⇒x=1 but x is root of (x−1) ⇒⇒wbitout answer
(x4+…+1)(x−1)=0×(x−1)⇒x5−1=0⇒x=1butxisrootof(x−1)⇒⇒wbitoutanswer
Answered by Rasheed.Sindhi last updated on 19/Jul/22
x^4 +x^3 +x^2 +x+1=0 (x−1)(x^4 +x^3 +x^2 +x+1)=0 x^5 −1=0 x^5 =1 x is fifth root of unity Let δ is one 5th root of unity. All the 5th roots are δ ,δ^2 ,δ^3 ,δ^4 , δ^5 =1 x=1 is root of x−1 and is not root of the given equation. ∴ δ ,δ^2 ,δ^3 ,δ^4 are the roots of the given equation.
x4+x3+x2+x+1=0(x−1)(x4+x3+x2+x+1)=0x5−1=0x5=1xisfifthrootofunityLetδisone5throotofunity.Allthe5throotsareδ,δ2,δ3,δ4,δ5=1x=1isrootofx−1andisnotrootofthegivenequation.∴δ,δ2,δ3,δ4aretherootsofthegivenequation.
Answered by BaliramKumar last updated on 20/Jul/22
x^4 +x^3 +x^2 +x+1=(x^2 +ax+1)(x^2 +bx+1)=0 x^4 +x^3 +x^2 +x+1=x^4 +(a+b)x^3 +(ab+2)x^2 +(a+b)x+1=0 a+b=1 ab+2=1 a = (((√5)+1)/2) b = −(((√5)−1)/2) x^4 +x^3 +x^2 +x+1=[x^2 +((((√5)+1)/2))x+1][x^2 −((((√5)−1)/2))x+1]=0 x^2 +((((√5)+1)/2))x + 1 = 0 & x^2 −((((√5)−1)/2))x + 1 = 0 .....................
x4+x3+x2+x+1=(x2+ax+1)(x2+bx+1)=0x4+x3+x2+x+1=x4+(a+b)x3+(ab+2)x2+(a+b)x+1=0a+b=1ab+2=1a=5+12b=−5−12x4+x3+x2+x+1=[x2+(5+12)x+1][x2−(5−12)x+1]=0x2+(5+12)x+1=0&x2−(5−12)x+1=0…………………

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *