x-5-4x-4-6x-3-8x-32-0-Find-at-least-one-root-

Question Number 55198 by ajfour last updated on 19/Feb/19

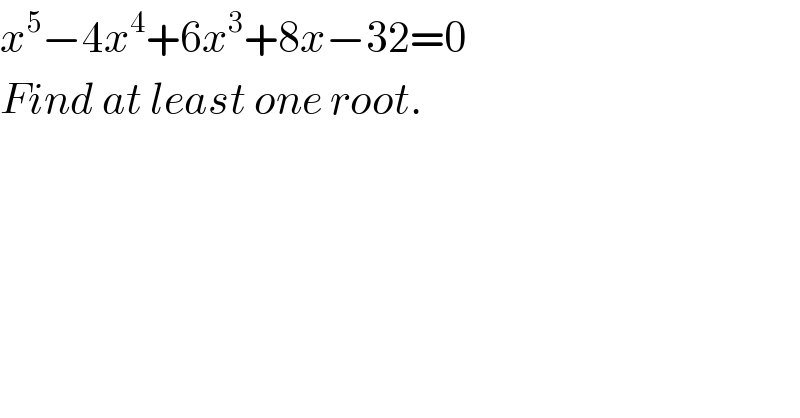
x^{5} - 4 x^{4} + 6 x^{3} + 8 x - 32 = 0

F i n d a t l e a s t o n e r o o t .

Commented by arvinddayama00@gmail.com last updated on 19/Feb/19

a l l v e l u e o f x = ?

Answered by rahul 19 last updated on 19/Feb/19

x = 2!

Commented by rahul 19 last updated on 19/Feb/19

simply by hit & trial . ��

Answered by tanmay.chaudhury50@gmail.com last updated on 19/Feb/19

x^{5} + 6 x^{3} + 8 x = 4 x^{4} + 32

r i g h t h a n d s i d e x^{e v e n p o w e r} s o r i g h t h a n d

s i d e i s a l w a y s + v e

n o w l e f t h a n d s i d e s h o u l d b e + v e

s o x c a n n o t b e l e s s t h a n z e r o

s o x ≮ 0

n o w

(x^{5} + 6 x^{3} + 8 x) - (4 x^{4} + 32) = 0

f (x) = (x^{5} + 6 x^{3} + 8 x) - (4 x^{4} + 32)

f (x) < 0 a t x = 0

f (x) < 0 a t x = 1

s o n o r o o t i n b e t w e e n (0, 1)

n o w c h e c k i n g b e t w e e n 1 a n d 2

f (2) = 32 + 48 + 16 - 64 - 32 = 0

s o x = 2 i s a r o o t . .

f (1) = 1 + 6 + 8 - 4 - 32 = - 21

n o w g r a d u a l l y w e i n c r e a s e t h e v a l u e o f x

s u c h a s 1.1, 1.2, \dots a n d o b s e r v e c h a n g e o f s i g n

f (x) = y = (x^{5} + 6 x^{3} + 8 x) - (4 x^{4} + 32)

\frac{d y}{d x} = 5 x^{4} + 18 x^{2} + 8 - 16 x^{3}

\frac{△ y}{△ x} \approx \frac{d y}{d x}

△ y = \frac{d y}{d x} \times △ x = (5 + 18 + 8 - 16) \times 0.1 = 1.5

s o f (1.1) = f (1) + △ y = - 21 + 1.5 = - 19.5

f (2.1) = f (2) + △ y = 0 + 1.5 = 1.5 > 0

f (3) = 3^{5} + 6 \times 3^{3} + 8 \times 3 - 4 \times 3^{4} - 32

= 3^{4} (3 - 4) + 6 \times 27 - 8

= - 81 + 162 - 8 > 0

s o n o r o o t b e t w e e n 2.1 a n d 3

\dots

f (4) = 4^{5} + 6 \times 4^{3} + 32 - 4 \times 4^{4} - 32 > 0

s o n o r o o t b e t w e e n 3 a n d 4

n o w \dots

f (x) = x^{5} + 6 x^{3} + 8 x - (4 x^{4} + 32)

f (x) = g (x) - h (x)

\frac{d f}{d x} = \frac{d g}{d x} - \frac{d h}{d x} = (5 x^{4} + 18 x^{2} + 8) - (16 x^{3})

= x^{3} (5 x - 16) + 18 x^{2} + 8 . .

n o w l o o k w h e n x > 3.2 \frac{d f}{d x} > 0

s o t h e r e w i l l b e n o s i g n c h a n g e w h e n x > 3.2

s o t h i s e q n h a s o n l y o n e r o o t t h a t i s

x = 2 \dots p l s p a y y o u r k i n d a t t e n t i o n \dots