Menu Close

x-6-1-x-4-1-dx-

Tinku Tara 0 Comments
Pin




Question Number 23849 by Sudipta Jana last updated on 08/Nov/17
∫((x^6 +1)/(x^4 +1))dx
$$\int\frac{{x}^{\mathrm{6}} +\mathrm{1}}{{x}^{\mathrm{4}} +\mathrm{1}}{dx} \\ $$
Answered by anunil1234 last updated on 08/Nov/17
∫(x^2 +(((−x^2 +1))/(x^4 +1)))dx (x^3 /3) −∫((x^2 −1)/(x^4 +1))dx+c1 (x^3 /3)−∫((1−(1/x^2 ))/(x^2 +(1/x^2 )))dx+c1 (x^3 /3)−∫(((1−(1/x^2 ))dx)/((x+(1/x))^2 −2))+c1 (x^3 /3)−(1/(2(√2)))log[(({x+(1/x)}−(√2))/({x+(1/x)}+(√2)))]+c
$$\int\left(\mathrm{x}^{\mathrm{2}} \:+\frac{\left(−\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}\right)}{\mathrm{x}^{\mathrm{4}} \:+\mathrm{1}}\right)\mathrm{dx} \\ $$$$\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}\:−\int\frac{\mathrm{x}^{\mathrm{2}} \:−\mathrm{1}}{\mathrm{x}^{\mathrm{4}} \:\:+\mathrm{1}}\mathrm{dx}+\mathrm{c1} \\ $$$$\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}−\int\frac{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }}{\mathrm{x}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }}\mathrm{dx}+\mathrm{c1} \\ $$$$\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}−\int\frac{\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }\right)\mathrm{dx}}{\left(\mathrm{x}+\frac{\mathrm{1}}{\mathrm{x}}\right)^{\mathrm{2}} −\mathrm{2}}+\mathrm{c1} \\ $$$$\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\mathrm{log}\left[\frac{\left\{\mathrm{x}+\frac{\mathrm{1}}{\mathrm{x}}\right\}−\sqrt{\mathrm{2}}}{\left\{\mathrm{x}+\frac{\mathrm{1}}{\mathrm{x}}\right\}+\sqrt{\mathrm{2}}}\right]+\mathrm{c} \\ $$
Answered by scottfeed last updated on 13/Nov/17
so using the quotient rule formula which says thus⋮ V(du/dx) + U(dv/dx) −−−−−−−−− V^2 v^2 =(x^4 +1)^2 so lets make u=x^6 +1 and v=x^4 +1 du/dx = 6x^5 dv/dx=4x^3 using the formula above we then input thier values (x^4 +1)(6x^5 ) + (x^6 +1)(4x^3 ) −−−−−−−−−−−−−−−−− (x^4 +1)^2 so by expanding we have 6x^9 +6x^5 + 4x^9 +4x^3 −−−−−−−−−−−−− (x^4 +1)^2 6x^9 +4x^9 +6x^5 +4x^3 −−−−−−−−−−−−− (x^4 +1)^2 so finally we have 10x^9 +6x^5 +4x^3 −−−−−−−−− (x^4 +1)^2
$${so}\:{using}\:{the}\:{quotient}\:{rule}\:{formula}\:{which}\:{says} \\ $$$${thus}\vdots\:\:\:\:\boldsymbol{\mathrm{V}}\frac{\boldsymbol{\mathrm{du}}}{\boldsymbol{\mathrm{dx}}}\:\:+\:\:\boldsymbol{\mathrm{U}}\frac{\boldsymbol{\mathrm{dv}}}{\boldsymbol{\mathrm{dx}}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−−−−−−−−− \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{V}}^{\mathrm{2}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{v}^{\mathrm{2}} =\left({x}^{\mathrm{4}} +\mathrm{1}\right)^{\mathrm{2}} \\ $$$${so}\:{lets}\:{make}\:\:\:\:\:\:{u}={x}^{\mathrm{6}} +\mathrm{1}\:\:\:\:\:\:\:\:\:\:\:{and}\:\:{v}={x}^{\mathrm{4}} +\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{du}/{dx}\:=\:\mathrm{6}{x}^{\mathrm{5}} \:\:\:\:\:\:\:{dv}/{dx}=\mathrm{4}{x}^{\mathrm{3}} \\ $$$${using}\:{the}\:{formula}\:{above}\:{we}\:{then}\:{input}\:{thier}\: \\ $$$${values} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\left({x}^{\mathrm{4}} +\mathrm{1}\right)\left(\mathrm{6}{x}^{\mathrm{5}} \right)\:\:+\:\:\left({x}^{\mathrm{6}} +\mathrm{1}\right)\left(\mathrm{4}{x}^{\mathrm{3}} \right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:−−−−−−−−−−−−−−−−− \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left({x}^{\mathrm{4}} +\mathrm{1}\right)^{\mathrm{2}} \\ $$$${so}\:{by}\:{expanding}\:{we}\:{have} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{6}{x}^{\mathrm{9}} +\mathrm{6}{x}^{\mathrm{5}} \:\:+\:\:\mathrm{4}{x}^{\mathrm{9}} +\mathrm{4}{x}^{\mathrm{3}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:−−−−−−−−−−−−− \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left({x}^{\mathrm{4}} +\mathrm{1}\right)^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{6}{x}^{\mathrm{9}} +\mathrm{4}{x}^{\mathrm{9}} +\mathrm{6}{x}^{\mathrm{5}} +\mathrm{4}{x}^{\mathrm{3}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−−−−−−−−−−−−− \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left({x}^{\mathrm{4}} +\mathrm{1}\right)^{\mathrm{2}} \\ $$$${so}\:{finally}\:{we}\:{have} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{10}{x}^{\mathrm{9}} +\mathrm{6}{x}^{\mathrm{5}} +\mathrm{4}{x}^{\mathrm{3}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−−−−−−−−− \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left({x}^{\mathrm{4}} +\mathrm{1}\right)^{\mathrm{2}} \\ $$
Commented by anunil1234 last updated on 13/Nov/17
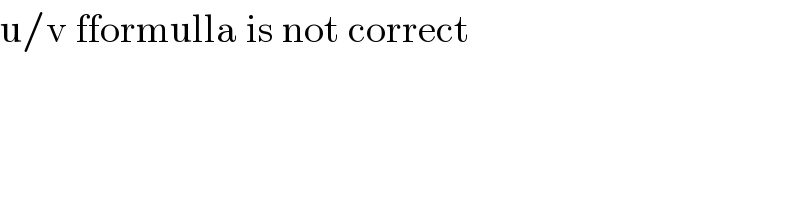
u/v fformulla is not correct
$$\mathrm{u}/\mathrm{v}\:\mathrm{fformulla}\:\mathrm{is}\:\mathrm{not}\:\mathrm{correct} \\ $$
Commented by scottfeed last updated on 13/Nov/17
ion get u
$${ion}\:{get}\:{u} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *