x-x-2-8-Find-x-

Question Number 56991 by Tawa1 last updated on 28/Mar/19

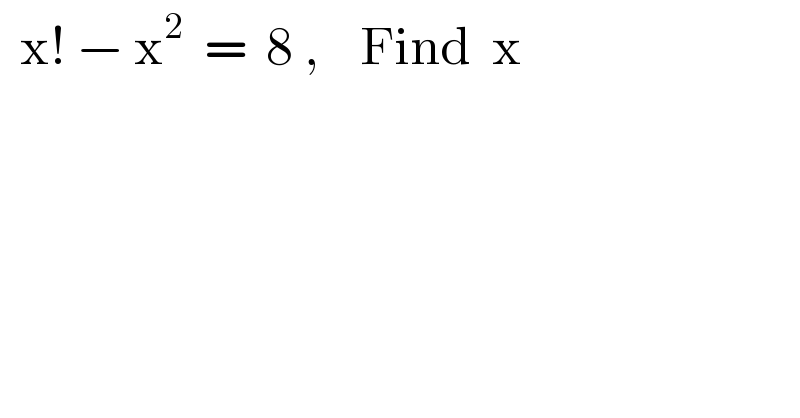
x! - x^{2} = 8, Find x

Commented by Joel578 last updated on 28/Mar/19

x = 4

Commented by Tawa1 last updated on 28/Mar/19

Any workings please

Commented by MJS last updated on 28/Mar/19

if x \in N we can only try

\Rightarrow x = 4

if x \in R we have to solve this :

Γ (x + 1) - x^{2} = 8

where Γ (x) = \underset{0}{\int^{\infty}} t^{x - 1} e^{- t} d t

and I {don}^{'} t think we are able to

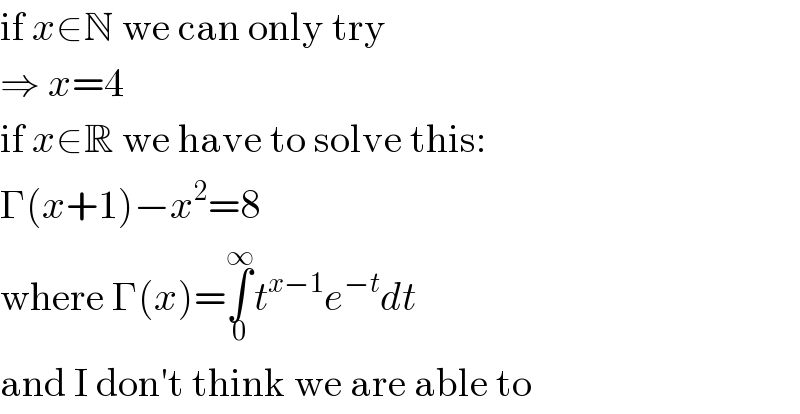
Commented by maxmathsup by imad last updated on 28/Mar/19

s i r t a w a 1 y o u m u s t f i r s t d e f i n e x!