x-x-3-x-faind-x-

Question Number 185599 by mathlove last updated on 24/Jan/23

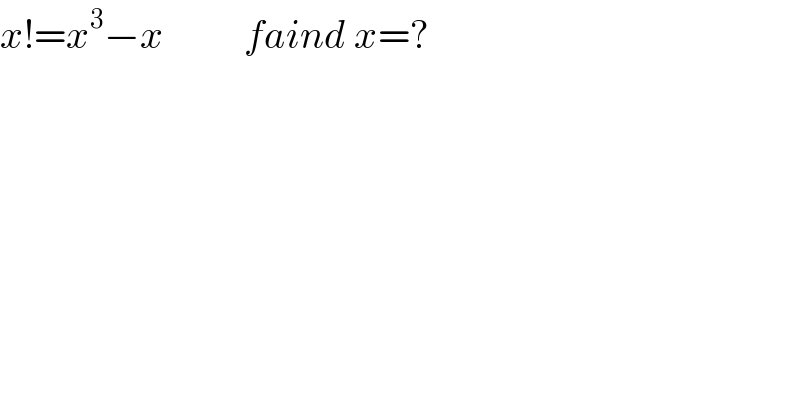
x! = x^{3} - x f a i n d x = ?

Commented by liuxinnan last updated on 24/Jan/23

5! = 120

5^{3} - 5 = 120

x < 5 x! < x^{3} - x

x > 5 x! > x^{3} - x

Commented by Rasheed.Sindhi last updated on 24/Jan/23

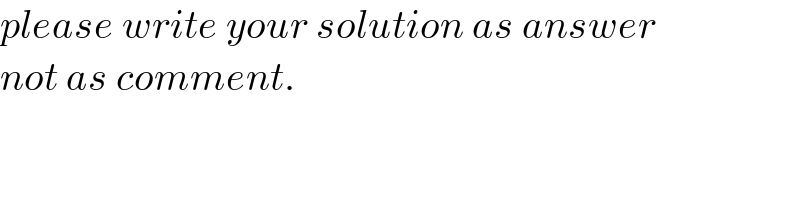
p l e a s e w r i t e y o u r s o l u t i o n a s a n s w e r

n o t a s c o m m e n t .

Commented by liuxinnan last updated on 24/Jan/23

n e x t m u s t

Answered by Frix last updated on 24/Jan/23

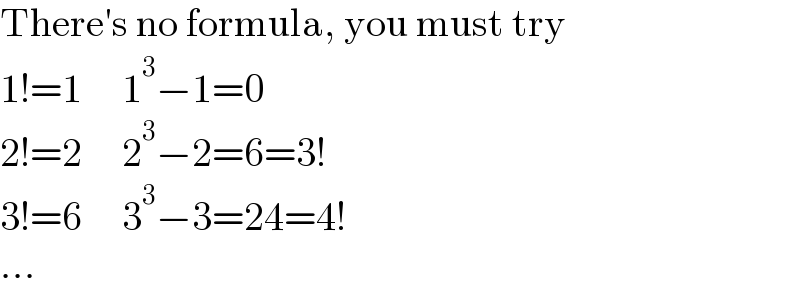
{There}^{'} s no formula, you must try

1! = 1 1^{3} - 1 = 0

2! = 2 2^{3} - 2 = 6 = 3!

3! = 6 3^{3} - 3 = 24 = 4!

\dots

Answered by ajfour last updated on 24/Jan/23

(x - 1)! + 1 = x^{2}

(x - 1)! + 1 = {(x - 1)}^{2} + 2 (x - 1) + 1

(x - 2)! = x - 2 + 3

(x - 3)! = 1 + \frac{3}{x - 2}

x > 3 a n d a l s o

(x - 3)! < 4

\Rightarrow x - 3 < 3

x < 6

s o l e t u s t r y x = 4 a n d x = 5

I g u e s s x = 5 s a t i s f i e s

5! = 120

5^{3} - 5 = 120

h e n c e x = 5

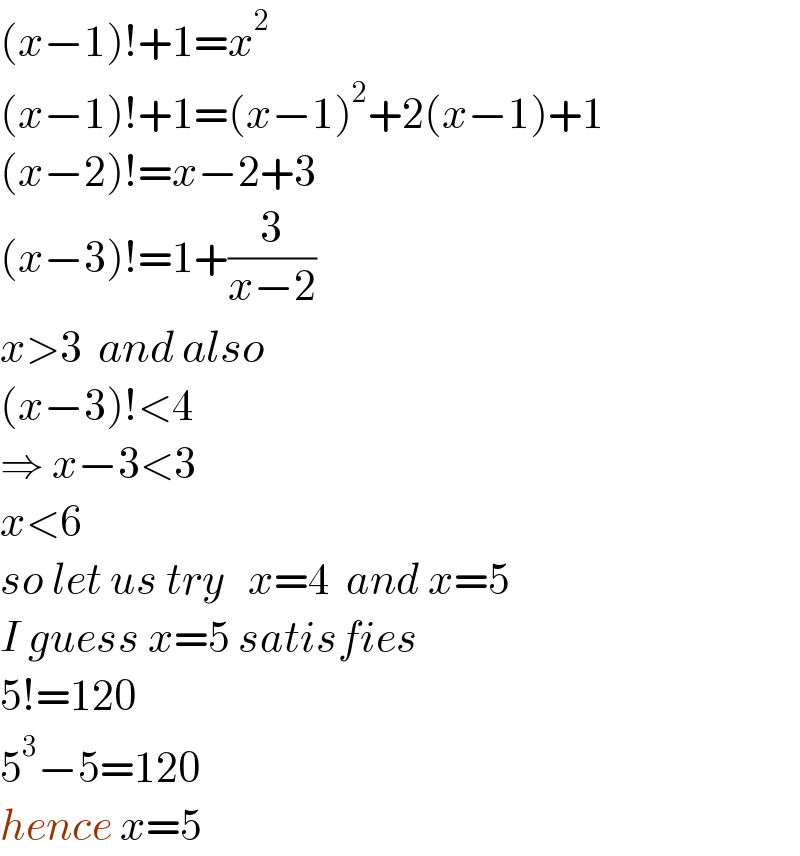
Commented by mathlove last updated on 24/Jan/23

t h a n k s

Answered by Rasheed.Sindhi last updated on 24/Jan/23

(x - 2)! (x - 1) x = x (x - 1) (x + 1)

(x - 2)! = x + 1

(x - 3)! = \frac{x + 1}{x - 2} - 1 + 1; x > 2

= \frac{3}{x - 2} + 1

x - 2 ∣ 3

x - 2 = 1, 3

x = 3, 5

3 f a i l s, 5 i s s u c c e s s f u l

x = 5^{✓} : (5 - 3)! = \frac{3}{5 - 2} + 1

2 = 2

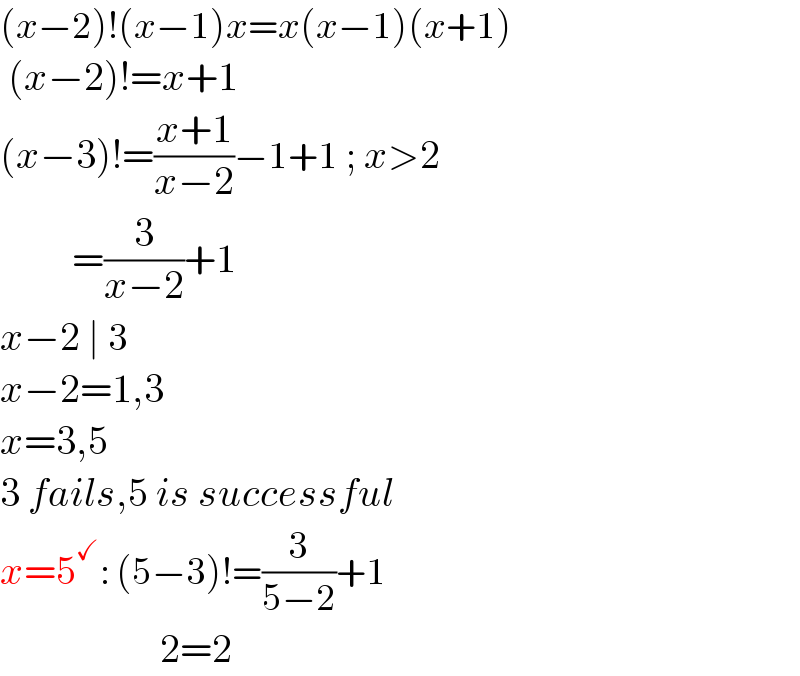
Answered by SEKRET last updated on 24/Jan/23

x \cdot (x - 1) \cdot (x - 2)! = x \cdot (x - 1) (x + 1)

(x - 2)! = x - 2 + 3

a! = a + 3 a > 0

1 = 4

2 = 5

6 = 6 a = 3

24 = 7 >>

x - 2 = 3

x = 5

Facebook Tweet Pin