x-x-64-find-x-

Question Number 65115 by hovea cw last updated on 25/Jul/19

x^{x} = 64

find x

Answered by mr W last updated on 25/Jul/19

x^{x} = 64

x = 64^{\frac{1}{x}}

x = e^{\frac{\ln 64}{x}}

1 = \frac{1}{x} e^{\frac{\ln 64}{x}}

\ln 64 = (\frac{\ln 64}{x}) e^{\frac{\ln 64}{x}}

\Rightarrow \frac{\ln 64}{x} = W (\ln 64)

\Rightarrow x = \frac{\ln 64}{W (\ln 64)} = \frac{\ln 64}{1.223517} = 3.399122

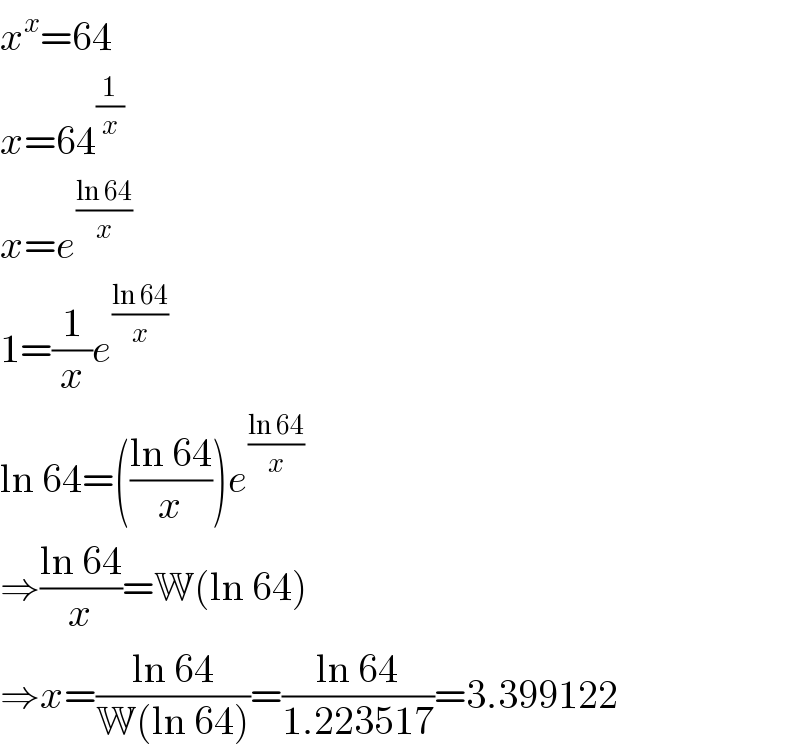
Commented by hovea cw last updated on 25/Jul/19

sir I don't know a lot of the Lambert omega function so am kinda stuck at the last few lines plz explain how the function comes about sir ��

Commented by mr W last updated on 25/Jul/19

L a m b e r t W f u n c t i o n i s t h e i n v e r s e

f u n c t i o n f r o m y = {x e}^{x} . i . e .

i f y = {x e}^{x} t h e n x = W (y) .

i n o u r e x a m p l e i h a v e f o r m e d t h e

o r i g i n a l e q u a t i o n i n t o

(\frac{\ln 64}{x}) e^{(\frac{\ln 64}{x})} = \ln 64

t h a t m e a n s

\frac{\ln 64}{x} = W (\ln 64)

\Rightarrow x = \frac{\ln 64}{W (\ln 64)}

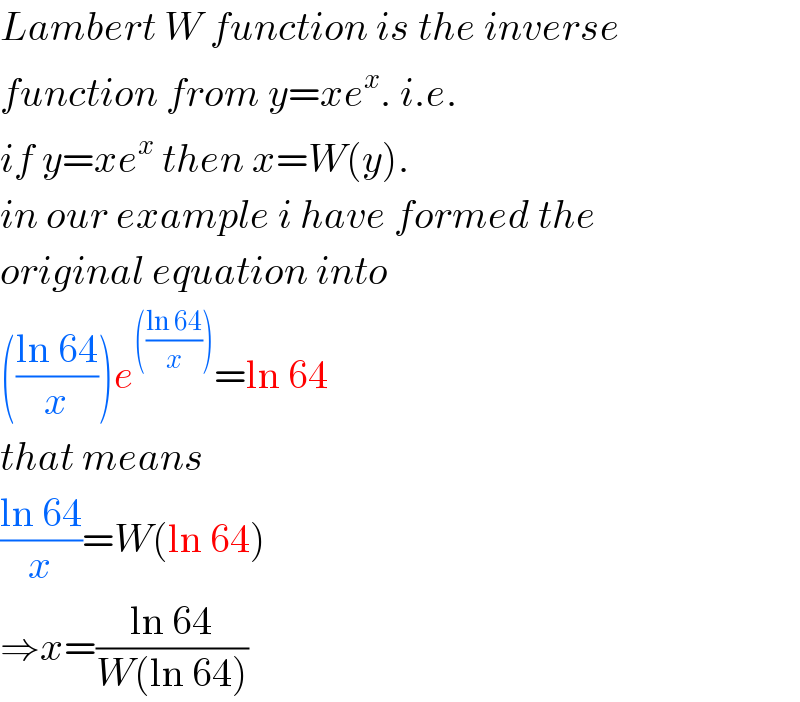
Commented by mr W last updated on 25/Jul/19

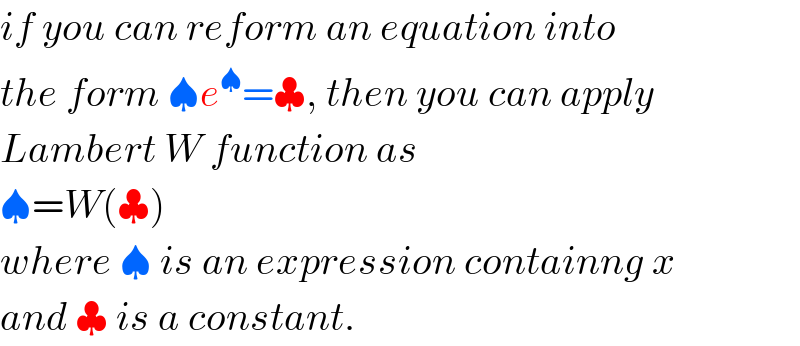
i f y o u c a n r e f o r m a n e q u a t i o n i n t o

t h e f o r m ♠ e^{♠} = ♣, t h e n y o u c a n a p p l y

L a m b e r t W f u n c t i o n a s

♠ = W (♣)

w h e r e ♠ i s a n e x p r e s s i o n c o n t a i n n g x

a n d ♣ i s a c o n s t a n t .

Commented by hovea cw last updated on 25/Jul/19

Thank u sir and how do we approximate the value of w or is it a constant number (*-*)

Commented by mr W last updated on 25/Jul/19

i n i n t e r n e t y o u m a y f i n d o n l i n e

c a l c u l a t o r f o r W f u n c t i o n v a l u e s .

b u t s i n c e W (\ln 64) i s t h e r o o t o f

{x e}^{x} = \ln 64

y o u m a y g e t t h e r o o t v i a g r a p h i c

m e t h o d . i u s e e . g . a n a p p n a m e d

G r a p h e r .

Facebook Tweet Pin