y-2-y-sec-3-x-

Question Number 26904 by sorour87 last updated on 31/Dec/17

y^{(2)} + y = \sec^{3} x

Commented by prakash jain last updated on 31/Dec/17

g (x) = \sec^{3} x

Homogenous solution

λ^{2} + 1 = 0 \Rightarrow λ = \pm i

y_{h} = c_{1} e^{i x} + c_{2} e^{- i x}

y_{h} = (c_{1} + c_{2}) \cos x + i (c_{1} - c_{2}) \cos x

y_{h} = C_{1} \cos x + C_{2} \cos x

y_{1} (x) = \cos x

y_{2} (x) = \sin (x)

W (y_{1}, y_{2}) = | \begin{matrix} \cos x & \sin x \\ - \sin x & \cos x \end{matrix} | = 1

u_{1} (x) = - \int \frac{y_{2} (x) g (x)}{1} d x

= - \int \sec^{3} x \sin x d x = - \int \sec^{2} x \tan x d x

= - \frac{1}{2} \sec^{2} x

u_{2} (x) = \int \frac{y_{1} (x) g (x)}{1} d x

= \int \sec^{3} x \cdot \cos x d x = \tan x

y_{p} (x) = u_{1} y_{1} + u_{2} y_{2} = - \frac{\sec x}{2} + \sin x \tan x

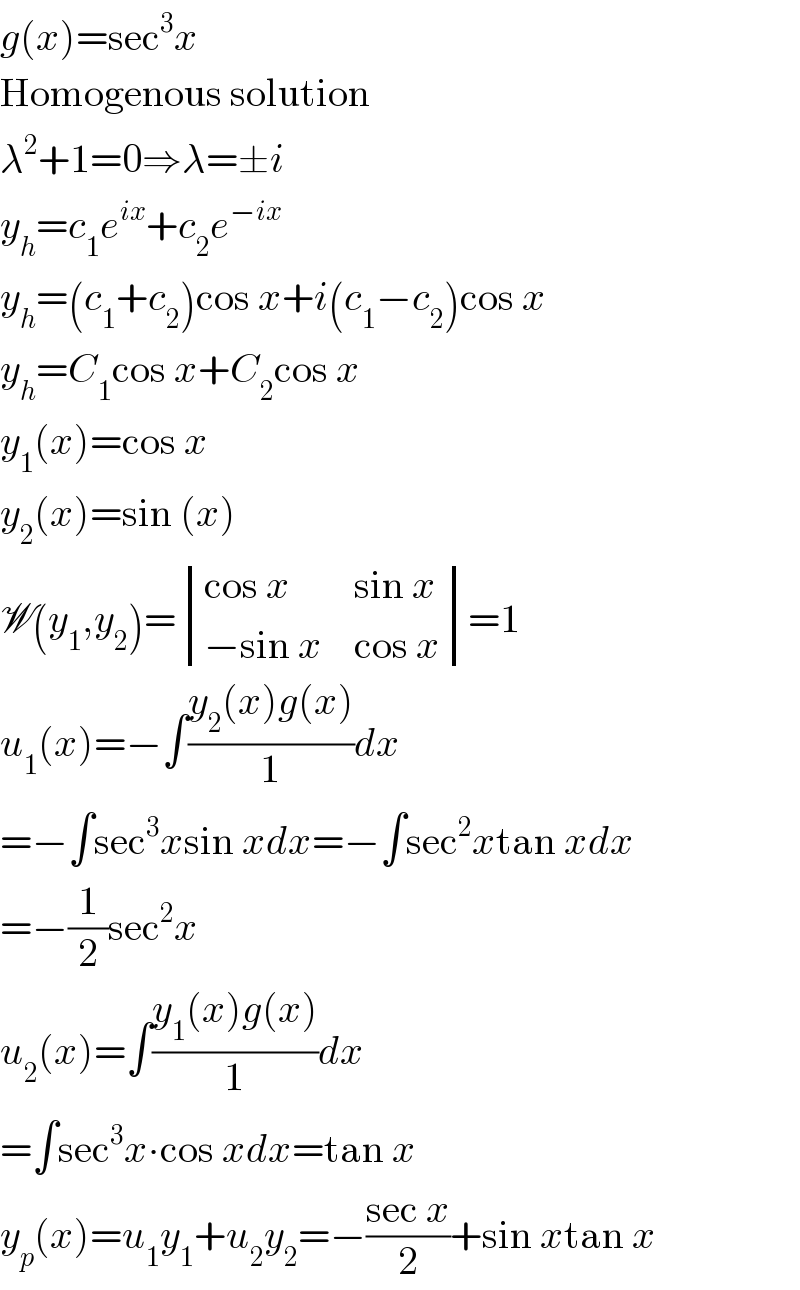
Answered by prakash jain last updated on 31/Dec/17

y = y_{h} + y_{p}

Commented by sorour87 last updated on 31/Dec/17

o k . t h a n k s

Commented by prakash jain last updated on 31/Dec/17

The following page desxribes the method of variation of parameters. http://tutorial.math.lamar.edu/Classes/DE/VariationofParameters.aspx