Question Number 113444 by Ar Brandon last updated on 13/Sep/20
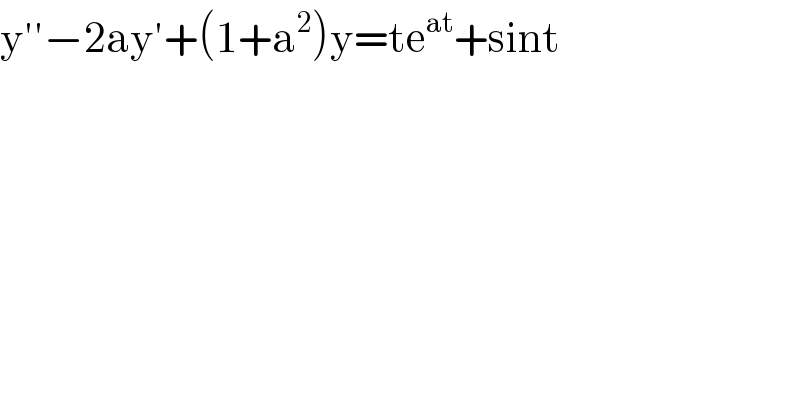
$$\mathrm{y}''−\mathrm{2ay}'+\left(\mathrm{1}+\mathrm{a}^{\mathrm{2}} \right)\mathrm{y}=\mathrm{te}^{\mathrm{at}} +\mathrm{sint} \\ $$
Answered by Ar Brandon last updated on 13/Sep/20
![y′′−2ay′+(1+a^2 )y=te^(at) +sint (h)→r^2 −2ar+1+a^2 =0⇒r=((2a±(√(4a^2 −4(1+a^2 ))))/2)=a±i y_h =e^(at) (Acost+Bsint) By varying parameters let; y_p =A(t)e^(at) cost+B(t)e^(at) sint ⇒y_p =au_1 +bu_2 { ((a′u_1 +b′u_2 =0),(...(1))),((a′u_1 ′+b′u_2 ′=te^(at) +sint),(...(2))) :} Finding a′ and b′ using Crammer′s method; W(u_1 ,u_2 )= determinant ((u_1 ,u_2 ),((u_1 ′),(u_2 ′)))= determinant (((e^(at) cost),(e^(at) sint)),((ae^(at) cost−e^(at) sint),(e^(at) cost+ae^(at) sint))) =e^(2at) [(cos^2 t+asintcost)−(asintcost−sin^2 t)]=e^(2at) w_1 = determinant ((0,(e^(at) sint)),((te^(at) +sint),(e^(at) cost+ae^(at) sint)))=−(te^(2at) sint+e^(at) sin^2 t) w_2 = determinant (((e^(at) cost),0),((ae^(at) cost−e^(at) sint),(te^(at) +sint)))=te^(2at) cost+e^(at) sintcost a′=(w_1 /W)⇒a=∫(w_1 /W)dt , b′=(w_2 /W)⇒b=∫(w_2 /W)dt ⇒A(t)=−∫((te^(2at) sint+e^(at) sin^2 t)/e^(2at) )dt, B(t)=∫((te^(2at) cost+e^(at) sintcost)/e^(2at) )dt A(t)=−∫(tsint+e^(−at) sin^2 t)dt ∫tsintdt=−tcost+∫costdt=sint−tcost ∫e^(−at) sin^2 tdt=−((sin^2 t)/a)∙e^(−at) +(2/a)∫sintcost∙e^(−at) dt =−((sin^2 t)/a)∙e^(−at) +(1/a)∫sin(2t)e^(−at) dt =−((sin^2 t)/a)∙e^(−at) +(1/a){−((sin(2t))/a)e^(−at) +(2/a)∫cos(2t)e^(−at) dt} =−((sin^2 t)/a)∙e^(−at) −((sin(2t))/a^2 )e^(−at) +(2/a^2 )[−((cos(2t))/a)e^(−at) −(2/a)∫sin(2t)e^(−at) dt] =−((sin^2 t)/a)∙e^(−at) −((sin(2t))/a^2 )e^(−at) −((2cos(2t))/a^3 )e^(−at) −(4/a^3 )∫sin(2t)e^(−at) dt =−((sin^2 t)/a)∙e^(−at) +(a^3 /(a^2 +4))[−((sin(2t))/a^2 )e^(−at) −((2cos(2t))/a^3 )e^(−at) ]+C ⇒A(t)=tcost−sint+((sin^2 t)/a)∙e^(−at) +(a^3 /(a^2 +4))[((sin(2t))/a^2 )e^(−at) +((2cos(2t))/a^3 )e^(−at) ]+C B(t)=∫((te^(2at) cost+e^(at) sintcost)/e^(2at) )dt=∫(tcost+e^(−at) sintcost)dt ∫tcostdt=tsint−∫sintdt=tsint+cost ∫e^(−at) sintcostdt=(1/2)∫e^(−at) sin(2t)dt =(1/2)∙(a^4 /(a^2 +4))[−((sin(2t))/a^2 )e^(−at) −((2cos(2t))/a^3 )e^(−at) ]+K ⇒B(t)=tsint+cost+(1/2)∙(a^4 /(a^2 +4))[−((sin(2t))/a^2 )e^(−at) −((2cos(2t))/a^3 )e^(−at) ]+K Or y_p =A(t)e^(at) cost+B(t)e^(at) sint et y_G =y_h +y_p y_G =e^(at) (Acost+Bsint)+e^(at) tcos^2 t−e^(at) sintcost+((sin^2 tcost)/a)+((a^3 cost)/(a^2 +4))[((sin(2t))/a^2 )+((2cos(2t))/a^3 )]+Ce^(at) cost e^(at) tsintcost+e^(at) cos^2 t+(1/2)∙((a^4 cost)/(a^2 +4))[−((sin(2t))/a^2 )−((2cos(2t))/a^3 )]+Ke^(at) cost](https://www.tinkutara.com/question/Q113446.png)
$$\mathrm{y}''−\mathrm{2ay}'+\left(\mathrm{1}+\mathrm{a}^{\mathrm{2}} \right)\mathrm{y}=\mathrm{te}^{\mathrm{at}} +\mathrm{sint} \\ $$$$\left(\mathrm{h}\right)\rightarrow\mathrm{r}^{\mathrm{2}} −\mathrm{2ar}+\mathrm{1}+\mathrm{a}^{\mathrm{2}} =\mathrm{0}\Rightarrow\mathrm{r}=\frac{\mathrm{2a}\pm\sqrt{\mathrm{4a}^{\mathrm{2}} −\mathrm{4}\left(\mathrm{1}+\mathrm{a}^{\mathrm{2}} \right)}}{\mathrm{2}}=\mathrm{a}\pm\mathrm{i} \\ $$$$\mathrm{y}_{\mathrm{h}} =\mathrm{e}^{\mathrm{at}} \left(\mathrm{Acost}+\mathrm{Bsint}\right) \\ $$$$\mathrm{By}\:\mathrm{varying}\:\mathrm{parameters}\:\mathrm{let};\:\mathrm{y}_{\mathrm{p}} =\mathrm{A}\left(\mathrm{t}\right)\mathrm{e}^{\mathrm{at}} \mathrm{cost}+\mathrm{B}\left(\mathrm{t}\right)\mathrm{e}^{\mathrm{at}} \mathrm{sint} \\ $$$$\Rightarrow\mathrm{y}_{\mathrm{p}} =\mathrm{au}_{\mathrm{1}} +\mathrm{bu}_{\mathrm{2}} \\ $$$$\begin{cases}{\mathrm{a}'\mathrm{u}_{\mathrm{1}} +\mathrm{b}'\mathrm{u}_{\mathrm{2}} =\mathrm{0}}&{…\left(\mathrm{1}\right)}\\{\mathrm{a}'\mathrm{u}_{\mathrm{1}} '+\mathrm{b}'\mathrm{u}_{\mathrm{2}} '=\mathrm{te}^{\mathrm{at}} +\mathrm{sint}}&{…\left(\mathrm{2}\right)}\end{cases} \\ $$$$\mathrm{Finding}\:\mathrm{a}'\:\mathrm{and}\:\mathrm{b}'\:\:\mathrm{using}\:\mathrm{Crammer}'\mathrm{s}\:\mathrm{method}; \\ $$$$\mathrm{W}\left(\mathrm{u}_{\mathrm{1}} ,\mathrm{u}_{\mathrm{2}} \right)=\begin{vmatrix}{\mathrm{u}_{\mathrm{1}} }&{\mathrm{u}_{\mathrm{2}} }\\{\mathrm{u}_{\mathrm{1}} '}&{\mathrm{u}_{\mathrm{2}} '}\end{vmatrix}=\begin{vmatrix}{\mathrm{e}^{\mathrm{at}} \mathrm{cost}}&{\mathrm{e}^{\mathrm{at}} \mathrm{sint}}\\{\mathrm{ae}^{\mathrm{at}} \mathrm{cost}−\mathrm{e}^{\mathrm{at}} \mathrm{sint}}&{\mathrm{e}^{\mathrm{at}} \mathrm{cost}+\mathrm{ae}^{\mathrm{at}} \mathrm{sint}}\end{vmatrix} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{e}^{\mathrm{2at}} \left[\left(\mathrm{cos}^{\mathrm{2}} \mathrm{t}+\mathrm{asintcost}\right)−\left(\mathrm{asintcost}−\mathrm{sin}^{\mathrm{2}} \mathrm{t}\right)\right]=\mathrm{e}^{\mathrm{2at}} \\ $$$$\mathrm{w}_{\mathrm{1}} =\begin{vmatrix}{\mathrm{0}}&{\mathrm{e}^{\mathrm{at}} \mathrm{sint}}\\{\mathrm{te}^{\mathrm{at}} +\mathrm{sint}}&{\mathrm{e}^{\mathrm{at}} \mathrm{cost}+\mathrm{ae}^{\mathrm{at}} \mathrm{sint}}\end{vmatrix}=−\left(\mathrm{te}^{\mathrm{2at}} \mathrm{sint}+\mathrm{e}^{\mathrm{at}} \mathrm{sin}^{\mathrm{2}} \mathrm{t}\right) \\ $$$$\mathrm{w}_{\mathrm{2}} =\begin{vmatrix}{\mathrm{e}^{\mathrm{at}} \mathrm{cost}}&{\mathrm{0}}\\{\mathrm{ae}^{\mathrm{at}} \mathrm{cost}−\mathrm{e}^{\mathrm{at}} \mathrm{sint}}&{\mathrm{te}^{\mathrm{at}} +\mathrm{sint}}\end{vmatrix}=\mathrm{te}^{\mathrm{2at}} \mathrm{cost}+\mathrm{e}^{\mathrm{at}} \mathrm{sintcost} \\ $$$$\mathrm{a}'=\frac{\mathrm{w}_{\mathrm{1}} }{\mathrm{W}}\Rightarrow\mathrm{a}=\int\frac{\mathrm{w}_{\mathrm{1}} }{\mathrm{W}}\mathrm{dt}\:,\:\mathrm{b}'=\frac{\mathrm{w}_{\mathrm{2}} }{\mathrm{W}}\Rightarrow\mathrm{b}=\int\frac{\mathrm{w}_{\mathrm{2}} }{\mathrm{W}}\mathrm{dt} \\ $$$$ \\ $$$$\Rightarrow\mathrm{A}\left(\mathrm{t}\right)=−\int\frac{\mathrm{te}^{\mathrm{2at}} \mathrm{sint}+\mathrm{e}^{\mathrm{at}} \mathrm{sin}^{\mathrm{2}} \mathrm{t}}{\mathrm{e}^{\mathrm{2at}} }\mathrm{dt},\:\mathrm{B}\left(\mathrm{t}\right)=\int\frac{\mathrm{te}^{\mathrm{2at}} \mathrm{cost}+\mathrm{e}^{\mathrm{at}} \mathrm{sintcost}}{\mathrm{e}^{\mathrm{2at}} }\mathrm{dt} \\ $$$$\mathrm{A}\left(\mathrm{t}\right)=−\int\left(\mathrm{tsint}+\mathrm{e}^{−\mathrm{at}} \mathrm{sin}^{\mathrm{2}} \mathrm{t}\right)\mathrm{dt} \\ $$$$\int\mathrm{tsintdt}=−\mathrm{tcost}+\int\mathrm{costdt}=\mathrm{sint}−\mathrm{tcost} \\ $$$$\int\mathrm{e}^{−\mathrm{at}} \mathrm{sin}^{\mathrm{2}} \mathrm{tdt}=−\frac{\mathrm{sin}^{\mathrm{2}} \mathrm{t}}{\mathrm{a}}\centerdot\mathrm{e}^{−\mathrm{at}} +\frac{\mathrm{2}}{\mathrm{a}}\int\mathrm{sintcost}\centerdot\mathrm{e}^{−\mathrm{at}} \mathrm{dt} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=−\frac{\mathrm{sin}^{\mathrm{2}} \mathrm{t}}{\mathrm{a}}\centerdot\mathrm{e}^{−\mathrm{at}} +\frac{\mathrm{1}}{\mathrm{a}}\int\mathrm{sin}\left(\mathrm{2t}\right)\mathrm{e}^{−\mathrm{at}} \mathrm{dt} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=−\frac{\mathrm{sin}^{\mathrm{2}} \mathrm{t}}{\mathrm{a}}\centerdot\mathrm{e}^{−\mathrm{at}} +\frac{\mathrm{1}}{\mathrm{a}}\left\{−\frac{\mathrm{sin}\left(\mathrm{2t}\right)}{\mathrm{a}}\mathrm{e}^{−\mathrm{at}} +\frac{\mathrm{2}}{\mathrm{a}}\int\mathrm{cos}\left(\mathrm{2t}\right)\mathrm{e}^{−\mathrm{at}} \mathrm{dt}\right\} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=−\frac{\mathrm{sin}^{\mathrm{2}} \mathrm{t}}{\mathrm{a}}\centerdot\mathrm{e}^{−\mathrm{at}} −\frac{\mathrm{sin}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{2}} }\mathrm{e}^{−\mathrm{at}} +\frac{\mathrm{2}}{\mathrm{a}^{\mathrm{2}} }\left[−\frac{\mathrm{cos}\left(\mathrm{2t}\right)}{\mathrm{a}}\mathrm{e}^{−\mathrm{at}} −\frac{\mathrm{2}}{\mathrm{a}}\int\mathrm{sin}\left(\mathrm{2t}\right)\mathrm{e}^{−\mathrm{at}} \mathrm{dt}\right] \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=−\frac{\mathrm{sin}^{\mathrm{2}} \mathrm{t}}{\mathrm{a}}\centerdot\mathrm{e}^{−\mathrm{at}} −\frac{\mathrm{sin}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{2}} }\mathrm{e}^{−\mathrm{at}} −\frac{\mathrm{2cos}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{3}} }\mathrm{e}^{−\mathrm{at}} −\frac{\mathrm{4}}{\mathrm{a}^{\mathrm{3}} }\int\mathrm{sin}\left(\mathrm{2t}\right)\mathrm{e}^{−\mathrm{at}} \mathrm{dt} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=−\frac{\mathrm{sin}^{\mathrm{2}} \mathrm{t}}{\mathrm{a}}\centerdot\mathrm{e}^{−\mathrm{at}} +\frac{\mathrm{a}^{\mathrm{3}} }{\mathrm{a}^{\mathrm{2}} +\mathrm{4}}\left[−\frac{\mathrm{sin}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{2}} }\mathrm{e}^{−\mathrm{at}} −\frac{\mathrm{2cos}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{3}} }\mathrm{e}^{−\mathrm{at}} \right]+\mathrm{C} \\ $$$$\Rightarrow\mathrm{A}\left(\mathrm{t}\right)=\mathrm{tcost}−\mathrm{sint}+\frac{\mathrm{sin}^{\mathrm{2}} \mathrm{t}}{\mathrm{a}}\centerdot\mathrm{e}^{−\mathrm{at}} +\frac{\mathrm{a}^{\mathrm{3}} }{\mathrm{a}^{\mathrm{2}} +\mathrm{4}}\left[\frac{\mathrm{sin}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{2}} }\mathrm{e}^{−\mathrm{at}} +\frac{\mathrm{2cos}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{3}} }\mathrm{e}^{−\mathrm{at}} \right]+\mathrm{C} \\ $$$$\mathrm{B}\left(\mathrm{t}\right)=\int\frac{\mathrm{te}^{\mathrm{2at}} \mathrm{cost}+\mathrm{e}^{\mathrm{at}} \mathrm{sintcost}}{\mathrm{e}^{\mathrm{2at}} }\mathrm{dt}=\int\left(\mathrm{tcost}+\mathrm{e}^{−\mathrm{at}} \mathrm{sintcost}\right)\mathrm{dt} \\ $$$$ \\ $$$$\int\mathrm{tcostdt}=\mathrm{tsint}−\int\mathrm{sintdt}=\mathrm{tsint}+\mathrm{cost} \\ $$$$\int\mathrm{e}^{−\mathrm{at}} \mathrm{sintcostdt}=\frac{\mathrm{1}}{\mathrm{2}}\int\mathrm{e}^{−\mathrm{at}} \mathrm{sin}\left(\mathrm{2t}\right)\mathrm{dt} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}\centerdot\frac{\mathrm{a}^{\mathrm{4}} }{\mathrm{a}^{\mathrm{2}} +\mathrm{4}}\left[−\frac{\mathrm{sin}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{2}} }\mathrm{e}^{−\mathrm{at}} −\frac{\mathrm{2cos}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{3}} }\mathrm{e}^{−\mathrm{at}} \right]+\mathrm{K} \\ $$$$\Rightarrow\mathrm{B}\left(\mathrm{t}\right)=\mathrm{tsint}+\mathrm{cost}+\frac{\mathrm{1}}{\mathrm{2}}\centerdot\frac{\mathrm{a}^{\mathrm{4}} }{\mathrm{a}^{\mathrm{2}} +\mathrm{4}}\left[−\frac{\mathrm{sin}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{2}} }\mathrm{e}^{−\mathrm{at}} −\frac{\mathrm{2cos}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{3}} }\mathrm{e}^{−\mathrm{at}} \right]+\mathrm{K} \\ $$$$\mathrm{Or}\:\mathrm{y}_{\mathrm{p}} =\mathrm{A}\left(\mathrm{t}\right)\mathrm{e}^{\mathrm{at}} \mathrm{cost}+\mathrm{B}\left(\mathrm{t}\right)\mathrm{e}^{\mathrm{at}} \mathrm{sint}\:\mathrm{et}\:\mathrm{y}_{\mathrm{G}} =\mathrm{y}_{\mathrm{h}} +\mathrm{y}_{\mathrm{p}} \\ $$$$\mathrm{y}_{\mathrm{G}} =\mathrm{e}^{\mathrm{at}} \left(\mathrm{Acost}+\mathrm{Bsint}\right)+\mathrm{e}^{\mathrm{at}} \mathrm{tcos}^{\mathrm{2}} \mathrm{t}−\mathrm{e}^{\mathrm{at}} \mathrm{sintcost}+\frac{\mathrm{sin}^{\mathrm{2}} \mathrm{tcost}}{\mathrm{a}}+\frac{\mathrm{a}^{\mathrm{3}} \mathrm{cost}}{\mathrm{a}^{\mathrm{2}} +\mathrm{4}}\left[\frac{\mathrm{sin}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{2}} }+\frac{\mathrm{2cos}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{3}} }\right]+\mathrm{Ce}^{\mathrm{at}} \mathrm{cost} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{e}^{\mathrm{at}} \mathrm{tsintcost}+\mathrm{e}^{\mathrm{at}} \mathrm{cos}^{\mathrm{2}} \mathrm{t}+\frac{\mathrm{1}}{\mathrm{2}}\centerdot\frac{\mathrm{a}^{\mathrm{4}} \mathrm{cost}}{\mathrm{a}^{\mathrm{2}} +\mathrm{4}}\left[−\frac{\mathrm{sin}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{2}} }−\frac{\mathrm{2cos}\left(\mathrm{2t}\right)}{\mathrm{a}^{\mathrm{3}} }\right]+\mathrm{Ke}^{\mathrm{at}} \mathrm{cost} \\ $$
Commented by bemath last updated on 13/Sep/20

$${amazing}\: \\ $$
Commented by bobhans last updated on 13/Sep/20

$$\mathrm{super}\:\mathrm{great}…. \\ $$
Commented by Ar Brandon last updated on 13/Sep/20
��
