Question Number 92468 by jagoll last updated on 07/May/20
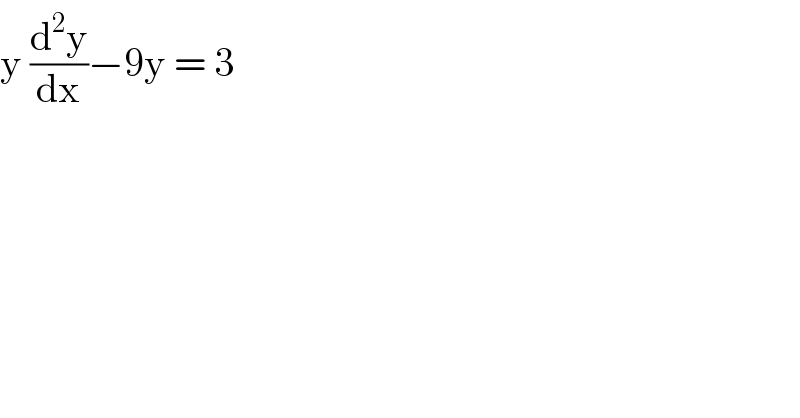
$$\mathrm{y}\:\frac{\mathrm{d}^{\mathrm{2}} \mathrm{y}}{\mathrm{dx}}−\mathrm{9y}\:=\:\mathrm{3} \\ $$
Answered by mr W last updated on 07/May/20
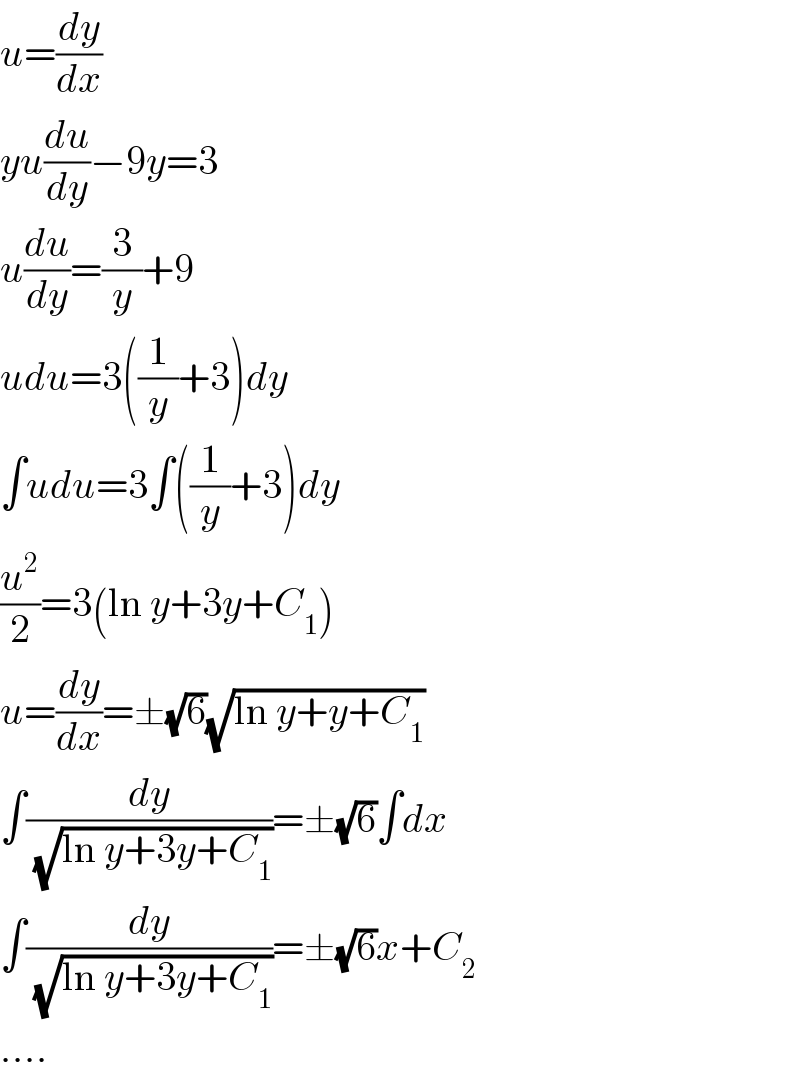
$${u}=\frac{{dy}}{{dx}} \\ $$$${yu}\frac{{du}}{{dy}}−\mathrm{9}{y}=\mathrm{3} \\ $$$${u}\frac{{du}}{{dy}}=\frac{\mathrm{3}}{{y}}+\mathrm{9} \\ $$$${udu}=\mathrm{3}\left(\frac{\mathrm{1}}{{y}}+\mathrm{3}\right){dy} \\ $$$$\int{udu}=\mathrm{3}\int\left(\frac{\mathrm{1}}{{y}}+\mathrm{3}\right){dy} \\ $$$$\frac{{u}^{\mathrm{2}} }{\mathrm{2}}=\mathrm{3}\left(\mathrm{ln}\:{y}+\mathrm{3}{y}+{C}_{\mathrm{1}} \right) \\ $$$${u}=\frac{{dy}}{{dx}}=\pm\sqrt{\mathrm{6}}\sqrt{\mathrm{ln}\:{y}+{y}+{C}_{\mathrm{1}} } \\ $$$$\int\frac{{dy}}{\:\sqrt{\mathrm{ln}\:{y}+\mathrm{3}{y}+{C}_{\mathrm{1}} }}=\pm\sqrt{\mathrm{6}}\int{dx} \\ $$$$\int\frac{{dy}}{\:\sqrt{\mathrm{ln}\:{y}+\mathrm{3}{y}+{C}_{\mathrm{1}} }}=\pm\sqrt{\mathrm{6}}{x}+{C}_{\mathrm{2}} \\ $$$$…. \\ $$
Commented by jagoll last updated on 07/May/20

$$\mathrm{tobe}\:\mathrm{continue}\:\mathrm{sir} \\ $$
Commented by mr W last updated on 07/May/20
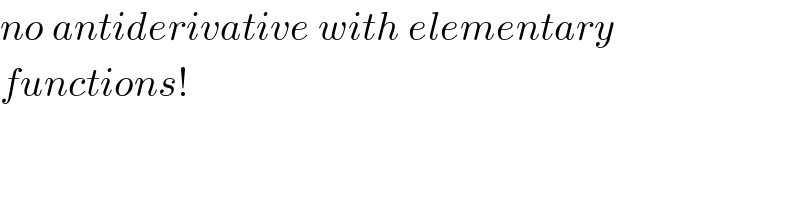
$${no}\:{antiderivative}\:{with}\:{elementary} \\ $$$${functions}! \\ $$
