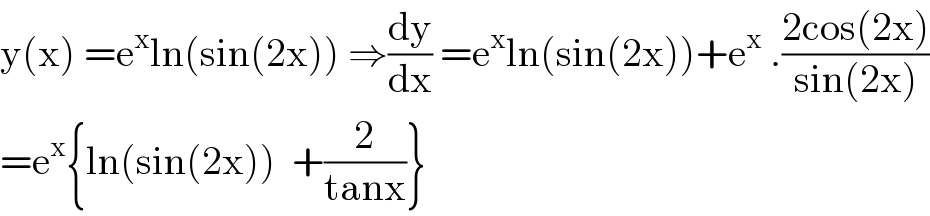y-e-x-ln-sin2x-dy-dx-

Question Number 108238 by Study last updated on 15/Aug/20

y = e^{x} l n (s i n 2 x) \frac{d y}{d x} = ? ?

Answered by Dwaipayan Shikari last updated on 15/Aug/20

\frac{d y}{d x} = e^{x} l o g (s i n 2 x) + 2 e^{x} \frac{c o s 2 x}{s i n 2 x}

\frac{d y}{d x} = e^{x} (l o g (s i n 2 x) + 2 c o t 2 x)

Answered by john santu last updated on 15/Aug/20

\frac{♡ J S ♡}{}

\frac{d y}{d x} = e^{x} \ln (\sin 2 x) + e^{x} (\frac{2 \cos 2 x}{\sin 2 x})

= e^{x} [\ln (\sin 2 x) + 2 \cot 2 x]

Answered by mathdave last updated on 15/Aug/20

s o l u t i o n

\frac{d y}{d x} = e^{x} ∙ 2 \frac{\cos 2 x}{\sin 2 x} + e^{x} \ln (\sin 2 x)

\frac{d y}{d x} - y = 2 e^{x} \cot 2 x

Answered by mathmax by abdo last updated on 15/Aug/20

y (x) = e^{x} \ln (\sin (2 x)) \Rightarrow \frac{dy}{dx} = e^{x} \ln (\sin (2 x)) + e^{x} . \frac{2 \cos (2 x)}{\sin (2 x)}

= e^{x} {\ln (\sin (2 x)) + \frac{2}{tanx}}