0-pi-2-1-1-tan-x-dx-

Question Number 64333 by Chi Mes Try last updated on 16/Jul/19

\underset{0}{\int^{π / 2}} \frac{1}{1 + \tan x} d x =

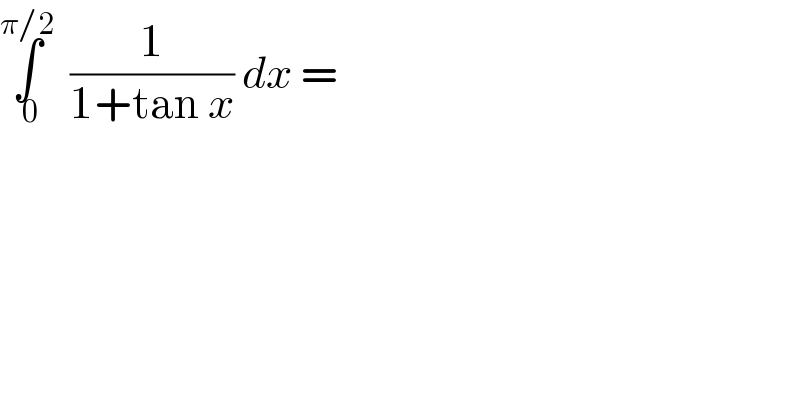
Commented by mathmax by abdo last updated on 16/Jul/19

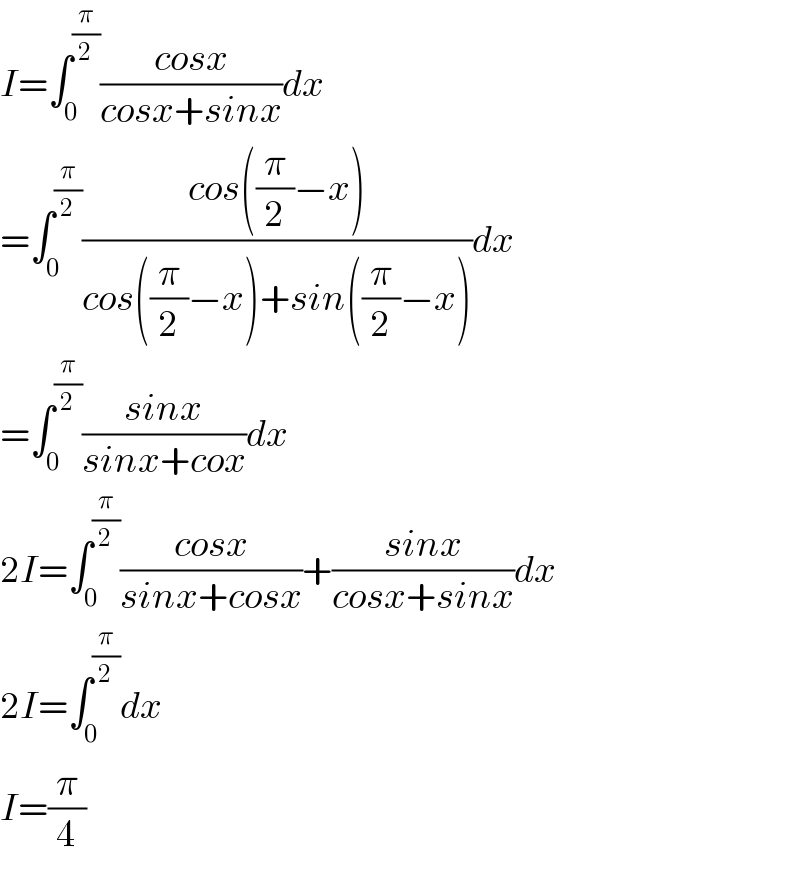
l e t I = \int_{0}^{\frac{π}{2}} \frac{d x}{1 + t a n x} c h a n g e m e n t t a n x = t g i v e

I = \int_{0}^{+ \infty} \frac{d t}{(1 + t^{2}) (1 + t)} l e t d e c o m p o s e F (t) = \frac{1}{(t + 1) (t^{2} + 1)}

F (t) = \frac{a}{t + 1} + \frac{b t + c}{t^{2} + 1}

a = {l i m}_{t \to - 1} (t + 1) F (t) = \frac{1}{2}

{l i m}_{t \to + \infty} t F (t) = 0 = a + b \Rightarrow b = - \frac{1}{2} \Rightarrow F (t) = \frac{1}{2 (t + 1)} + \frac{- \frac{1}{2} t + c}{t^{2} + 1}

F (0) = 1 = \frac{1}{2} + c \Rightarrow c = \frac{1}{2} \Rightarrow F (t) = \frac{1}{2 (t + 1)} - \frac{1}{2} \frac{t - 1}{t^{2} + 1} \Rightarrow

I = \int_{0}^{\infty} (\frac{1}{2 (t + 1)} - \frac{1}{4} \frac{2 t}{t^{2} + 1}) d t + \frac{1}{2} \int_{0}^{\infty} \frac{d t}{t^{2} + 1}

= \frac{1}{2} {[l n ∣ t + 1 ∣ - \frac{1}{2} l n (t^{2} + 1)]}_{0}^{+ \infty} + \frac{π}{4}

= \frac{1}{2} {[l n ∣ \frac{t + 1}{\sqrt{t^{2} + 1}} ∣]}_{0}^{+ \infty} + \frac{π}{4} = 0 + \frac{π}{4} \Rightarrow I = \frac{π}{4} .

Commented by Tony Lin last updated on 17/Jul/19

\int_{0}^{\frac{π}{2}} \frac{1}{1 + t a n x} d x

= \int_{0}^{\frac{π}{2}} \frac{c o s x}{c o s x + s i n x} d x

= \int_{0}^{\frac{π}{2}} \frac{c o s (c o s x - s i n x)}{(c o s x + s i n x) (c o s x - s i n x)} d x

= \int_{0}^{\frac{π}{2}} \frac{{c o s}^{2} x - c o s x s i n x}{{c o s}^{2} x - {s i n}^{2} x} d x

= \frac{1}{2} \int_{0}^{\frac{π}{2}} \frac{c o s 2 x + 1 - s i n 2 x}{c o s 2 x} d x

= \frac{1}{2} \int_{0}^{\frac{π}{2}} d x - \frac{1}{2} \int_{0}^{\frac{π}{2}} t a n 2 x d x + \frac{1}{2} \int_{0}^{\frac{π}{2}} s e c 2 x d x

= \frac{π}{4}

Answered by Rio Michael last updated on 16/Jul/19

b e g i n w i t h

\int \frac{1}{1 + t a n x} d x

I = \int \frac{1}{1 + t a n x} d x u s e t h e s u b s t i t u t i o n u = t a n x

I = \int \frac{1}{(1 + u^{2}) (1 + u)} d u

I = \frac{1}{2} \int (\frac{1}{1 + u^{2}} + \frac{1}{1 + u}) d u

I = \frac{1}{2} \int (\frac{1}{1 + u^{2}} - \frac{1}{2} \frac{2 u}{1 + u^{2}} + \frac{1}{1 + u}) d u

I = \frac{1}{2} (a r c t a n u - \frac{1}{2} l n (1 + u^{2}) + l n (1 + u))

I = ⌈ \frac{1}{2} (x - l n (s e c x) + l n (1 + t a n x)) ⌉_{0}^{\frac{π}{2}}

I = \frac{1}{2} (\frac{π}{2} + l n (s i n \frac{π}{2} + c o s \frac{π}{2})) = \frac{π}{4}

Answered by Tanmay chaudhury last updated on 17/Jul/19

I = \int_{0}^{\frac{π}{2}} \frac{c o s x}{c o s x + s i n x} d x

= \int_{0}^{\frac{π}{2}} \frac{c o s (\frac{π}{2} - x)}{c o s (\frac{π}{2} - x) + s i n (\frac{π}{2} - x)} d x

= \int_{0}^{\frac{π}{2}} \frac{s i n x}{s i n x + c o x} d x

2 I = \int_{0}^{\frac{π}{2}} \frac{c o s x}{s i n x + c o s x} + \frac{s i n x}{c o s x + s i n x} d x

2 I = \int_{0}^{\frac{π}{2}} d x

I = \frac{π}{4}