Menu Close

A-train-150-metres-long-completely-passes-a-boy-walking-in-the-opposite-direction-at-6-kmph-in-9-seconds-and-a-car-travelling-in-the-opposite-direction-in-6-sec-Find-the-speed-of-the-car-

Tinku Tara 0 Comments
Pin




Question Number 4891 by Rasheed Soomro last updated on 19/Mar/16
A train 150 metres long completely passes a boy walking in the opposite direction at 6 kmph in 9 seconds and a car travelling in the opposite direction in 6 sec. Find the speed of the car.
$$\mathrm{A}\:\mathrm{train}\:\mathrm{150}\:\mathrm{metres}\:\mathrm{long}\:\mathrm{completely} \\ $$$$\mathrm{passes}\:\mathrm{a}\:\mathrm{boy}\:\mathrm{walking}\:\mathrm{in}\:\mathrm{the}\:\mathrm{opposite} \\ $$$$\mathrm{direction}\:\mathrm{at}\:\mathrm{6}\:\mathrm{kmph}\:\mathrm{in}\:\mathrm{9}\:\mathrm{seconds}\:\mathrm{and} \\ $$$$\mathrm{a}\:\mathrm{car}\:\:\mathrm{travelling}\:\:\mathrm{in}\:\mathrm{the}\:\:\mathrm{opposite}\: \\ $$$$\mathrm{direction}\:\mathrm{in}\:\mathrm{6}\:\mathrm{sec}.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{speed}\:\mathrm{of} \\ $$$$\mathrm{the}\:\mathrm{car}. \\ $$
Commented by prakash jain last updated on 19/Mar/16
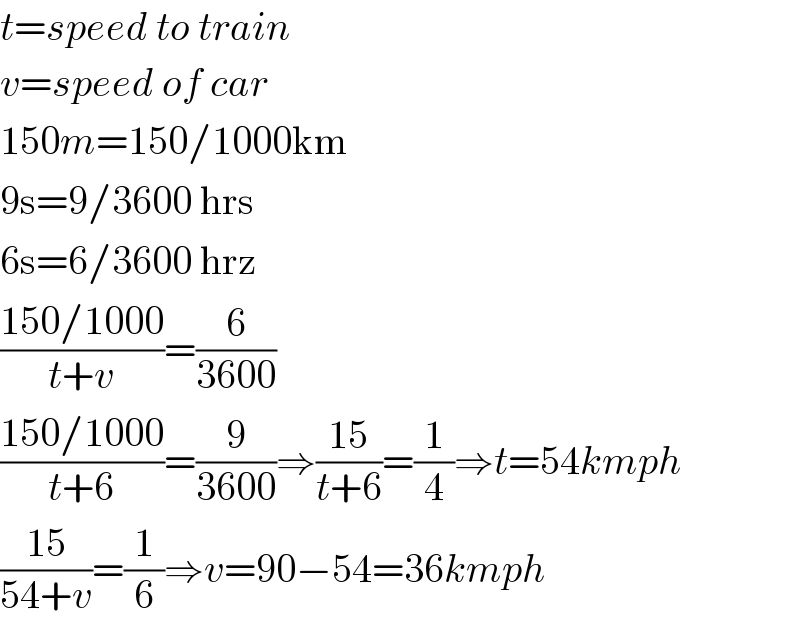
t=speed to train v=speed of car 150m=150/1000km 9s=9/3600 hrs 6s=6/3600 hrz ((150/1000)/(t+v))=(6/(3600)) ((150/1000)/(t+6))=(9/(3600))⇒((15)/(t+6))=(1/4)⇒t=54kmph ((15)/(54+v))=(1/6)⇒v=90−54=36kmph
$${t}={speed}\:{to}\:{train} \\ $$$${v}={speed}\:{of}\:{car} \\ $$$$\mathrm{150}{m}=\mathrm{150}/\mathrm{1000km} \\ $$$$\mathrm{9s}=\mathrm{9}/\mathrm{3600}\:\mathrm{hrs} \\ $$$$\mathrm{6s}=\mathrm{6}/\mathrm{3600}\:\mathrm{hrz} \\ $$$$\frac{\mathrm{150}/\mathrm{1000}}{{t}+{v}}=\frac{\mathrm{6}}{\mathrm{3600}} \\ $$$$\frac{\mathrm{150}/\mathrm{1000}}{{t}+\mathrm{6}}=\frac{\mathrm{9}}{\mathrm{3600}}\Rightarrow\frac{\mathrm{15}}{{t}+\mathrm{6}}=\frac{\mathrm{1}}{\mathrm{4}}\Rightarrow{t}=\mathrm{54}{kmph} \\ $$$$\frac{\mathrm{15}}{\mathrm{54}+{v}}=\frac{\mathrm{1}}{\mathrm{6}}\Rightarrow{v}=\mathrm{90}−\mathrm{54}=\mathrm{36}{kmph} \\ $$
Answered by Yozzii last updated on 19/Mar/16
Consider the relative velocity between the train and the boy. Their velocities satisfy v−u_b =c where c is the velocity with which the train passes the boy from the train′s perception of the boy while the boy is in motion. The value of c is actually given by c=((length of train )/(time for the train to move pass the boy (or vice versa))) c=((150/1000)/(9/3600))=((15)/1)×((36)/9)=60kmph So,the train approaches/passes the boy with an apparent velocity of 60kmph from the perspective of the train. The train moves opposite in direction to the boy. So let v be the positive direction and u_b =−6 kmph be the negative direction. ∴v=60−6=54kmph. For the relative velocity between the train and the car we have once more an equation of the form v−v_c =n. Here, n=((length of train)/(time taken for the train to pass the car)) n=((150/1000)/(6/3600))=((15)/1)×(6/1)=90kmph. Hence, the car moves with velocity v_c =54−90=−36kmph. The speed of the car is 36kmph.
$${Consider}\:{the}\:{relative}\:{velocity}\:{between} \\ $$$${the}\:{train}\:{and}\:{the}\:{boy}.\:{Their}\:{velocities} \\ $$$${satisfy}\:\:{v}−{u}_{{b}} ={c}\:\:{where}\:{c}\:{is}\:{the}\: \\ $$$${velocity}\:{with}\:{which}\:{the}\:{train}\:{passes} \\ $$$${the}\:{boy}\:{from}\:{the}\:{train}'{s}\:{perception} \\ $$$${of}\:{the}\:{boy}\:{while}\:{the}\:{boy}\:{is}\:{in}\:{motion}. \\ $$$${The}\:{value}\:{of}\:{c}\:{is}\:{actually}\:{given}\:{by} \\ $$$${c}=\frac{{length}\:{of}\:{train}\:}{{time}\:{for}\:{the}\:{train}\:{to}\:{move}\:{pass}\:{the}\:{boy}\:\left({or}\:{vice}\:{versa}\right)} \\ $$$${c}=\frac{\mathrm{150}/\mathrm{1000}}{\mathrm{9}/\mathrm{3600}}=\frac{\mathrm{15}}{\mathrm{1}}×\frac{\mathrm{36}}{\mathrm{9}}=\mathrm{60}{kmph} \\ $$$${So},{the}\:{train}\:{approaches}/{passes}\:{the} \\ $$$${boy}\:{with}\:{an}\:{apparent}\:{velocity}\:{of}\:\mathrm{60}{kmph} \\ $$$${from}\:{the}\:{perspective}\:{of}\:{the}\:{train}. \\ $$$${The}\:{train}\:{moves}\:{opposite}\:{in}\:{direction} \\ $$$${to}\:{the}\:{boy}.\:{So}\:{let}\:{v}\:{be}\:{the}\:{positive} \\ $$$${direction}\:{and}\:{u}_{{b}} =−\mathrm{6}\:{kmph}\:{be} \\ $$$${the}\:{negative}\:{direction}. \\ $$$$\therefore{v}=\mathrm{60}−\mathrm{6}=\mathrm{54}{kmph}. \\ $$$${For}\:{the}\:{relative}\:{velocity}\:{between}\:{the} \\ $$$${train}\:{and}\:{the}\:{car}\:{we}\:{have}\:{once}\:{more} \\ $$$${an}\:{equation}\:{of}\:{the}\:{form} \\ $$$$\:\:\:{v}−{v}_{{c}} ={n}. \\ $$$${Here},\:{n}=\frac{{length}\:{of}\:{train}}{{time}\:{taken}\:{for}\:{the}\:{train}\:{to}\:{pass}\:{the}\:{car}} \\ $$$${n}=\frac{\mathrm{150}/\mathrm{1000}}{\mathrm{6}/\mathrm{3600}}=\frac{\mathrm{15}}{\mathrm{1}}×\frac{\mathrm{6}}{\mathrm{1}}=\mathrm{90}{kmph}. \\ $$$${Hence},\:{the}\:{car}\:{moves}\:{with}\:{velocity} \\ $$$${v}_{{c}} =\mathrm{54}−\mathrm{90}=−\mathrm{36}{kmph}. \\ $$$${The}\:{speed}\:{of}\:{the}\:{car}\:{is}\:\mathrm{36}{kmph}. \\ $$$$ \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *