Question Number 53359 by gunawan last updated on 20/Jan/19
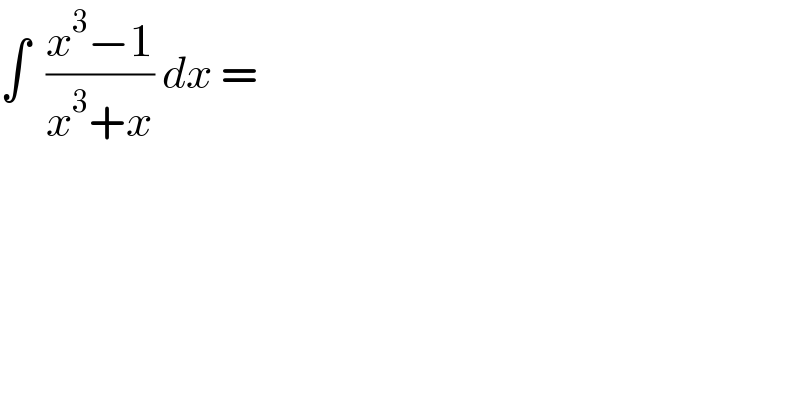
$$\int\:\:\frac{{x}^{\mathrm{3}} −\mathrm{1}}{{x}^{\mathrm{3}} +{x}}\:{dx}\:= \\ $$
Answered by Smail last updated on 21/Jan/19

$${I}=\int\frac{{x}^{\mathrm{3}} +{x}−{x}−\mathrm{1}}{{x}^{\mathrm{3}} +{x}}{dx}=\int\left(\mathrm{1}−\frac{{x}+\mathrm{1}}{{x}\left({x}^{\mathrm{2}} +\mathrm{1}\right)}\right){dx} \\ $$$$={x}−\int\frac{{x}+\mathrm{1}}{{x}\left({x}^{\mathrm{2}} +\mathrm{1}\right)}{dx}+{c}_{\mathrm{1}} \\ $$$$\frac{{x}+\mathrm{1}}{{x}\left({x}^{\mathrm{2}} +\mathrm{1}\right)}=\frac{{a}}{{x}}+\frac{{bx}+{c}}{{x}^{\mathrm{2}} +\mathrm{1}} \\ $$$${a}=−{b}={c}=\mathrm{1} \\ $$$${I}={x}−\int\left(\frac{\mathrm{1}}{{x}}−\frac{{x}−\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{1}}\right){dx}+{c}_{\mathrm{1}} \\ $$$$={x}−{ln}\mid{x}\mid+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\mathrm{1}}−{tan}^{−\mathrm{1}} \left({x}\right)+{c}_{\mathrm{2}} \\ $$$$={x}−{ln}\mid{x}\mid+\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({x}^{\mathrm{2}} +\mathrm{1}\right)−{tan}^{−\mathrm{1}} \left({x}\right)+{C} \\ $$$${I}={x}+{ln}\mid\frac{\sqrt{{x}^{\mathrm{2}} +\mathrm{1}}}{{x}}\mid−{tan}^{−\mathrm{1}} \left({x}\right)+{C} \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 21/Jan/19
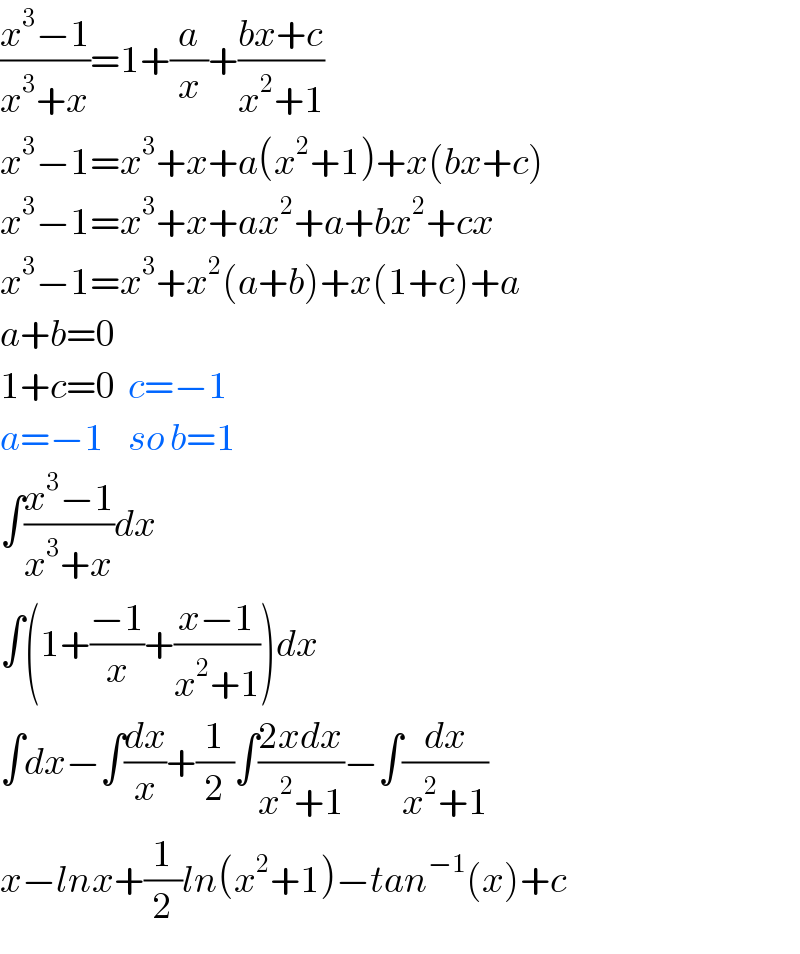
$$\frac{{x}^{\mathrm{3}} −\mathrm{1}}{{x}^{\mathrm{3}} +{x}}=\mathrm{1}+\frac{{a}}{{x}}+\frac{{bx}+{c}}{{x}^{\mathrm{2}} +\mathrm{1}} \\ $$$${x}^{\mathrm{3}} −\mathrm{1}={x}^{\mathrm{3}} +{x}+{a}\left({x}^{\mathrm{2}} +\mathrm{1}\right)+{x}\left({bx}+{c}\right) \\ $$$${x}^{\mathrm{3}} −\mathrm{1}={x}^{\mathrm{3}} +{x}+{ax}^{\mathrm{2}} +{a}+{bx}^{\mathrm{2}} +{cx} \\ $$$${x}^{\mathrm{3}} −\mathrm{1}={x}^{\mathrm{3}} +{x}^{\mathrm{2}} \left({a}+{b}\right)+{x}\left(\mathrm{1}+{c}\right)+{a} \\ $$$${a}+{b}=\mathrm{0} \\ $$$$\mathrm{1}+{c}=\mathrm{0}\:\:{c}=−\mathrm{1} \\ $$$${a}=−\mathrm{1}\:\:\:\:{so}\:{b}=\mathrm{1} \\ $$$$\int\frac{{x}^{\mathrm{3}} −\mathrm{1}}{{x}^{\mathrm{3}} +{x}}{dx} \\ $$$$\int\left(\mathrm{1}+\frac{−\mathrm{1}}{{x}}+\frac{{x}−\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{1}}\right){dx} \\ $$$$\int{dx}−\int\frac{{dx}}{{x}}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{2}{xdx}}{{x}^{\mathrm{2}} +\mathrm{1}}−\int\frac{{dx}}{{x}^{\mathrm{2}} +\mathrm{1}} \\ $$$${x}−{lnx}+\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({x}^{\mathrm{2}} +\mathrm{1}\right)−{tan}^{−\mathrm{1}} \left({x}\right)+{c} \\ $$
