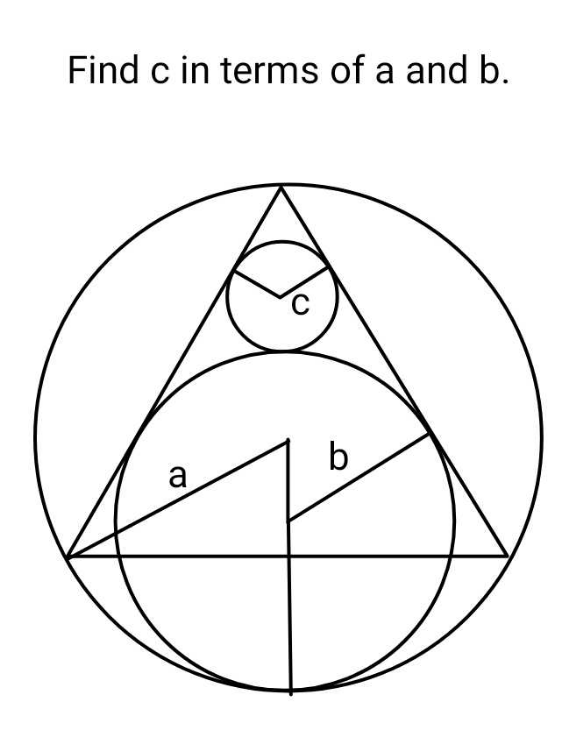
Question Number 194056 by ajfour last updated on 26/Jun/23
Answered by a.lgnaoui last updated on 27/Jun/23
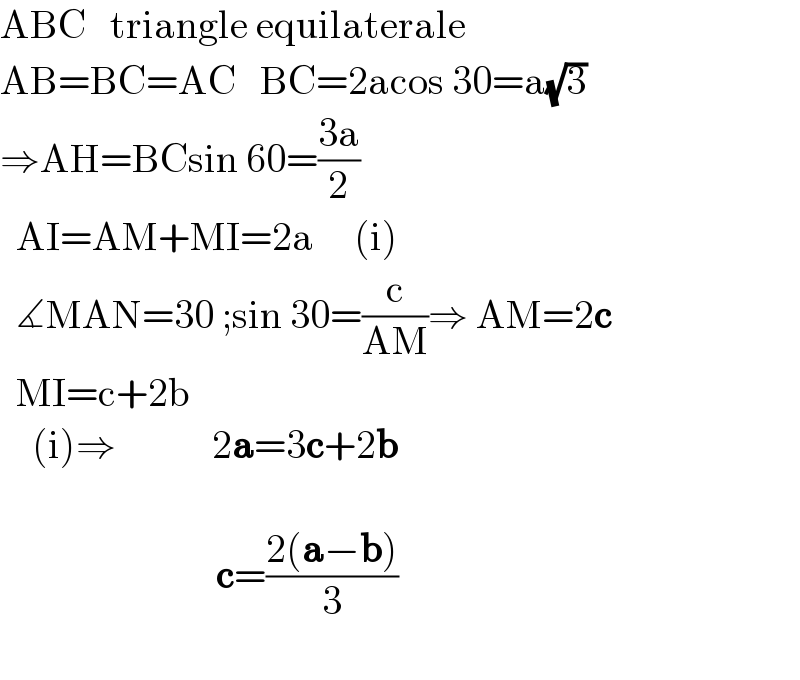
$$\mathrm{ABC}\:\:\:\mathrm{triangle}\:\mathrm{equilaterale}\:\: \\ $$$$\mathrm{AB}=\mathrm{BC}=\mathrm{AC}\:\:\:\mathrm{BC}=\mathrm{2acos}\:\mathrm{30}=\mathrm{a}\sqrt{\mathrm{3}} \\ $$$$\Rightarrow\mathrm{AH}=\mathrm{BCsin}\:\mathrm{60}=\frac{\mathrm{3a}}{\mathrm{2}} \\ $$$$\:\:\mathrm{AI}=\mathrm{AM}+\mathrm{MI}=\mathrm{2a}\:\:\:\:\:\left(\mathrm{i}\right) \\ $$$$\:\:\measuredangle\mathrm{MAN}=\mathrm{30}\:;\mathrm{sin}\:\mathrm{30}=\frac{\mathrm{c}}{\mathrm{AM}}\Rightarrow\:\mathrm{AM}=\mathrm{2}\boldsymbol{\mathrm{c}} \\ $$$$\:\:\mathrm{MI}=\mathrm{c}+\mathrm{2b}\: \\ $$$$\:\:\:\:\left(\mathrm{i}\right)\Rightarrow\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}\boldsymbol{\mathrm{a}}=\mathrm{3}\boldsymbol{\mathrm{c}}+\mathrm{2}\boldsymbol{\mathrm{b}} \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{c}}=\frac{\mathrm{2}\left(\boldsymbol{\mathrm{a}}−\boldsymbol{\mathrm{b}}\right)}{\mathrm{3}} \\ $$$$ \\ $$
Commented by ajfour last updated on 27/Jun/23

$${x}+\mathrm{3}={y} \\ $$$$\Rightarrow\:\:\:{x}=\mathrm{0}\:\:{because}\:\: \\ $$$${y}\:\:{should}\:{be}\:\mathrm{3}. \\ $$
Commented by a.lgnaoui last updated on 27/Jun/23
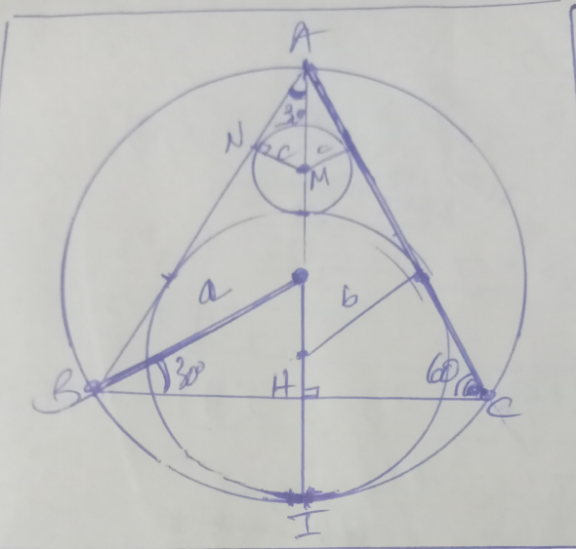
Commented by mr W last updated on 27/Jun/23
$${triangle}\:{is}\:{not}\:{equilateral}! \\ $$
Answered by mr W last updated on 27/Jun/23

$$\frac{{c}}{{b}}=\frac{\mathrm{2}{a}−\mathrm{2}{b}−{c}}{\mathrm{2}{a}−{b}} \\ $$$$\Rightarrow{c}=\frac{\left({a}−{b}\right){b}}{{a}} \\ $$
Commented by ajfour last updated on 30/Jun/23

$${Excellent} \\ $$
