Given-three-Real-numbers-x-y-z-such-that-x-2-y-2-z-2-1-maximize-x-4-y-4-2z-4-3-2-xyz-

Question Number 195570 by York12 last updated on 05/Aug/23

Given three Real numbers (x, y, z), s u c h t h a t

x^{2} + y^{2} + z^{2} = 1

m a x i m i z e

x^{4} + y^{4} - 2 z^{4} - 3 \sqrt{2} x y z

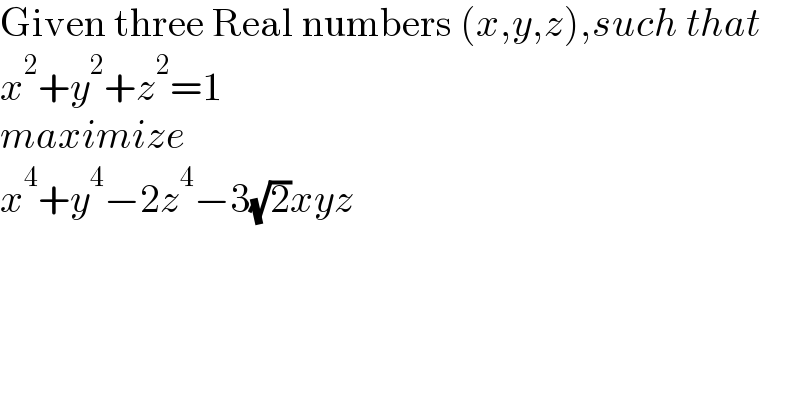
Commented by Frix last updated on 05/Aug/23

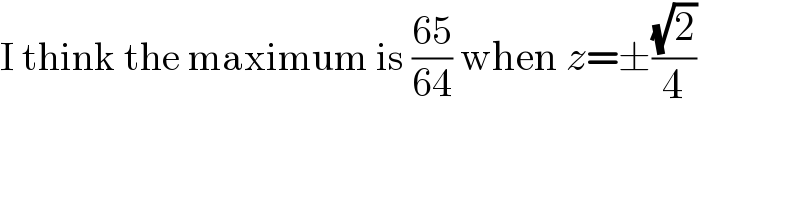
I think the maximum is ((65)/(64)) when z=±((√2)/4)

I think the maximum is \frac{65}{64} when z = \pm \frac{\sqrt{2}}{4}

Commented by York12 last updated on 05/Aug/23

p l e a s e p r o v i d e w o r k i n g s

Answered by Frix last updated on 05/Aug/23

x^2 +y^2 =1−z^2 ⇒ x^4 +y^4 =−2x^2 y^2 +z^4 −2z^2 +1 x^4 +y^4 −2z^4 −3(√2)xyz= =−2x^2 y^2 −3(√2)xyz−z^4 −2z^2 +1 Let t=xy −2t^2 −2(√2)tz−z^4 −2z^2 +1 ((d[−2t^2 −2(√2)tz−z^4 −2z^2 +1])/dt)=0 −4t−3(√2)z=0 ⇒ t=−((3(√2)z)/4) Inserting −z^4 +(z^2 /4)+1 ((d[−z^4 +(z^2 /4)+1])/dz)=0 −4z^3 +(z/2)=0 ⇒ z=±((√2)/4) [∨z=0_(minimum) ^(rejected because) ] ⇒ maximum is ((65)/(64)) ★ We have z=((√2)/4)∧xy=−(3/8) ∨ z=−((√2)/4)∧xy=(3/8) x^2 +y^2 =1−z^2 =(7/8) The values are easy to calculate

x^{2} + y^{2} = 1 - z^{2} \Rightarrow x^{4} + y^{4} = - 2 x^{2} y^{2} + z^{4} - 2 z^{2} + 1

x^{4} + y^{4} - 2 z^{4} - 3 \sqrt{2} x y z =

= - 2 x^{2} y^{2} - 3 \sqrt{2} x y z - z^{4} - 2 z^{2} + 1

Let t = x y

- 2 t^{2} - 2 \sqrt{2} t z - z^{4} - 2 z^{2} + 1

\frac{d [- 2 t^{2} - 2 \sqrt{2} t z - z^{4} - 2 z^{2} + 1]}{d t} = 0

- 4 t - 3 \sqrt{2} z = 0 \Rightarrow t = - \frac{3 \sqrt{2} z}{4}

Inserting

- z^{4} + \frac{z^{2}}{4} + 1

\frac{d [- z^{4} + \frac{z^{2}}{4} + 1]}{d z} = 0

- 4 z^{3} + \frac{z}{2} = 0 \Rightarrow z = \pm \frac{\sqrt{2}}{4} [\lor z = 0_{minimum}^{rejected because}]

\Rightarrow maximum is \frac{65}{64} ★

We have

z = \frac{\sqrt{2}}{4} \land x y = - \frac{3}{8} \lor z = - \frac{\sqrt{2}}{4} \land x y = \frac{3}{8}

x^{2} + y^{2} = 1 - z^{2} = \frac{7}{8}

The values are easy to calculate

Commented by York12 last updated on 05/Aug/23

O R Z

Commented by York12 last updated on 05/Aug/23

where to learn inequalities and are you using discord I would like to invite you to our community

w h e r e t o l e a r n i n e q u a l i t i e s

a n d a r e y o u u s i n g d i s c o r d

I w o u l d l i k e t o i n v i t e y o u t o o u r c o m m u n i t y

Commented by Frix last updated on 05/Aug/23

Sorry I don't use any social media platforms. I studied mathematics & physics decades ago at a university, I don't know where else you could learn these things.

Commented by York12 last updated on 05/Aug/23

o k a y s i r t h a n k s

Commented by York12 last updated on 05/Aug/23

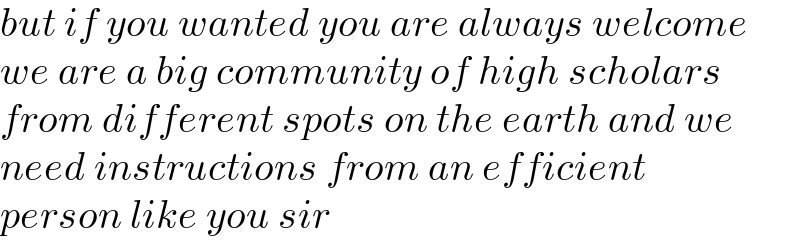
but if you wanted you are always welcome we are a big community of high scholars from different spots on the earth and we need instructions from an efficient person like you sir

b u t i f y o u w a n t e d y o u a r e a l w a y s w e l c o m e

w e a r e a b i g c o m m u n i t y o f h i g h s c h o l a r s

f r o m d i f f e r e n t s p o t s o n t h e e a r t h a n d w e

n e e d i n s t r u c t i o n s f r o m a n e f f i c i e n t

p e r s o n l i k e y o u s i r

Commented by necx122 last updated on 06/Aug/23

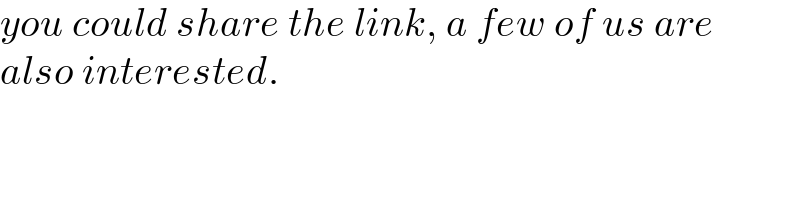
you could share the link, a few of us are also interested.

y o u c o u l d s h a r e t h e l i n k, a f e w o f u s a r e

a l s o i n t e r e s t e d .

Commented by York12 last updated on 06/Aug/23

g r e a t

Commented by York12 last updated on 06/Aug/23

https://discord.gg/mods

Facebook Tweet Pin

Given-three-Real-numbers-x-y-z-such-that-x-2-y-2-z-2-1-maximize-x-4-y-4-2z-4-3-2-xyz-

Leave a Reply Cancel reply