Question Number 196084 by Mingma last updated on 17/Aug/23
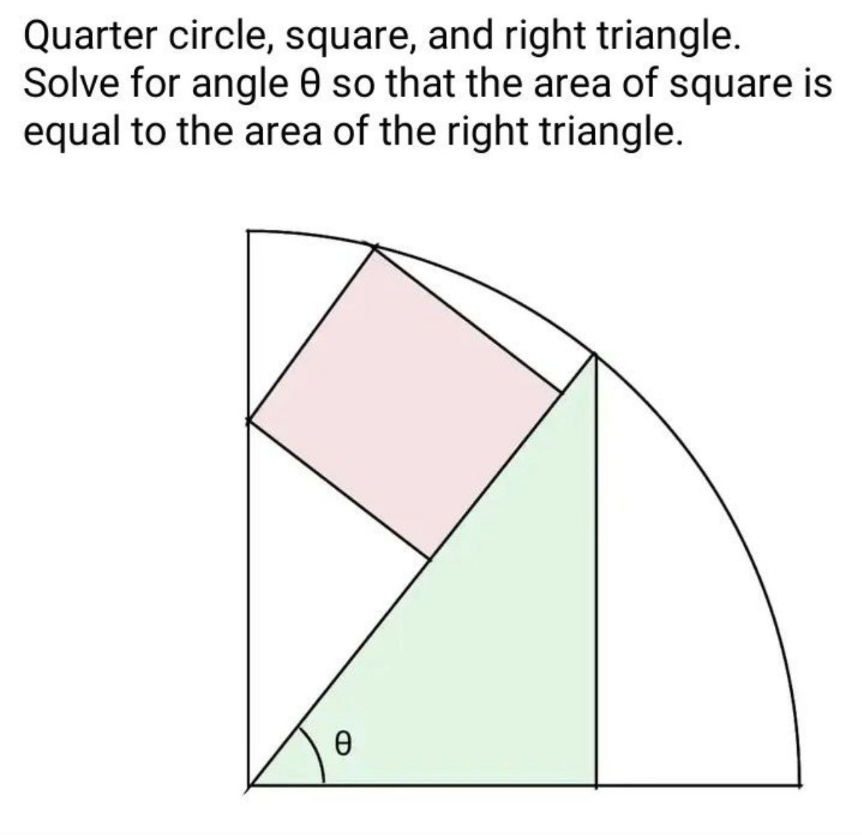
Answered by mr W last updated on 17/Aug/23
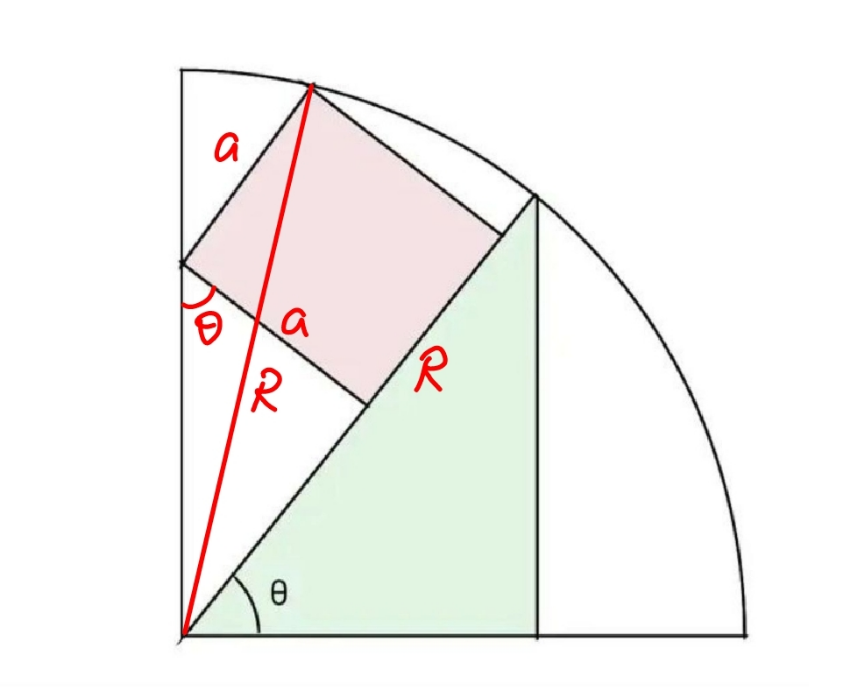
Commented by mr W last updated on 17/Aug/23
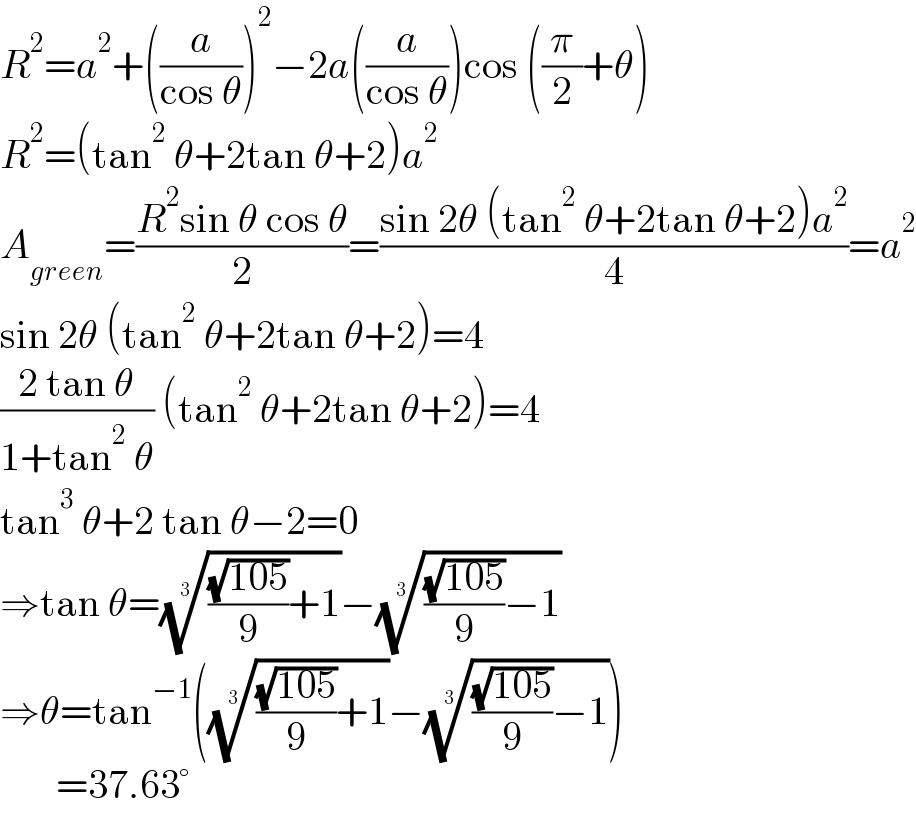
$${R}^{\mathrm{2}} ={a}^{\mathrm{2}} +\left(\frac{{a}}{\mathrm{cos}\:\theta}\right)^{\mathrm{2}} −\mathrm{2}{a}\left(\frac{{a}}{\mathrm{cos}\:\theta}\right)\mathrm{cos}\:\left(\frac{\pi}{\mathrm{2}}+\theta\right) \\ $$$${R}^{\mathrm{2}} =\left(\mathrm{tan}^{\mathrm{2}} \:\theta+\mathrm{2tan}\:\theta+\mathrm{2}\right){a}^{\mathrm{2}} \\ $$$${A}_{{green}} =\frac{{R}^{\mathrm{2}} \mathrm{sin}\:\theta\:\mathrm{cos}\:\theta}{\mathrm{2}}=\frac{\mathrm{sin}\:\mathrm{2}\theta\:\left(\mathrm{tan}^{\mathrm{2}} \:\theta+\mathrm{2tan}\:\theta+\mathrm{2}\right){a}^{\mathrm{2}} }{\mathrm{4}}={a}^{\mathrm{2}} \\ $$$$\mathrm{sin}\:\mathrm{2}\theta\:\left(\mathrm{tan}^{\mathrm{2}} \:\theta+\mathrm{2tan}\:\theta+\mathrm{2}\right)=\mathrm{4} \\ $$$$\frac{\mathrm{2}\:\mathrm{tan}\:\theta}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \:\theta}\:\left(\mathrm{tan}^{\mathrm{2}} \:\theta+\mathrm{2tan}\:\theta+\mathrm{2}\right)=\mathrm{4} \\ $$$$\mathrm{tan}^{\mathrm{3}} \:\theta+\mathrm{2}\:\mathrm{tan}\:\theta−\mathrm{2}=\mathrm{0} \\ $$$$\Rightarrow\mathrm{tan}\:\theta=\sqrt[{\mathrm{3}}]{\frac{\sqrt{\mathrm{105}}}{\mathrm{9}}+\mathrm{1}}−\sqrt[{\mathrm{3}}]{\frac{\sqrt{\mathrm{105}}}{\mathrm{9}}−\mathrm{1}} \\ $$$$\Rightarrow\theta=\mathrm{tan}^{−\mathrm{1}} \left(\sqrt[{\mathrm{3}}]{\frac{\sqrt{\mathrm{105}}}{\mathrm{9}}+\mathrm{1}}−\sqrt[{\mathrm{3}}]{\frac{\sqrt{\mathrm{105}}}{\mathrm{9}}−\mathrm{1}}\right) \\ $$$$\:\:\:\:\:\:\:=\mathrm{37}.\mathrm{63}° \\ $$
Commented by Mingma last updated on 18/Aug/23
Perfect sir!
