Question Number 196257 by mathlove last updated on 21/Aug/23
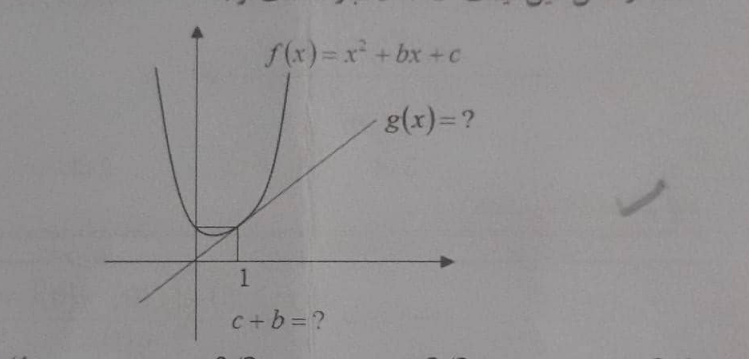
Answered by cortano12 last updated on 21/Aug/23

$$\:\:\mathrm{g}\left(\mathrm{x}\right)\:\Rightarrow\mathrm{m}\:=\:\mathrm{2}+\mathrm{b}\:\mathrm{and}\:\mathrm{passes}\:\mathrm{through} \\ $$$$\:\mathrm{the}\:\mathrm{point}\:\left(\mathrm{1},\:\mathrm{1}+\mathrm{b}+\mathrm{c}\right) \\ $$$$\:\Rightarrow\mathrm{y}−\left(\mathrm{1}+\mathrm{b}+\mathrm{c}\right)=\:\left(\mathrm{2}+\mathrm{b}\right)\left(\mathrm{x}−\mathrm{1}\right) \\ $$$$\:\Rightarrow\mathrm{y}=\:\left(\mathrm{2}+\mathrm{b}\right)\mathrm{x}−\mathrm{2}−\mathrm{b}+\mathrm{1}+\mathrm{b}+\mathrm{c} \\ $$$$\:\Rightarrow\mathrm{y}=\:\left(\mathrm{2}+\mathrm{b}\right)\mathrm{x}+\mathrm{c}−\mathrm{1} \\ $$$$\:\left(\mathrm{0},\mathrm{0}\right)\Rightarrow\mathrm{0}\:=\:\mathrm{c}−\mathrm{1}\:;\:\mathrm{c}\:=\:\mathrm{1} \\ $$$$\:\Rightarrow\mathrm{g}\left(\mathrm{x}\right)=\:\left(\mathrm{2}+\mathrm{b}\right)\mathrm{x}\: \\ $$$$\:\mathrm{passes}\:\mathrm{througt}\:\mathrm{the}\:\mathrm{point}\:\left(\mathrm{1},\mathrm{1}\right) \\ $$$$\:\Rightarrow\mathrm{1}\:=\:\left(\mathrm{2}+\mathrm{b}\right).\mathrm{1}\:\Rightarrow\mathrm{b}=−\mathrm{1} \\ $$$$\:\:\begin{cases}{\mathrm{f}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}}\\{\mathrm{g}\left(\mathrm{x}\right)=\:\mathrm{x}\:}\end{cases}\:\Rightarrow\mathrm{c}+\mathrm{b}\:=\:\mathrm{0}\:\: \\ $$$$\:\:\: \\ $$
Commented by mathlove last updated on 21/Aug/23

$${thanks} \\ $$
