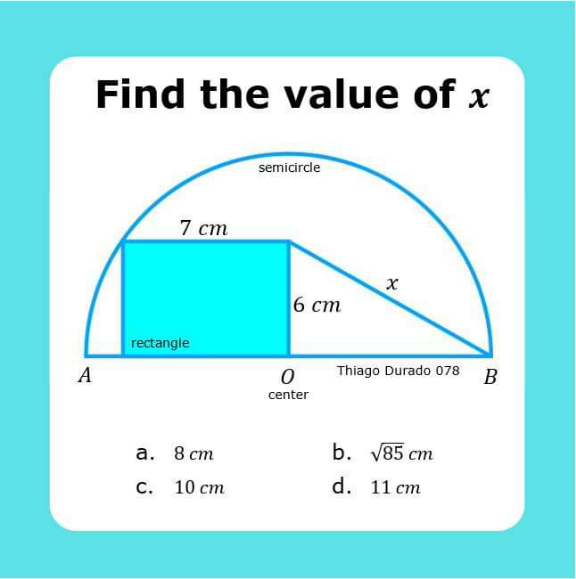
Question Number 196571 by cortano12 last updated on 27/Aug/23
Answered by som(math1967) last updated on 27/Aug/23
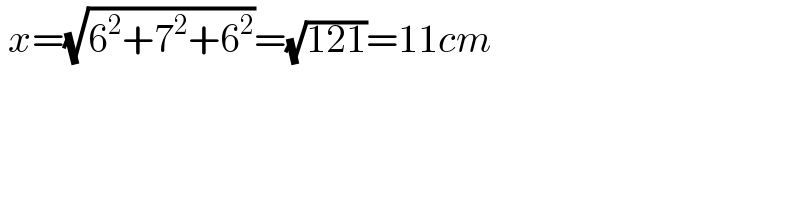
$$\:{x}=\sqrt{\mathrm{6}^{\mathrm{2}} +\mathrm{7}^{\mathrm{2}} +\mathrm{6}^{\mathrm{2}} }=\sqrt{\mathrm{121}}=\mathrm{11}{cm} \\ $$
Answered by hafeez last updated on 27/Aug/23
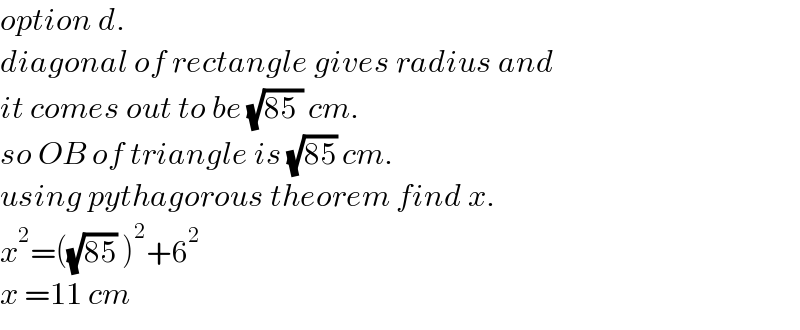
$${option}\:{d}. \\ $$$${diagonal}\:{of}\:{rectangle}\:{gives}\:{radius}\:{and}\: \\ $$$${it}\:{comes}\:{out}\:{to}\:{be}\:\sqrt{\mathrm{85}\:}\:{cm}. \\ $$$${so}\:{OB}\:{of}\:{triangle}\:{is}\:\sqrt{\mathrm{85}}\:{cm}. \\ $$$${using}\:{pythagorous}\:{theorem}\:{find}\:{x}. \\ $$$${x}^{\mathrm{2}} =\left(\sqrt{\mathrm{85}}\:\right)^{\mathrm{2}} +\mathrm{6}^{\mathrm{2}} \\ $$$${x}\:=\mathrm{11}\:{cm} \\ $$
