sir-number-of-3-digit-numbers-which-are-divisible-by-a-3-b-4-c-6-d-7-e-8-f-9-g-11-when-repetetion-is-1-Allowwd-2-Not-allowed-kindly-help-me-sir-

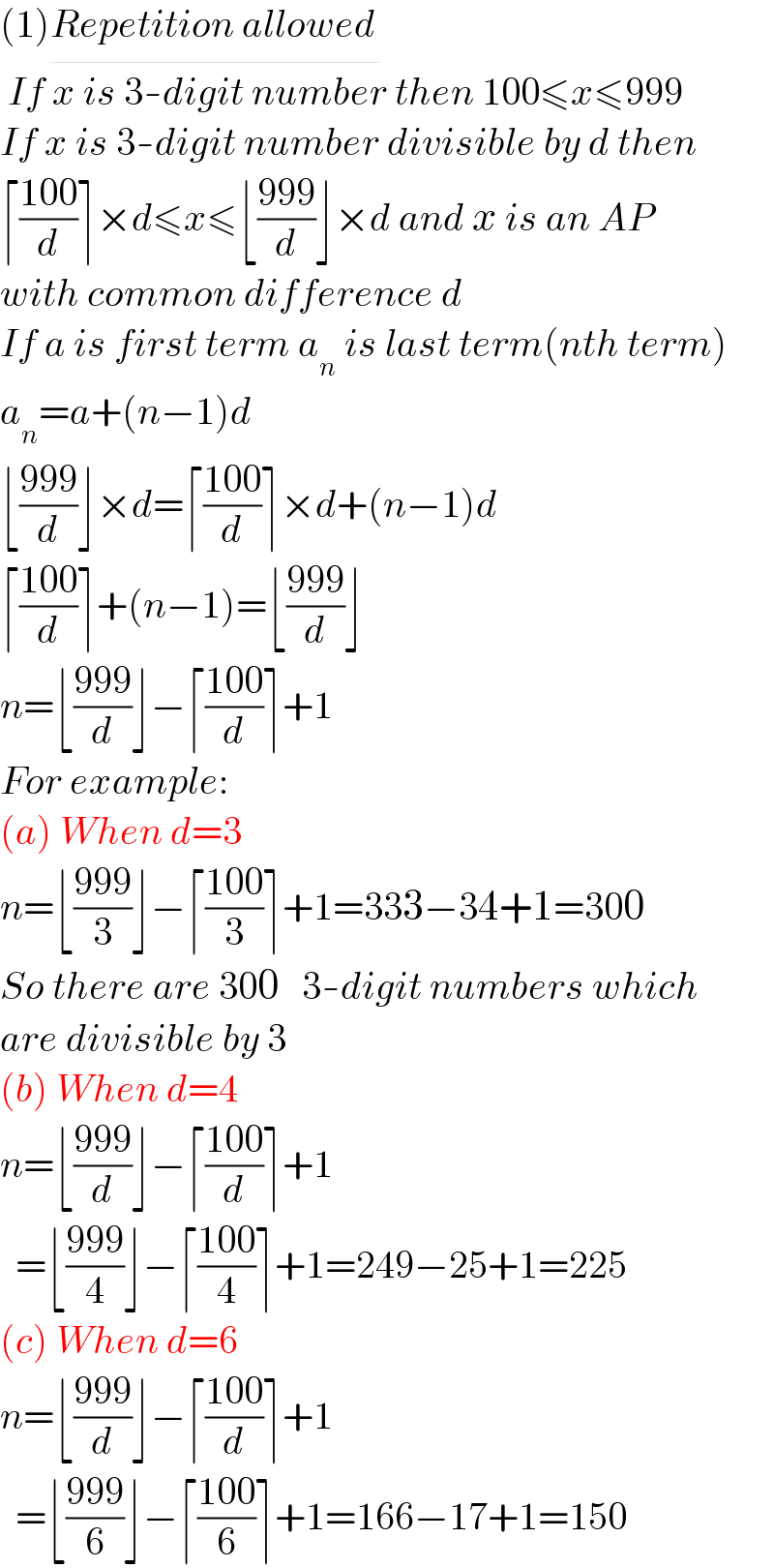
Question Number 197564 by SLVR last updated on 21/Sep/23

s i r \dots n u m b e r o f 3 d i g i t

n u m b e r s w h i c h a r e d i v i s i b l e

b y

a) 3 b) 4 c) 6 d) 7 e) 8 f) 9 g) 11

w h e n r e p e t e t i o n i s

1) A l l o w w d 2) N o t a l l o w e d . .

k i n d l y h e l p m e s i r

Commented by Tinku Tara last updated on 21/Sep/23

All numbers are divisble by one .

check your question

Commented by SLVR last updated on 21/Sep/23

i a m s o r r y b y 1 w a s w r o g l y t y p e d

Commented by JDamian last updated on 22/Sep/23

I n t h e c a s e 1),

[A] t h e 3 - d i g i t n u m b e r s s p a n

100 t o 999 .

[B] t h e n u m b e r s w h i c h a r e d i v i s i b l e

b y \boldsymbol n a p p e a r s e v e r y \boldsymbol n p o s i t i o n s .

W i t h t h e s e h i n t s I p r e s u m e y o u a r e

s m a r t e n o u g h t o c a l c u l a t e t h e v a l u e s

y o u a s k e d f o r .

Commented by mr W last updated on 24/Sep/23

2) r e p e t i t i o n n o t a l l o w e d

a) d i v i s i b l e b y 3

\Rightarrow s e e e x a m p l e i n Q 197589

n u m b e r s o f t y p e p q r : 30 \times 3! = 180

n u m b e r s o f t y p e p 0 q : 2 \times (3 + 3 \times 3) \times 2! = 48

t o t a l l y : 180 + 48 = 228 ✓

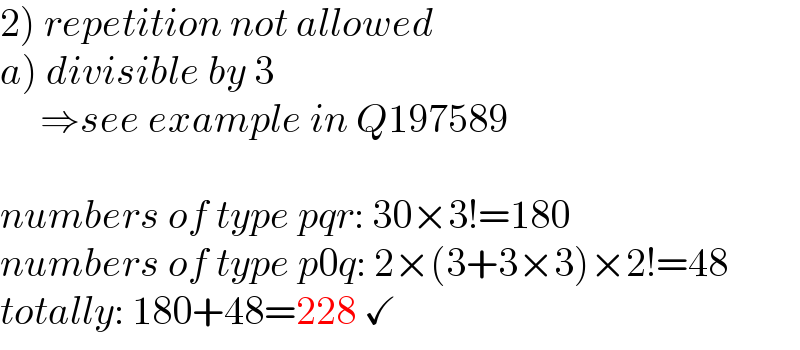
Answered by Rasheed.Sindhi last updated on 22/Sep/23

(1) \underset{―}{R e p e t i t i o n {a l l o w e d}_{}}

I f x i s 3 - d i g i t n u m b e r t h e n 100 ⩽ x ⩽ 999

I f x i s 3 - d i g i t n u m b e r d i v i s i b l e b y d t h e n

⌈ \frac{100}{d} ⌉ \times d ⩽ x ⩽ ⌊ \frac{999}{d} ⌋ \times d a n d x i s a n A P

w i t h c o m m o n d i f f e r e n c e d

I f a i s f i r s t t e r m a_{n} i s l a s t t e r m (n t h t e r m)

a_{n} = a + (n - 1) d

⌊ \frac{999}{d} ⌋ \times d = ⌈ \frac{100}{d} ⌉ \times d + (n - 1) d

⌈ \frac{100}{d} ⌉ + (n - 1) = ⌊ \frac{999}{d} ⌋

n = ⌊ \frac{999}{d} ⌋ - ⌈ \frac{100}{d} ⌉ + 1

F o r e x a m p l e :

(a) W h e n d = 3

n = ⌊ \frac{999}{3} ⌋ - ⌈ \frac{100}{3} ⌉ + 1 = 333 - 34 + 1 = 300

S o t h e r e a r e 300 3 - d i g i t n u m b e r s w h i c h

a r e d i v i s i b l e b y 3

(b) W h e n d = 4

n = ⌊ \frac{999}{d} ⌋ - ⌈ \frac{100}{d} ⌉ + 1

= ⌊ \frac{999}{4} ⌋ - ⌈ \frac{100}{4} ⌉ + 1 = 249 - 25 + 1 = 225

(c) W h e n d = 6

n = ⌊ \frac{999}{d} ⌋ - ⌈ \frac{100}{d} ⌉ + 1

= ⌊ \frac{999}{6} ⌋ - ⌈ \frac{100}{6} ⌉ + 1 = 166 - 17 + 1 = 150