Find-the-number-of-integers-greater-than-6200-that-can-be-formed-from-the-digits-1-3-6-8-and-9-where-each-digit-is-used-at-most-once-

Question Number 199333 by necx122 last updated on 01/Nov/23

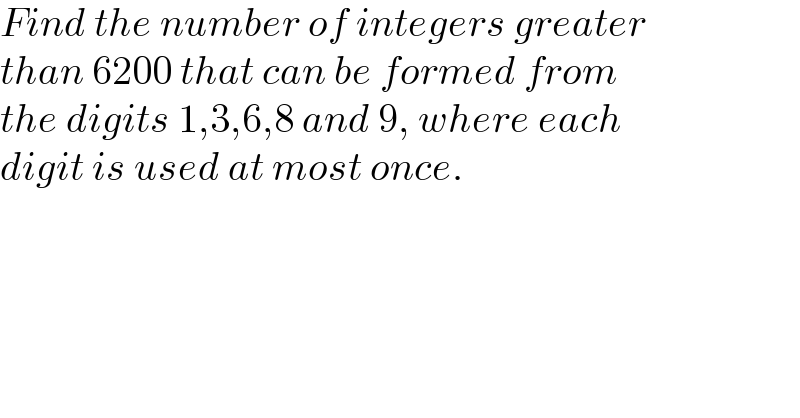
F i n d t h e n u m b e r o f i n t e g e r s g r e a t e r

t h a n 6200 t h a t c a n b e f o r m e d f r o m

t h e d i g i t s 1, 3, 6, 8 a n d 9, w h e r e e a c h

d i g i t i s u s e d a t m o s t o n c e .

Answered by aleks041103 last updated on 01/Nov/23

C a s e 1 : 4 - d i g i t n u m s

T h e f i r s t d i g i t c a n b e o n l y 6, 8 o r 9 .

C a s e 1.1 : 8 o r 9

w e h a v e 4 d i g i t s l e f t f o r 3 p o s i t i o n s .

\Rightarrow i n t o t a l = 2 \times (4 \times 3 \times 2) = 48

C a s e 1.2 : 6

t h e n u m b e r c a n b e o f t y p e s :

63 * *, 68 * *, 69 * *

f o r e a c h o f w h i c h w e h a v e 6 o p t i o n s

\Rightarrow i n t o t a l = 3 \times 6 = 18

C a s e 2 : 5 - d i g i t n u m s

A l l 5 - d i g i t a r e g r e a t e r t h n 6200 .

\Rightarrow i n t o t a l = 5 \times 4 \times 3 \times 2 \times 1 = 120

A n s w e r = 48 + 18 + 120

\Rightarrow A n s . = 186

Commented by necx122 last updated on 01/Nov/23

Thank you. This reminds me of how I learnt this those days with Mr. W's method. Thank you so much our old friend, Aleks.

Answered by mr W last updated on 01/Nov/23

1) 5 - d i g i t n u m b e r s :

5! = 120

2) v a l i d 4 - d i g i t n u m b e r s :

63 x y, 68 x y, 69 x y,

81 x y, 83 x y, 86 x y, 89 x y,

91 x y, 93 x y, 96 x y, 98 x y

x y = 2 f r o m 3 = P_{2}^{3}

\Rightarrow 11 \times P_{2}^{3} = 66

t o t a l l y : 120 + 66 = 186 ✓

Commented by necx122 last updated on 01/Nov/23

always on it sir. Thank you. This is clear and well understood.