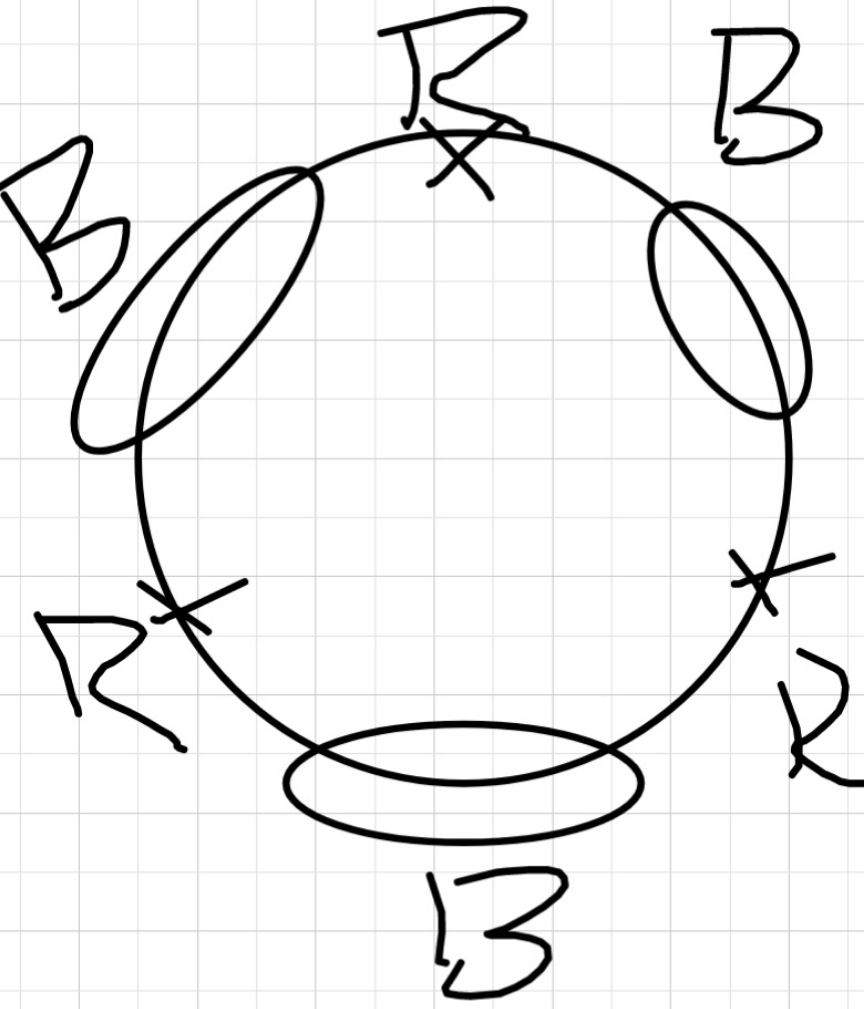
Question Number 200051 by cortano12 last updated on 13/Nov/23

Commented by mr W last updated on 13/Nov/23

Commented by cortano12 last updated on 13/Nov/23

Answered by mr W last updated on 13/Nov/23

Commented by cortano12 last updated on 13/Nov/23
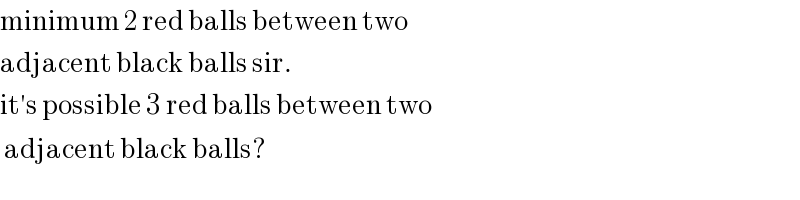
Commented by mr W last updated on 13/Nov/23

Commented by cortano12 last updated on 13/Nov/23

Commented by cortano12 last updated on 13/Nov/23
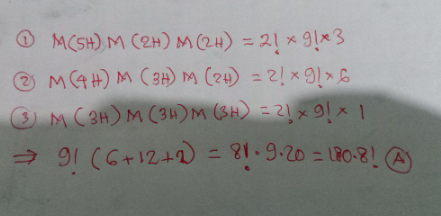
Commented by mr W last updated on 13/Nov/23
Answered by MM42 last updated on 13/Nov/23
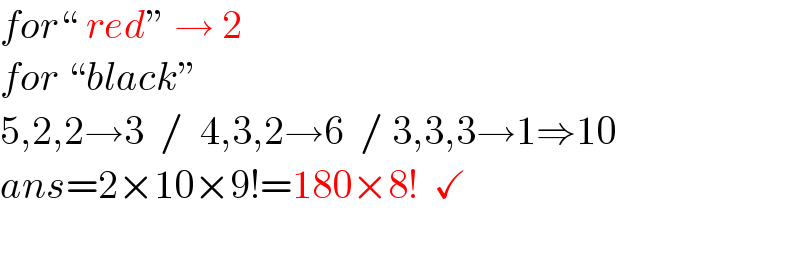
Commented by MM42 last updated on 13/Nov/23