Question-201848

Question Number 201848 by cortano12 last updated on 14/Dec/23

Answered by dimentri last updated on 14/Dec/23

1820 = 2^2 ×5×7×13 the number of positive factors from 1820 = Σ_(i=0) ^5 ((5),(i) ) = 2^5 = 32

$$\:\:\mathrm{1820}\:=\:\mathrm{2}^{\mathrm{2}} ×\mathrm{5}×\mathrm{7}×\mathrm{13} \\ $$$$\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{positive}\:\mathrm{factors} \\ $$$$\:\mathrm{from}\:\mathrm{1820}\:=\:\underset{\mathrm{i}=\mathrm{0}} {\overset{\mathrm{5}} {\sum}}\begin{pmatrix}{\mathrm{5}}\\{\mathrm{i}}\end{pmatrix}\:=\:\mathrm{2}^{\mathrm{5}} =\:\mathrm{32} \\ $$

Answered by mr W last updated on 14/Dec/23

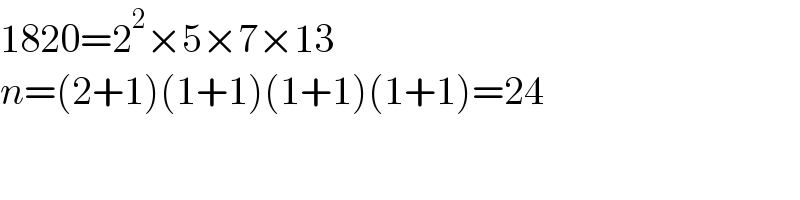
1820=2^2 ×5×7×13 n=(2+1)(1+1)(1+1)(1+1)=24

$$\mathrm{1820}=\mathrm{2}^{\mathrm{2}} ×\mathrm{5}×\mathrm{7}×\mathrm{13} \\ $$$${n}=\left(\mathrm{2}+\mathrm{1}\right)\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{1}+\mathrm{1}\right)=\mathrm{24} \\ $$

Commented by cortano12 last updated on 14/Dec/23

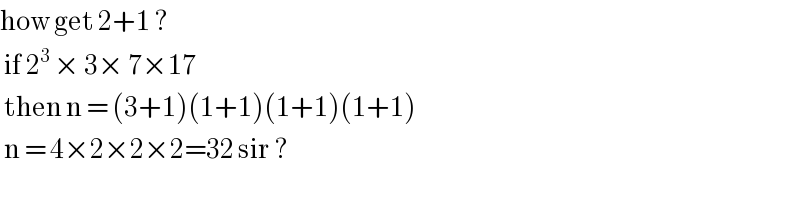
how get 2+1 ? if 2^3 × 3× 7×17 then n = (3+1)(1+1)(1+1)(1+1) n = 4×2×2×2=32 sir ?

$$\mathrm{how}\:\mathrm{get}\:\mathrm{2}+\mathrm{1}\:?\: \\ $$$$\:\mathrm{if}\:\mathrm{2}^{\mathrm{3}} \:×\:\mathrm{3}×\:\mathrm{7}×\mathrm{17}\: \\ $$$$\:\mathrm{then}\:\mathrm{n}\:=\:\left(\mathrm{3}+\mathrm{1}\right)\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{1}+\mathrm{1}\right) \\ $$$$\:\mathrm{n}\:=\:\mathrm{4}×\mathrm{2}×\mathrm{2}×\mathrm{2}=\mathrm{32}\:\mathrm{sir}\:? \\ $$

Commented by mr W last updated on 14/Dec/23

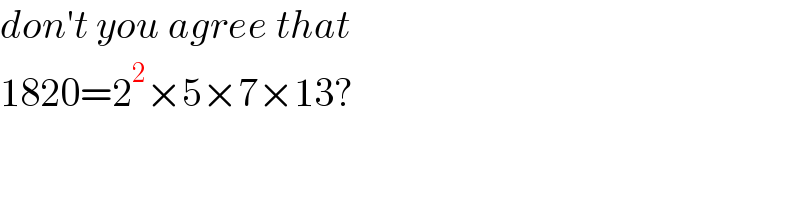
$${don}'{t}\:{you}\:{agree}\:{that} \\ $$$$\mathrm{1820}=\mathrm{2}^{\mathrm{2}} ×\mathrm{5}×\mathrm{7}×\mathrm{13}? \\ $$

Commented by cortano12 last updated on 14/Dec/23

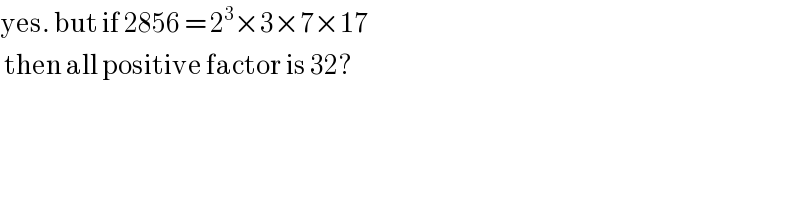
yes. but if 2856 = 2^3 ×3×7×17 then all positive factor is 32?

$$\mathrm{yes}.\:\mathrm{but}\:\mathrm{if}\:\mathrm{2856}\:=\:\mathrm{2}^{\mathrm{3}} ×\mathrm{3}×\mathrm{7}×\mathrm{17} \\ $$$$\:\mathrm{then}\:\mathrm{all}\:\mathrm{positive}\:\mathrm{factor}\:\mathrm{is}\:\mathrm{32}? \\ $$

Commented by cortano12 last updated on 14/Dec/23

$$\mathrm{what}\:\mathrm{this}\:\mathrm{formula}? \\ $$

Commented by mr W last updated on 14/Dec/23

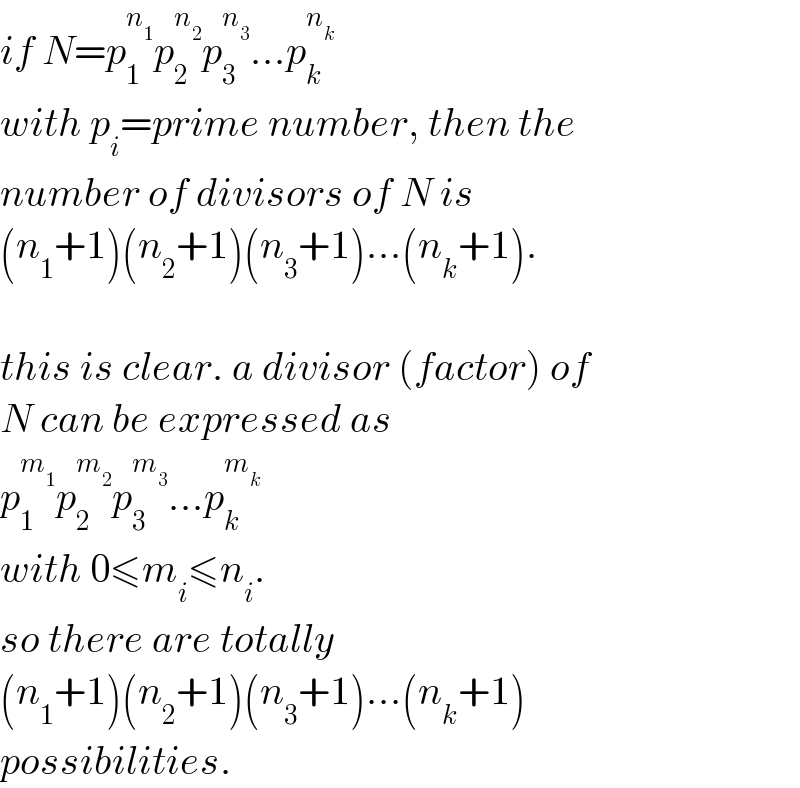
if N=p_1 ^n_1 p_2 ^n_2 p_3 ^n_3 ...p_k ^n_k with p_i =prime number, then the number of divisors of N is (n_1 +1)(n_2 +1)(n_3 +1)...(n_k +1). this is clear. a divisor (factor) of N can be expressed as p_1 ^m_1 p_2 ^m_2 p_3 ^m_3 ...p_k ^m_k with 0≤m_i ≤n_i . so there are totally (n_1 +1)(n_2 +1)(n_3 +1)...(n_k +1) possibilities.

$${if}\:{N}={p}_{\mathrm{1}} ^{{n}_{\mathrm{1}} } {p}_{\mathrm{2}} ^{{n}_{\mathrm{2}} } {p}_{\mathrm{3}} ^{{n}_{\mathrm{3}} } …{p}_{{k}} ^{{n}_{{k}} } \\ $$$${with}\:{p}_{{i}} ={prime}\:{number},\:{then}\:{the} \\ $$$${number}\:{of}\:{divisors}\:{of}\:{N}\:{is} \\ $$$$\left({n}_{\mathrm{1}} +\mathrm{1}\right)\left({n}_{\mathrm{2}} +\mathrm{1}\right)\left({n}_{\mathrm{3}} +\mathrm{1}\right)…\left({n}_{{k}} +\mathrm{1}\right). \\ $$$$ \\ $$$${this}\:{is}\:{clear}.\:{a}\:{divisor}\:\left({factor}\right)\:{of} \\ $$$${N}\:{can}\:{be}\:{expressed}\:{as} \\ $$$${p}_{\mathrm{1}} ^{{m}_{\mathrm{1}} } {p}_{\mathrm{2}} ^{{m}_{\mathrm{2}} } {p}_{\mathrm{3}} ^{{m}_{\mathrm{3}} } …{p}_{{k}} ^{{m}_{{k}} } \\ $$$${with}\:\mathrm{0}\leqslant{m}_{{i}} \leqslant{n}_{{i}} . \\ $$$${so}\:{there}\:{are}\:{totally} \\ $$$$\left({n}_{\mathrm{1}} +\mathrm{1}\right)\left({n}_{\mathrm{2}} +\mathrm{1}\right)\left({n}_{\mathrm{3}} +\mathrm{1}\right)…\left({n}_{{k}} +\mathrm{1}\right) \\ $$$${possibilities}. \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply