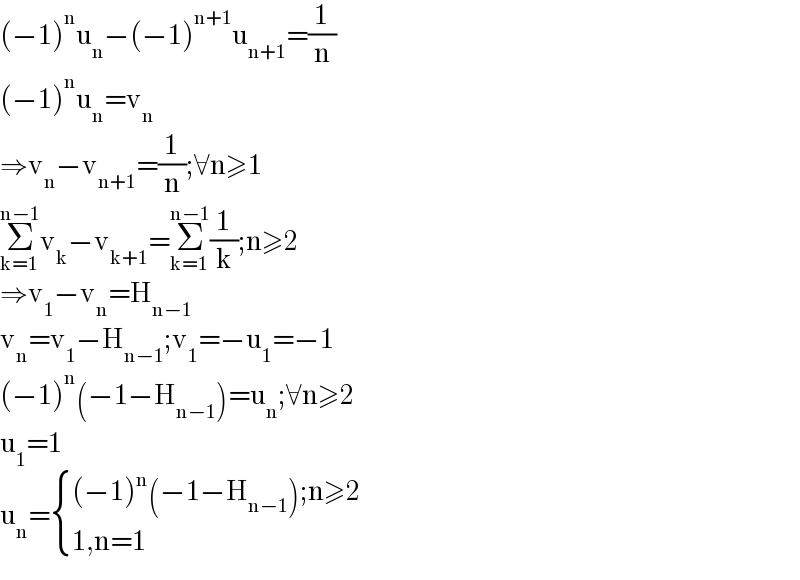find-the-seauence-u-n-wich-verify-u-0-1-and-u-n-u-n-1-1-n-n-for-n-1-

Question Number 202865 by Mathspace last updated on 04/Jan/24

f i n d t h e s e a u e n c e u_{n} w i c h v e r i f y

u_{0} = 1 a n d u_{n} + u_{n + 1} = \frac{{(- 1)}^{n}}{n}

f o r n ⩾ 1

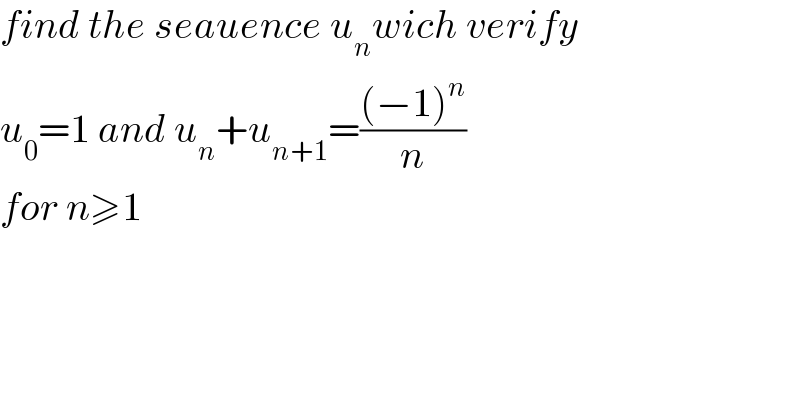
Commented by mr W last updated on 05/Jan/24

y o u m e a n u_{1} = 1 ?

Answered by witcher3 last updated on 04/Jan/24

not defined

may bee u_{n} + u_{n + 1} = \frac{{(- 1)}^{n + 1}}{n + 1}

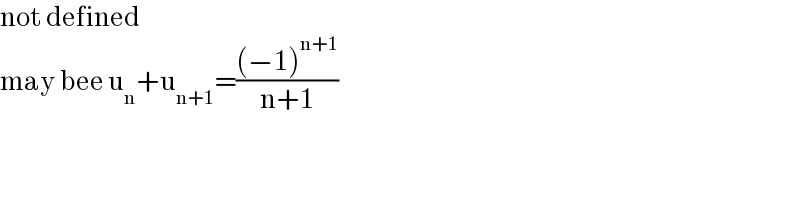
Commented by Mathspace last updated on 05/Jan/24

n o s i r t h i s s e q u e n c e i s d e f i n e d \dots

Answered by Mathspace last updated on 05/Jan/24

t a k e u_{1} = 1

Answered by witcher3 last updated on 05/Jan/24

{(- 1)}^{n} u_{n} - {(- 1)}^{n + 1} u_{n + 1} = \frac{1}{n}

{(- 1)}^{n} u_{n} = v_{n}

\Rightarrow v_{n} - v_{n + 1} = \frac{1}{n}; \forall n ⩾ 1

\underset{k = 1}{\sum^{n - 1}} v_{k} - v_{k + 1} = \underset{k = 1}{\sum^{n - 1}} \frac{1}{k}; n ⩾ 2

\Rightarrow v_{1} - v_{n} = H_{n - 1}

v_{n} = v_{1} - H_{n - 1}; v_{1} = - u_{1} = - 1

{(- 1)}^{n} (- 1 - H_{n - 1}) = u_{n}; \forall n ⩾ 2

u_{1} = 1

u_{n} = {\begin{cases} {(- 1)}^{n} (- 1 - H_{n - 1}); n ⩾ 2 \\ 1, n = 1 \end{cases}

Facebook Tweet Pin