Question Number 205627 by lmcp1203 last updated on 25/Mar/24

Answered by Rasheed.Sindhi last updated on 27/Mar/24
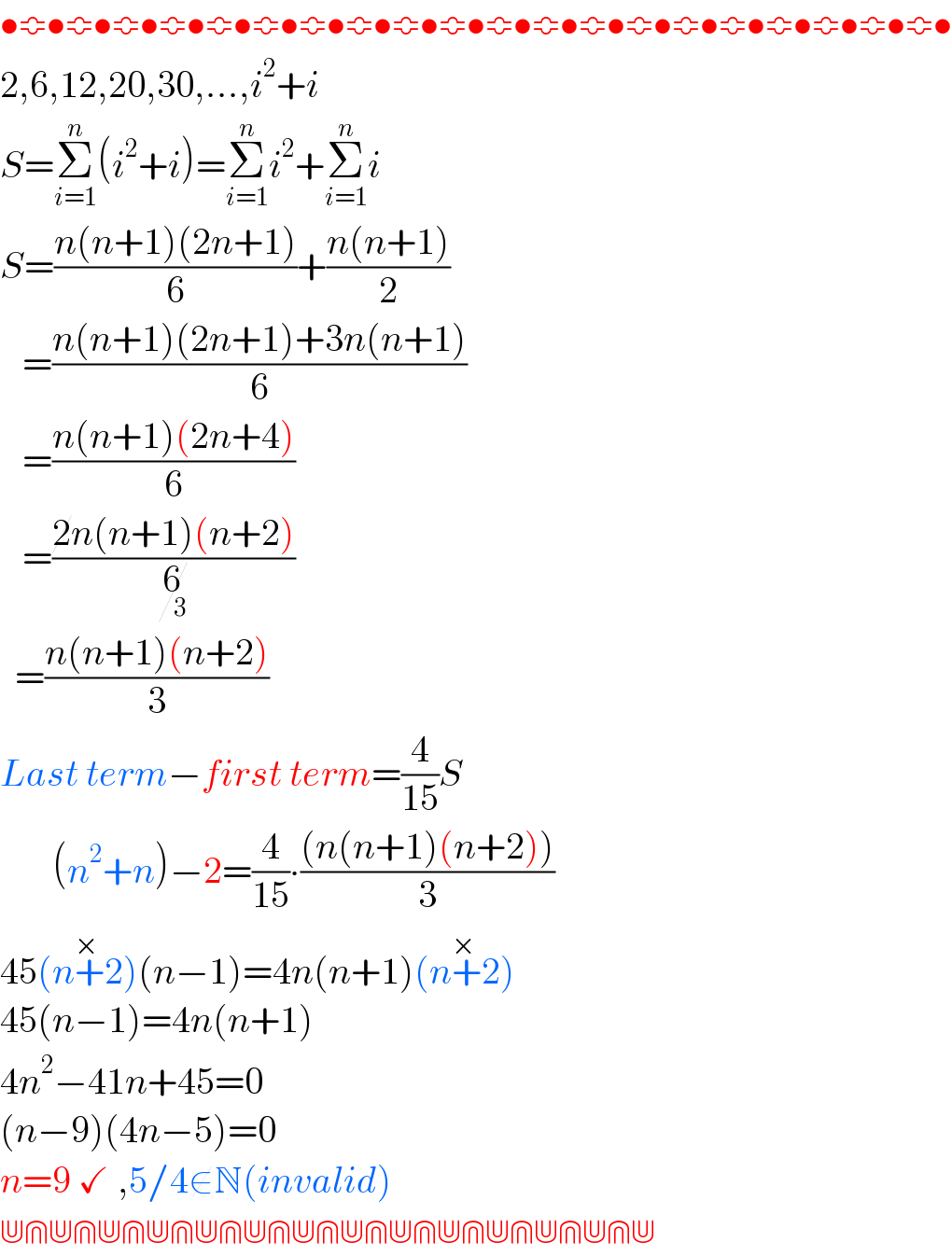
$$\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet\Bumpeq\bullet \\ $$$$\mathrm{2},\mathrm{6},\mathrm{12},\mathrm{20},\mathrm{30},…,{i}^{\mathrm{2}} +{i} \\ $$$${S}=\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}\left({i}^{\mathrm{2}} +{i}\right)=\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{i}^{\mathrm{2}} +\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{i} \\ $$$${S}=\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)}{\mathrm{6}}+\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}} \\ $$$$\:\:\:=\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)+\mathrm{3}{n}\left({n}+\mathrm{1}\right)}{\mathrm{6}} \\ $$$$\:\:\:=\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{4}\right)}{\mathrm{6}} \\ $$$$\:\:\:=\frac{\cancel{\mathrm{2}}{n}\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right)}{\cancel{\underset{\:\:\:\:\mathrm{3}} {\mathrm{6}}}} \\ $$$$\:\:=\frac{{n}\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right)}{\mathrm{3}} \\ $$$${Last}\:{term}−{first}\:{term}=\frac{\mathrm{4}}{\mathrm{15}}{S} \\ $$$$\:\:\:\:\:\:\:\left({n}^{\mathrm{2}} +{n}\right)−\mathrm{2}=\frac{\mathrm{4}}{\mathrm{15}}\centerdot\frac{\left({n}\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right)\right)}{\mathrm{3}} \\ $$$$\mathrm{45}\overset{×} {\left({n}+\mathrm{2}\right)}\left({n}−\mathrm{1}\right)=\mathrm{4}{n}\left({n}+\mathrm{1}\right)\overset{×} {\left({n}+\mathrm{2}\right)} \\ $$$$\mathrm{45}\left({n}−\mathrm{1}\right)=\mathrm{4}{n}\left({n}+\mathrm{1}\right) \\ $$$$\mathrm{4}{n}^{\mathrm{2}} −\mathrm{41}{n}+\mathrm{45}=\mathrm{0} \\ $$$$\left({n}−\mathrm{9}\right)\left(\mathrm{4}{n}−\mathrm{5}\right)=\mathrm{0} \\ $$$${n}=\mathrm{9}\:\checkmark\:,\mathrm{5}/\mathrm{4}\notin\mathbb{N}\left({invalid}\right) \\ $$$$\Cup\Cap\Cup\Cap\Cup\Cap\Cup\Cap\Cup\Cap\Cup\Cap\Cup\Cap\Cup\Cap\Cup\Cap\Cup\Cap\Cup\Cap\Cup\Cap\Cup\Cap\Cup\: \\ $$
Commented by lmcp1203 last updated on 27/Mar/24

$${thanks} \\ $$
