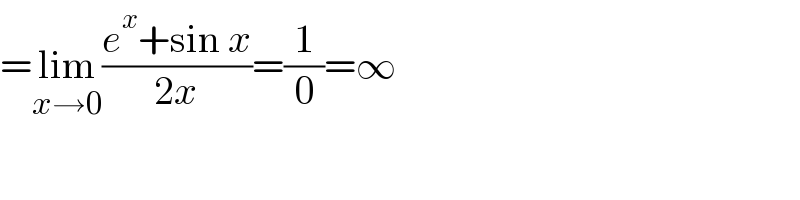calculate-lim-x-0-e-x-cosx-x-2-

Question Number 205727 by mathzup last updated on 28/Mar/24

c a l c u l a t e {l i m}_{x \to 0} \frac{e^{x} - c o s x}{x^{2}}

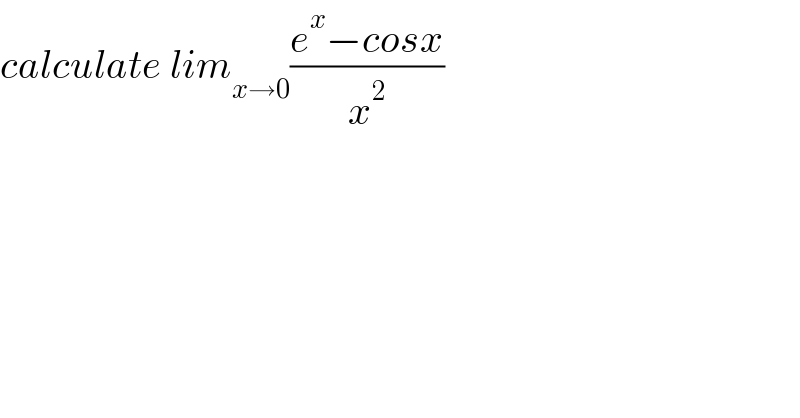
Commented by lepuissantcedricjunior last updated on 29/Mar/24

\lim_{x \to 0} \frac{e^{x} - c o s x}{x^{2}} = \frac{0}{0} = FI

\Leftrightarrow \lim_{x \to 0} \frac{e^{x} - 1 - (c o s x - 1)}{x^{2}}

\Leftrightarrow \lim_{x \to 0} (\frac{e^{x} - 1}{x^{2}} - \frac{c o s x - 1}{x^{2}})

d l e^{x} = 1 + x + \frac{x^{2}}{2!} + \frac{x^{3}}{3!} + x^{3} (0)

d l c o s (x) = 1 - \frac{x^{2}}{2!} + \frac{x^{4}}{4!} + x^{4} (0)

\lim_{x \to 0} \frac{1 + x + \frac{x^{2}}{2} + \frac{x^{3}}{3!} - 1 - 1 + \frac{x^{2}}{2} - \frac{x^{4}}{4} + 1}{x^{2}}

\Leftrightarrow \lim_{x \to 0} \frac{1 + x + \frac{x^{2}}{3!} - \frac{x^{3}}{4!}}{x} = \frac{1}{0} = \infty

\lim_{x \to 0} \frac{e^{x} - c o s x}{x^{2}} = \infty

\dots \dots \dots \dots . l e p u i s s a n t D r \dots \dots \dots \dots \dots \dots \dots .

Commented by mr W last updated on 28/Mar/24

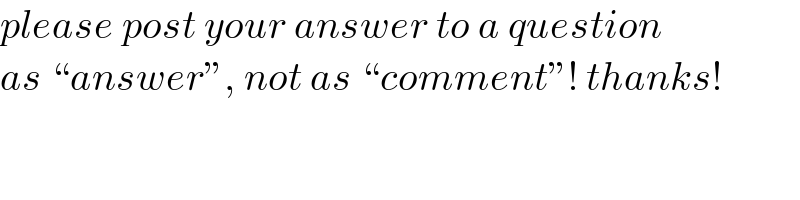
p l e a s e p o s t y o u r a n s w e r t o a q u e s t i o n

a s “ {a n s w e r}^{″}, n o t a s “ {c o m m e n t}^{″}! t h a n k s!

Commented by mr W last updated on 28/Mar/24

veuillez poster votre réponse à une question comme "réponse", pas comme "commentaire"! Merci!

Commented by York12 last updated on 29/Mar/24

Tu es Francis!?

Answered by mr W last updated on 28/Mar/24

= \lim_{x \to 0} \frac{e^{x} + \sin x}{2 x} = \frac{1}{0} = \infty

Facebook Tweet Pin