Question Number 206198 by lmcp1203 last updated on 09/Apr/24
Answered by A5T last updated on 09/Apr/24

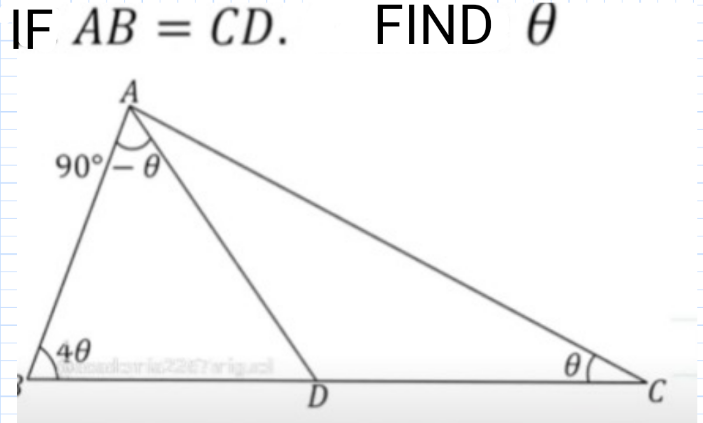
$$\frac{{sin}\mathrm{4}\theta}{{AD}}=\frac{{sin}\left(\mathrm{90}−\mathrm{3}\theta\right)}{{AB}};\frac{{sin}\left(\theta\right)}{{AD}}=\frac{{sin}\left(\mathrm{90}−\mathrm{4}\theta\right)}{{CD}={AB}} \\ $$$$\Rightarrow\frac{{sin}\left(\mathrm{4}\theta\right)}{{sin}\left(\mathrm{90}−\mathrm{3}\theta\right)}=\frac{{sin}\left(\theta\right)}{{sin}\left(\mathrm{90}−\mathrm{4}\theta\right)}\Rightarrow{x}=\mathrm{20}° \\ $$
Answered by lmcp1203 last updated on 09/Apr/24

$${thanks}. \\ $$
