Question-208913

Question Number 208913 by efronzo1 last updated on 27/Jun/24

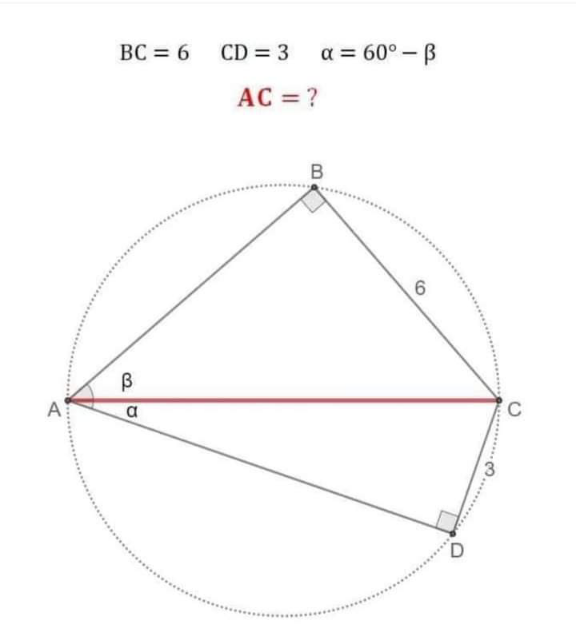
Answered by A5T last updated on 27/Jun/24

\frac{s i n β}{6} = \frac{1}{A C}; \frac{s i n (60 - β)}{3} = \frac{1}{A C}

\Rightarrow \frac{s i n (60 - β)}{s i n β} = \frac{1}{2} \Rightarrow β \approx 40.8934

\Rightarrow s i n β \approx 0.654654; w e c a n t h e n f i n d A C = \frac{6}{s i n β}

A C \approx 9.165151

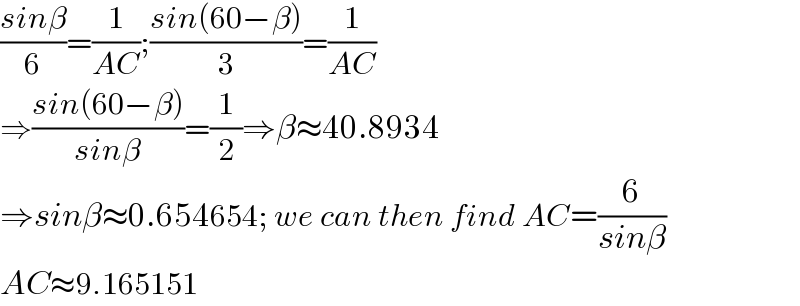
Answered by mr W last updated on 27/Jun/24

∠ B C D = 90 ° - α + 90 ° - β = 120 °

{B D}^{2} = 6^{2} + 3^{2} - 2 \times 3 \times 6 \cos 120 ° = 63

A C = 2 R = \frac{B D}{\sin ∠ B C D} = \frac{\sqrt{63}}{\sin 120 °}

= 2 \sqrt{21} \approx 9.165

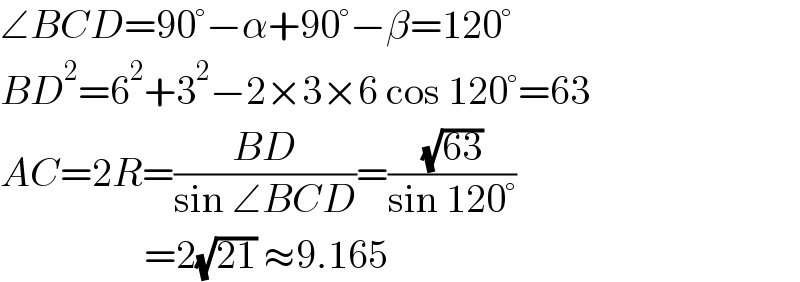
Answered by mr W last updated on 27/Jun/24

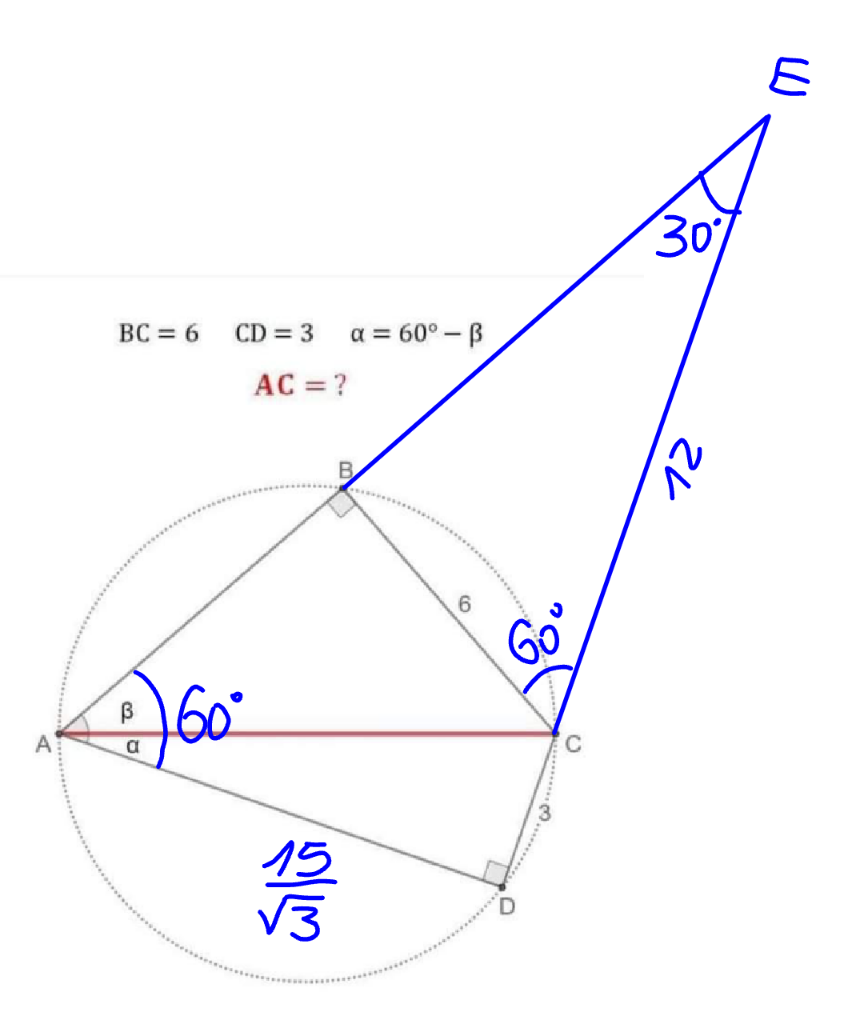
Commented by mr W last updated on 27/Jun/24

C E = 2 \times 6 = 12

D E = 12 + 3 = 15

A D = \frac{15}{\sqrt{3}} = 5 \sqrt{3}

A C = \sqrt{{(5 \sqrt{3})}^{2} + 3^{2}} = 2 \sqrt{21} \approx 9.165

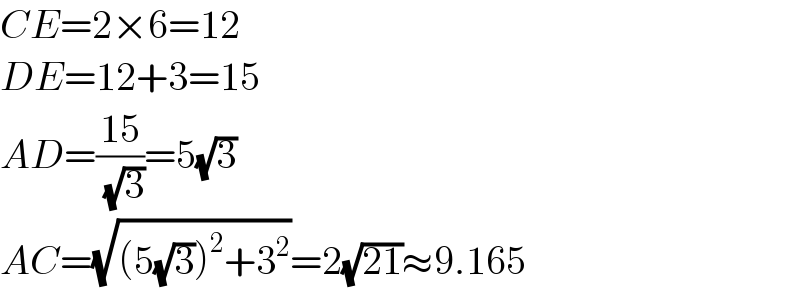
Commented by Tawa11 last updated on 27/Jun/24

weldone sirs

Facebook Tweet Pin