Question Number 79352 by M±th+et£s last updated on 24/Jan/20

$$\int\frac{{dx}}{\mathrm{1}−\sqrt{{cos}\left({x}\right)}} \\ $$
Commented by mr W last updated on 24/Jan/20

$${no}\:{solution}\:{with}\:{elementary}\:{functions}. \\ $$$${or}\:{do}\:{you}\:{know}\:{a}\:{solution}? \\ $$
Commented by M±th+et£s last updated on 24/Jan/20
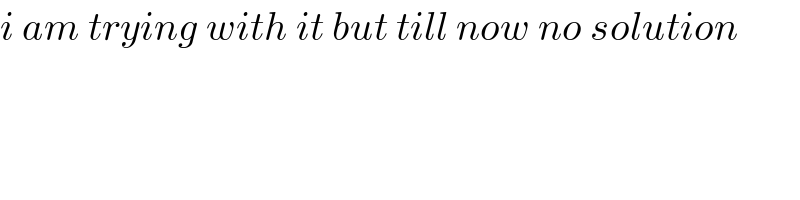
$${i}\:{am}\:{trying}\:{with}\:{it}\:{but}\:{till}\:{now}\:{no}\:{solution} \\ $$
Answered by mind is power last updated on 24/Jan/20
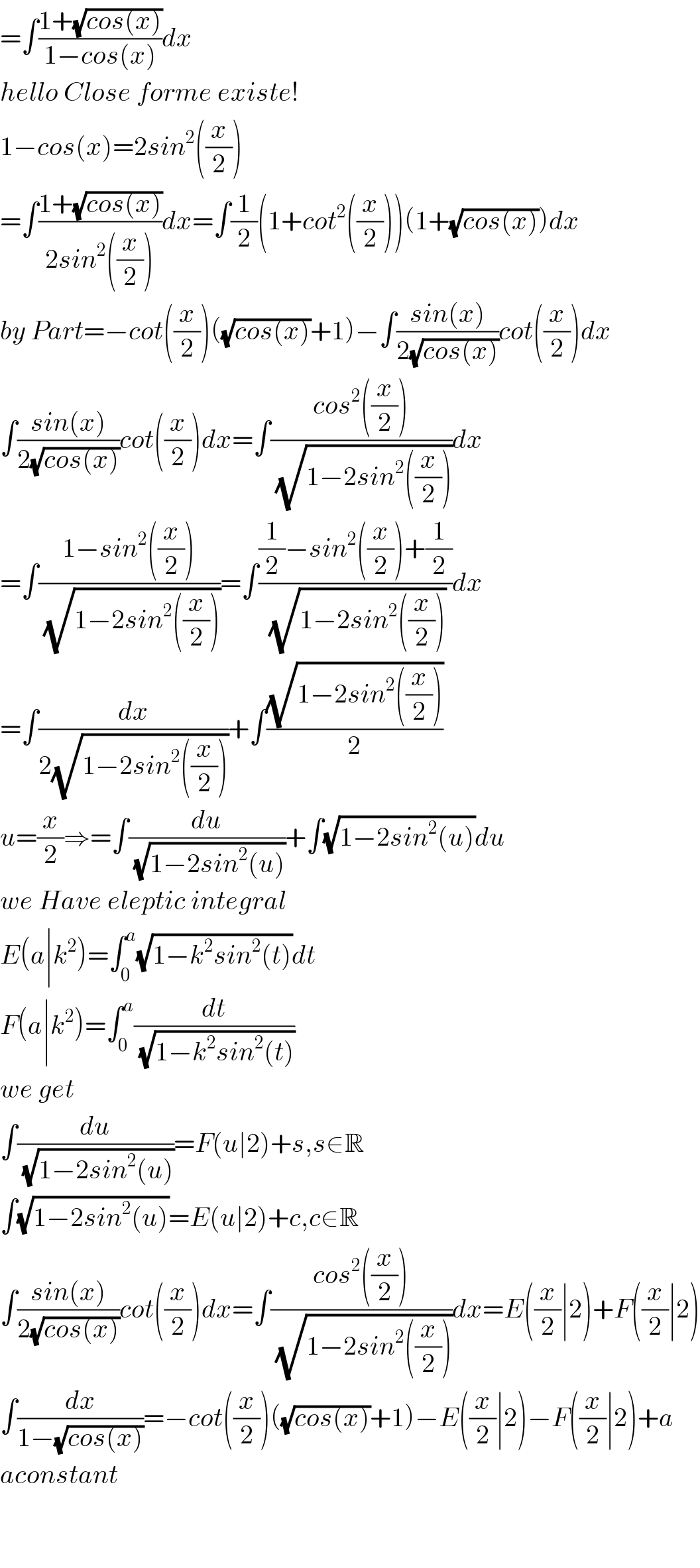
$$=\int\frac{\mathrm{1}+\sqrt{{cos}\left({x}\right)}}{\mathrm{1}−{cos}\left({x}\right)}{dx} \\ $$$${hello}\:{Close}\:{forme}\:{existe}! \\ $$$$\mathrm{1}−{cos}\left({x}\right)=\mathrm{2}{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right) \\ $$$$=\int\frac{\mathrm{1}+\sqrt{{cos}\left({x}\right)}}{\mathrm{2}{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}{dx}=\int\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}+{cot}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)\right)\left(\mathrm{1}+\sqrt{{cos}\left({x}\right)}\right){dx} \\ $$$${by}\:{Part}=−{cot}\left(\frac{{x}}{\mathrm{2}}\right)\left(\sqrt{{cos}\left({x}\right)}+\mathrm{1}\right)−\int\frac{{sin}\left({x}\right)}{\mathrm{2}\sqrt{{cos}\left({x}\right)}}{cot}\left(\frac{{x}}{\mathrm{2}}\right){dx} \\ $$$$\int\frac{{sin}\left({x}\right)}{\mathrm{2}\sqrt{{cos}\left({x}\right)}}{cot}\left(\frac{{x}}{\mathrm{2}}\right){dx}=\int\frac{{cos}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}{\:\sqrt{\mathrm{1}−\mathrm{2}{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}}{dx} \\ $$$$=\int\frac{\mathrm{1}−{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}{\:\sqrt{\mathrm{1}−\mathrm{2}{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}}=\int\frac{\frac{\mathrm{1}}{\mathrm{2}}−{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)+\frac{\mathrm{1}}{\mathrm{2}}}{\:\sqrt{\mathrm{1}−\mathrm{2}{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}}{dx} \\ $$$$=\int\frac{{dx}}{\mathrm{2}\sqrt{\mathrm{1}−\mathrm{2}{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}}+\int\frac{\sqrt{\mathrm{1}−\mathrm{2}{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}}{\mathrm{2}} \\ $$$${u}=\frac{{x}}{\mathrm{2}}\Rightarrow=\int\frac{{du}}{\:\sqrt{\mathrm{1}−\mathrm{2}{sin}^{\mathrm{2}} \left({u}\right)}}+\int\sqrt{\mathrm{1}−\mathrm{2}{sin}^{\mathrm{2}} \left({u}\right)}{du} \\ $$$${we}\:{Have}\:{eleptic}\:{integral} \\ $$$${E}\left({a}\mid{k}^{\mathrm{2}} \right)=\int_{\mathrm{0}} ^{{a}} \sqrt{\mathrm{1}−{k}^{\mathrm{2}} {sin}^{\mathrm{2}} \left({t}\right)}{dt} \\ $$$${F}\left({a}\mid{k}^{\mathrm{2}} \right)=\int_{\mathrm{0}} ^{{a}} \frac{{dt}}{\:\sqrt{\mathrm{1}−{k}^{\mathrm{2}} {sin}^{\mathrm{2}} \left({t}\right)}} \\ $$$${we}\:{get} \\ $$$$\int\frac{{du}}{\:\sqrt{\mathrm{1}−\mathrm{2}{sin}^{\mathrm{2}} \left({u}\right)}}={F}\left({u}\mid\mathrm{2}\right)+{s},{s}\in\mathbb{R} \\ $$$$\int\sqrt{\mathrm{1}−\mathrm{2}{sin}^{\mathrm{2}} \left({u}\right)}={E}\left({u}\mid\mathrm{2}\right)+{c},{c}\in\mathbb{R} \\ $$$$\int\frac{{sin}\left({x}\right)}{\mathrm{2}\sqrt{{cos}\left({x}\right)}}{cot}\left(\frac{{x}}{\mathrm{2}}\right){dx}=\int\frac{{cos}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}{\:\sqrt{\mathrm{1}−\mathrm{2}{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}}{dx}={E}\left(\frac{{x}}{\mathrm{2}}\mid\mathrm{2}\right)+{F}\left(\frac{{x}}{\mathrm{2}}\mid\mathrm{2}\right) \\ $$$$\int\frac{{dx}}{\mathrm{1}−\sqrt{{cos}\left({x}\right)}}=−{cot}\left(\frac{{x}}{\mathrm{2}}\right)\left(\sqrt{{cos}\left({x}\right)}+\mathrm{1}\right)−{E}\left(\frac{{x}}{\mathrm{2}}\mid\mathrm{2}\right)−{F}\left(\frac{{x}}{\mathrm{2}}\mid\mathrm{2}\right)+{a} \\ $$$${aconstant} \\ $$$$ \\ $$$$ \\ $$
Commented by mr W last updated on 24/Jan/20
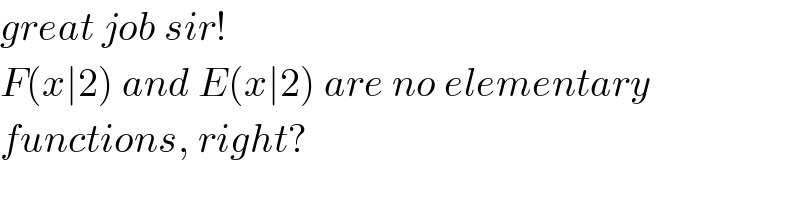
$${great}\:{job}\:{sir}! \\ $$$${F}\left({x}\mid\mathrm{2}\right)\:{and}\:{E}\left({x}\mid\mathrm{2}\right)\:{are}\:{no}\:{elementary} \\ $$$${functions},\:{right}? \\ $$
Commented by mind is power last updated on 24/Jan/20

$${non}\:{first}\:{and}\:\mathrm{2}{nd}\:{eleptic}\:{function}\:,{eleptic}\:{function} \\ $$$${they}\:{appear}\:{in}\:{bassel}\:{function}\:{as}\:{solution}\:{in}\:{physics}\:{in}\:{somm} \\ $$$${differential}\:{equation} \\ $$
