Menu Close

Let-us-call-complex-triangle-which-has-either-sides-or-angles-are-complex-numbers-Let-a-b-c-R-which-are-sides-of-a-complex-triangle-which-need-not-satisfy-triangle-inequality-say-a-1-b-2-and-c-4-P

Tinku Tara 0 Comments
Pin




Question Number 15759 by prakash jain last updated on 13/Jun/17
Let us call complex triangle which has either sides or angles are complex numbers. Let a,b,c ∈R which are sides of a complex triangle which need not satisfy triangle inequality. say a=1,b=2 and c=4. Prove (or counter example) (a/(sin A))=(b/(sin B))=(c/(sin C)) Is A+B+C=π? Assume only principle solution for A,B and C. For such a triangle A,B and C will take complex values.
$$\mathrm{Let}\:\mathrm{us}\:\mathrm{call}\:\mathrm{complex}\:\mathrm{triangle}\:\mathrm{which} \\ $$$$\mathrm{has}\:\mathrm{either}\:\mathrm{sides}\:\mathrm{or}\:\mathrm{angles}\:\mathrm{are} \\ $$$$\mathrm{complex}\:\mathrm{numbers}. \\ $$$$\mathrm{Let}\:{a},{b},{c}\:\in\mathbb{R}\:\mathrm{which}\:\mathrm{are}\:\mathrm{sides}\:\mathrm{of} \\ $$$$\mathrm{a}\:\mathrm{complex}\:\mathrm{triangle}\:\mathrm{which}\:\mathrm{need} \\ $$$$\mathrm{not}\:\mathrm{satisfy}\:\mathrm{triangle}\:\mathrm{inequality}. \\ $$$$\mathrm{say}\:{a}=\mathrm{1},{b}=\mathrm{2}\:\mathrm{and}\:{c}=\mathrm{4}. \\ $$$$\mathrm{Prove}\:\left(\mathrm{or}\:\mathrm{counter}\:\mathrm{example}\right) \\ $$$$\frac{{a}}{\mathrm{sin}\:{A}}=\frac{{b}}{\mathrm{sin}\:{B}}=\frac{{c}}{\mathrm{sin}\:{C}} \\ $$$$\mathrm{Is}\:{A}+{B}+{C}=\pi? \\ $$$$\mathrm{Assume}\:\mathrm{only}\:\mathrm{principle}\:\mathrm{solution} \\ $$$$\mathrm{for}\:{A},{B}\:\mathrm{and}\:{C}. \\ $$$$\mathrm{For}\:\mathrm{such}\:\mathrm{a}\:\mathrm{triangle}\:{A},{B}\:\mathrm{and}\:{C} \\ $$$$\mathrm{will}\:\mathrm{take}\:\mathrm{complex}\:\mathrm{values}. \\ $$
Answered by b.e.h.i.8.3.4.1.7@gmail.com last updated on 14/Jun/17
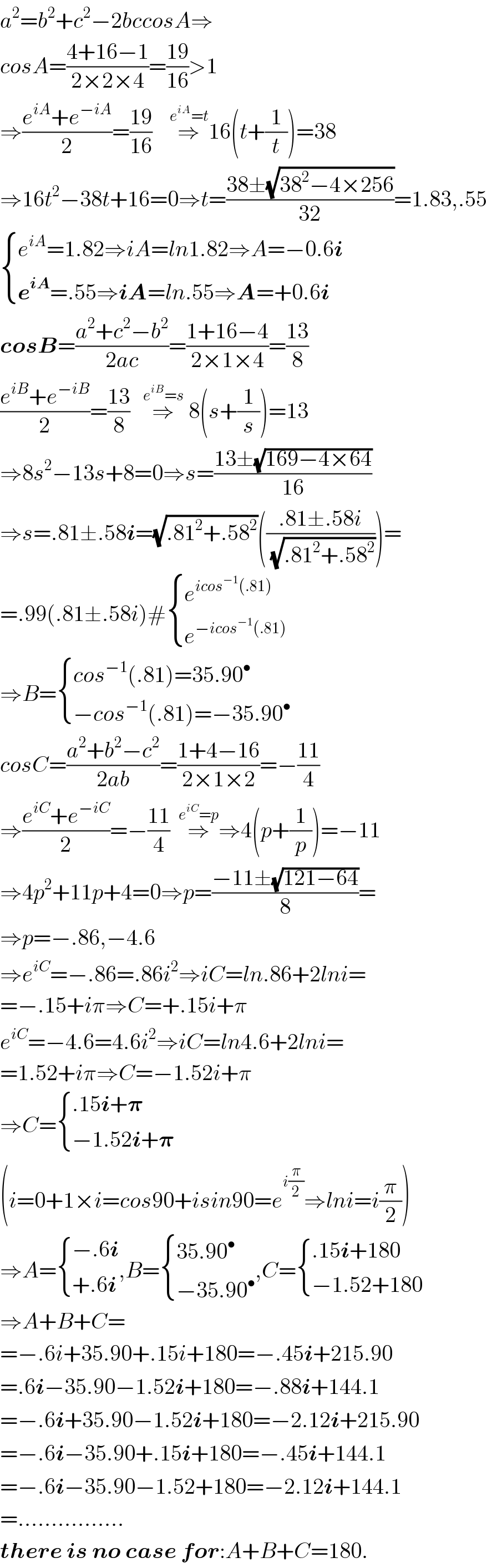
a^2 =b^2 +c^2 −2bccosA⇒ cosA=((4+16−1)/(2×2×4))=((19)/(16))>1 ⇒((e^(iA) +e^(−iA) )/2)=((19)/(16)) ⇒^(e^(iA) =t) 16(t+(1/t))=38 ⇒16t^2 −38t+16=0⇒t=((38±(√(38^2 −4×256)))/(32))=1.83,.55 { ((e^(iA) =1.82⇒iA=ln1.82⇒A=−0.6i)),((e^(iA) =.55⇒iA=ln.55⇒A=+0.6i)) :} cosB=((a^2 +c^2 −b^2 )/(2ac))=((1+16−4)/(2×1×4))=((13)/8) ((e^(iB) +e^(−iB) )/2)=((13)/8) ⇒^(e^(iB) =s) 8(s+(1/s))=13 ⇒8s^2 −13s+8=0⇒s=((13±(√(169−4×64)))/(16)) ⇒s=.81±.58i=(√(.81^2 +.58^2 ))(((.81±.58i)/( (√(.81^2 +.58^2 )))))= =.99(.81±.58i)# { (e^(icos^(−1) (.81)) ),(e^(−icos^(−1) (.81)) ) :} ⇒B= { ((cos^(−1) (.81)=35.90^• )),((−cos^(−1) (.81)=−35.90^• )) :} cosC=((a^2 +b^2 −c^2 )/(2ab))=((1+4−16)/(2×1×2))=−((11)/4) ⇒((e^(iC) +e^(−iC) )/2)=−((11)/4) ⇒^(e^(iC) =p) ⇒4(p+(1/p))=−11 ⇒4p^2 +11p+4=0⇒p=((−11±(√(121−64)))/8)= ⇒p=−.86,−4.6 ⇒e^(iC) =−.86=.86i^2 ⇒iC=ln.86+2lni= =−.15+iπ⇒C=+.15i+π e^(iC) =−4.6=4.6i^2 ⇒iC=ln4.6+2lni= =1.52+iπ⇒C=−1.52i+π ⇒C= { ((.15i+𝛑)),((−1.52i+𝛑)) :} (i=0+1×i=cos90+isin90=e^(i(π/2)) ⇒lni=i(π/2)) ⇒A= { ((−.6i)),((+.6i)) :},B= { ((35.90^• )),((−35.90^• )) :},C= { ((.15i+180)),((−1.52+180)) :} ⇒A+B+C= =−.6i+35.90+.15i+180=−.45i+215.90 =.6i−35.90−1.52i+180=−.88i+144.1 =−.6i+35.90−1.52i+180=−2.12i+215.90 =−.6i−35.90+.15i+180=−.45i+144.1 =−.6i−35.90−1.52+180=−2.12i+144.1 =................ there is no case for:A+B+C=180.
$${a}^{\mathrm{2}} ={b}^{\mathrm{2}} +{c}^{\mathrm{2}} −\mathrm{2}{bccosA}\Rightarrow \\ $$$${cosA}=\frac{\mathrm{4}+\mathrm{16}−\mathrm{1}}{\mathrm{2}×\mathrm{2}×\mathrm{4}}=\frac{\mathrm{19}}{\mathrm{16}}>\mathrm{1} \\ $$$$\Rightarrow\frac{{e}^{{iA}} +{e}^{−{iA}} }{\mathrm{2}}=\frac{\mathrm{19}}{\mathrm{16}}\:\:\:\:\overset{{e}^{{iA}} ={t}} {\Rightarrow}\mathrm{16}\left({t}+\frac{\mathrm{1}}{{t}}\right)=\mathrm{38} \\ $$$$\Rightarrow\mathrm{16}{t}^{\mathrm{2}} −\mathrm{38}{t}+\mathrm{16}=\mathrm{0}\Rightarrow{t}=\frac{\mathrm{38}\pm\sqrt{\mathrm{38}^{\mathrm{2}} −\mathrm{4}×\mathrm{256}}}{\mathrm{32}}=\mathrm{1}.\mathrm{83},.\mathrm{55} \\ $$$$\begin{cases}{{e}^{{iA}} =\mathrm{1}.\mathrm{82}\Rightarrow{iA}={ln}\mathrm{1}.\mathrm{82}\Rightarrow{A}=−\mathrm{0}.\mathrm{6}\boldsymbol{{i}}}\\{\boldsymbol{{e}}^{\boldsymbol{{iA}}} =.\mathrm{55}\Rightarrow\boldsymbol{{iA}}={ln}.\mathrm{55}\Rightarrow\boldsymbol{{A}}=+\mathrm{0}.\mathrm{6}\boldsymbol{{i}}}\end{cases} \\ $$$$\boldsymbol{{cosB}}=\frac{{a}^{\mathrm{2}} +{c}^{\mathrm{2}} −{b}^{\mathrm{2}} }{\mathrm{2}{ac}}=\frac{\mathrm{1}+\mathrm{16}−\mathrm{4}}{\mathrm{2}×\mathrm{1}×\mathrm{4}}=\frac{\mathrm{13}}{\mathrm{8}} \\ $$$$\frac{{e}^{{iB}} +{e}^{−{iB}} }{\mathrm{2}}=\frac{\mathrm{13}}{\mathrm{8}}\:\:\:\overset{{e}^{{iB}} ={s}} {\Rightarrow}\:\mathrm{8}\left({s}+\frac{\mathrm{1}}{{s}}\right)=\mathrm{13} \\ $$$$\Rightarrow\mathrm{8}{s}^{\mathrm{2}} −\mathrm{13}{s}+\mathrm{8}=\mathrm{0}\Rightarrow{s}=\frac{\mathrm{13}\pm\sqrt{\mathrm{169}−\mathrm{4}×\mathrm{64}}}{\mathrm{16}} \\ $$$$\Rightarrow{s}=.\mathrm{81}\pm.\mathrm{58}\boldsymbol{{i}}=\sqrt{.\mathrm{81}^{\mathrm{2}} +.\mathrm{58}^{\mathrm{2}} }\left(\frac{.\mathrm{81}\pm.\mathrm{58}{i}}{\:\sqrt{.\mathrm{81}^{\mathrm{2}} +.\mathrm{58}^{\mathrm{2}} }}\right)= \\ $$$$=.\mathrm{99}\left(.\mathrm{81}\pm.\mathrm{58}{i}\right)#\begin{cases}{{e}^{{icos}^{−\mathrm{1}} \left(.\mathrm{81}\right)} }\\{{e}^{−{icos}^{−\mathrm{1}} \left(.\mathrm{81}\right)} }\end{cases} \\ $$$$\Rightarrow{B}=\begin{cases}{{cos}^{−\mathrm{1}} \left(.\mathrm{81}\right)=\mathrm{35}.\mathrm{90}^{\bullet} }\\{−{cos}^{−\mathrm{1}} \left(.\mathrm{81}\right)=−\mathrm{35}.\mathrm{90}^{\bullet} }\end{cases} \\ $$$${cosC}=\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} −{c}^{\mathrm{2}} }{\mathrm{2}{ab}}=\frac{\mathrm{1}+\mathrm{4}−\mathrm{16}}{\mathrm{2}×\mathrm{1}×\mathrm{2}}=−\frac{\mathrm{11}}{\mathrm{4}} \\ $$$$\Rightarrow\frac{{e}^{{iC}} +{e}^{−{iC}} }{\mathrm{2}}=−\frac{\mathrm{11}}{\mathrm{4}}\:\:\overset{{e}^{{iC}} ={p}} {\Rightarrow}\Rightarrow\mathrm{4}\left({p}+\frac{\mathrm{1}}{{p}}\right)=−\mathrm{11} \\ $$$$\Rightarrow\mathrm{4}{p}^{\mathrm{2}} +\mathrm{11}{p}+\mathrm{4}=\mathrm{0}\Rightarrow{p}=\frac{−\mathrm{11}\pm\sqrt{\mathrm{121}−\mathrm{64}}}{\mathrm{8}}= \\ $$$$\Rightarrow{p}=−.\mathrm{86},−\mathrm{4}.\mathrm{6} \\ $$$$\Rightarrow{e}^{{iC}} =−.\mathrm{86}=.\mathrm{86}{i}^{\mathrm{2}} \Rightarrow{iC}={ln}.\mathrm{86}+\mathrm{2}{lni}= \\ $$$$=−.\mathrm{15}+{i}\pi\Rightarrow{C}=+.\mathrm{15}{i}+\pi \\ $$$${e}^{{iC}} =−\mathrm{4}.\mathrm{6}=\mathrm{4}.\mathrm{6}{i}^{\mathrm{2}} \Rightarrow{iC}={ln}\mathrm{4}.\mathrm{6}+\mathrm{2}{lni}= \\ $$$$=\mathrm{1}.\mathrm{52}+{i}\pi\Rightarrow{C}=−\mathrm{1}.\mathrm{52}{i}+\pi \\ $$$$\Rightarrow{C}=\begin{cases}{.\mathrm{15}\boldsymbol{{i}}+\boldsymbol{\pi}}\\{−\mathrm{1}.\mathrm{52}\boldsymbol{{i}}+\boldsymbol{\pi}}\end{cases} \\ $$$$\left({i}=\mathrm{0}+\mathrm{1}×{i}={cos}\mathrm{90}+{isin}\mathrm{90}={e}^{{i}\frac{\pi}{\mathrm{2}}} \Rightarrow{lni}={i}\frac{\pi}{\mathrm{2}}\right) \\ $$$$\Rightarrow{A}=\begin{cases}{−.\mathrm{6}\boldsymbol{{i}}}\\{+.\mathrm{6}\boldsymbol{{i}}}\end{cases},{B}=\begin{cases}{\mathrm{35}.\mathrm{90}^{\bullet} }\\{−\mathrm{35}.\mathrm{90}^{\bullet} }\end{cases},{C}=\begin{cases}{.\mathrm{15}\boldsymbol{{i}}+\mathrm{180}}\\{−\mathrm{1}.\mathrm{52}+\mathrm{180}}\end{cases} \\ $$$$\Rightarrow{A}+{B}+{C}= \\ $$$$=−.\mathrm{6}{i}+\mathrm{35}.\mathrm{90}+.\mathrm{15}{i}+\mathrm{180}=−.\mathrm{45}\boldsymbol{{i}}+\mathrm{215}.\mathrm{90} \\ $$$$=.\mathrm{6}\boldsymbol{{i}}−\mathrm{35}.\mathrm{90}−\mathrm{1}.\mathrm{52}\boldsymbol{{i}}+\mathrm{180}=−.\mathrm{88}\boldsymbol{{i}}+\mathrm{144}.\mathrm{1} \\ $$$$=−.\mathrm{6}\boldsymbol{{i}}+\mathrm{35}.\mathrm{90}−\mathrm{1}.\mathrm{52}\boldsymbol{{i}}+\mathrm{180}=−\mathrm{2}.\mathrm{12}\boldsymbol{{i}}+\mathrm{215}.\mathrm{90} \\ $$$$=−.\mathrm{6}\boldsymbol{{i}}−\mathrm{35}.\mathrm{90}+.\mathrm{15}\boldsymbol{{i}}+\mathrm{180}=−.\mathrm{45}\boldsymbol{{i}}+\mathrm{144}.\mathrm{1} \\ $$$$=−.\mathrm{6}\boldsymbol{{i}}−\mathrm{35}.\mathrm{90}−\mathrm{1}.\mathrm{52}+\mathrm{180}=−\mathrm{2}.\mathrm{12}\boldsymbol{{i}}+\mathrm{144}.\mathrm{1} \\ $$$$=……………. \\ $$$$\boldsymbol{{there}}\:\boldsymbol{{is}}\:\boldsymbol{{no}}\:\boldsymbol{{case}}\:\boldsymbol{{for}}:{A}+{B}+{C}=\mathrm{180}. \\ $$
Commented by prakash jain last updated on 14/Jun/17
cos^(−1) (−((11)/4))+cos^(−1) (((19)/(16)))+cos^(−1) (((13)/8))=π I think you mixed degrees and radians somewhere. I think theory of triangle remains valid even with complex sides and angles.
$$\mathrm{cos}^{−\mathrm{1}} \left(−\frac{\mathrm{11}}{\mathrm{4}}\right)+\mathrm{cos}^{−\mathrm{1}} \left(\frac{\mathrm{19}}{\mathrm{16}}\right)+\mathrm{cos}^{−\mathrm{1}} \left(\frac{\mathrm{13}}{\mathrm{8}}\right)=\pi \\ $$$$\mathrm{I}\:\mathrm{think}\:\mathrm{you}\:\mathrm{mixed}\:\mathrm{degrees}\:\mathrm{and}\:\mathrm{radians} \\ $$$$\mathrm{somewhere}. \\ $$$$\mathrm{I}\:\mathrm{think}\:\mathrm{theory}\:\mathrm{of}\:\mathrm{triangle}\:\mathrm{remains} \\ $$$$\mathrm{valid}\:\mathrm{even}\:\mathrm{with}\:\mathrm{complex}\:\mathrm{sides} \\ $$$$\mathrm{and}\:\mathrm{angles}. \\ $$
Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 15/Jun/17
mr prakash! cos^(−1) (((19)/(16))),not a valid angle with primary elements of geometry and trigonometry.
$${mr}\:{prakash}!\:{cos}^{−\mathrm{1}} \left(\frac{\mathrm{19}}{\mathrm{16}}\right),{not}\:{a}\:{valid}\: \\ $$$${angle}\:{with}\:{primary}\:{elements}\:{of}\: \\ $$$${geometry}\:{and}\:{trigonometry}. \\ $$
Commented by prakash jain last updated on 18/Jun/17
Yes. I started this question by calling these triangle complex triangles.
$$\mathrm{Yes}.\:\mathrm{I}\:\mathrm{started}\:\mathrm{this}\:\mathrm{question}\:\mathrm{by} \\ $$$$\mathrm{calling}\:\mathrm{these}\:\mathrm{triangle}\:\mathrm{complex} \\ $$$$\mathrm{triangles}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *