Menu Close

In-ABC-sides-a-b-c-are-roots-of-the-equation-x-3-px-2-qx-r-0-Prove-that-area-of-ABC-is-1-4-p-4pq-p-3-8r-

Tinku Tara 0 Comments
Pin




Question Number 15893 by Tinkutara last updated on 15/Jun/17
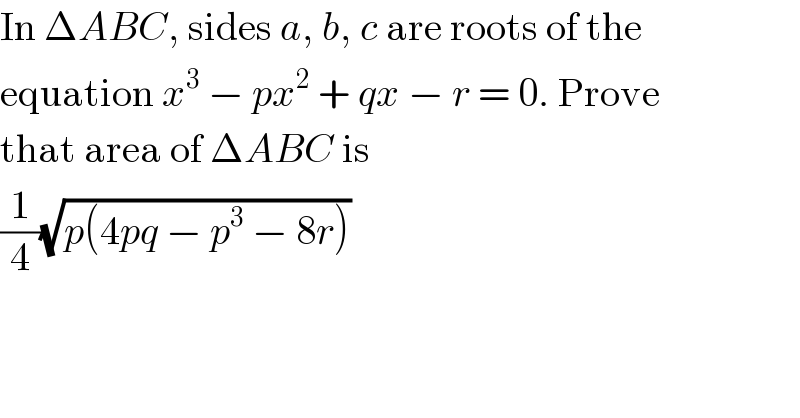
In ΔABC, sides a, b, c are roots of the equation x^3 − px^2 + qx − r = 0. Prove that area of ΔABC is (1/4)(√(p(4pq − p^3 − 8r)))
$$\mathrm{In}\:\Delta{ABC},\:\mathrm{sides}\:{a},\:{b},\:{c}\:\mathrm{are}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{equation}\:{x}^{\mathrm{3}} \:−\:{px}^{\mathrm{2}} \:+\:{qx}\:−\:{r}\:=\:\mathrm{0}.\:\mathrm{Prove} \\ $$$$\mathrm{that}\:\mathrm{area}\:\mathrm{of}\:\Delta{ABC}\:\mathrm{is} \\ $$$$\frac{\mathrm{1}}{\mathrm{4}}\sqrt{{p}\left(\mathrm{4}{pq}\:−\:{p}^{\mathrm{3}} \:−\:\mathrm{8}{r}\right)} \\ $$
Answered by RasheedSoomro last updated on 15/Jun/17
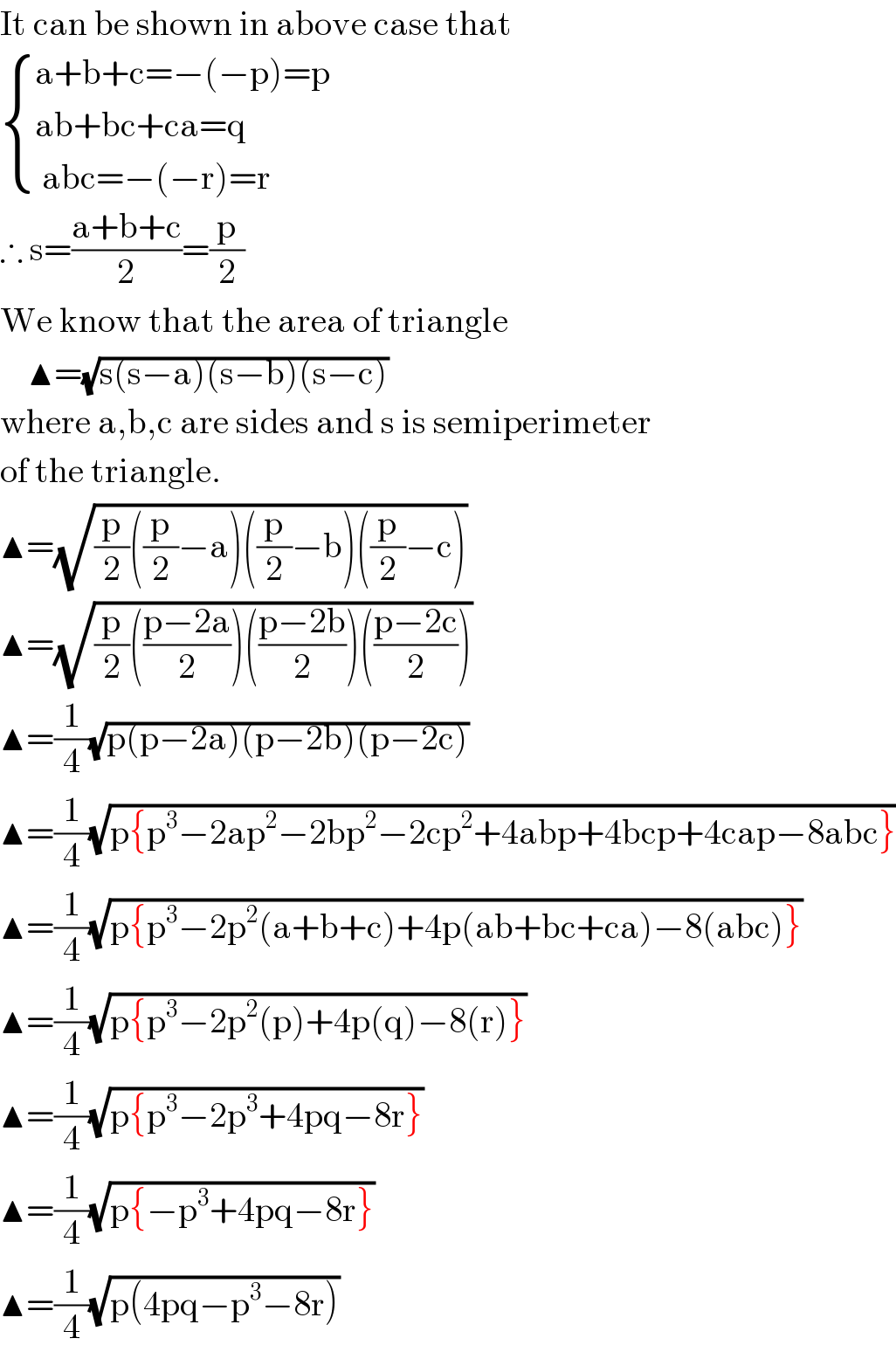
It can be shown in above case that { ((a+b+c=−(−p)=p )),((ab+bc+ca=q)),(( abc=−(−r)=r)) :} ∴ s=((a+b+c)/2)=(p/2) We know that the area of triangle ▲=(√(s(s−a)(s−b)(s−c))) where a,b,c are sides and s is semiperimeter of the triangle. ▲=(√((p/2)((p/2)−a)((p/2)−b)((p/2)−c))) ▲=(√((p/2)(((p−2a)/2))(((p−2b)/2))(((p−2c)/2)))) ▲=(1/4)(√(p(p−2a)(p−2b)(p−2c))) ▲=(1/4)(√(p{p^3 −2ap^2 −2bp^2 −2cp^2 +4abp+4bcp+4cap−8abc})) ▲=(1/4)(√(p{p^3 −2p^2 (a+b+c)+4p(ab+bc+ca)−8(abc)})) ▲=(1/4)(√(p{p^3 −2p^2 (p)+4p(q)−8(r)})) ▲=(1/4)(√(p{p^3 −2p^3 +4pq−8r})) ▲=(1/4)(√(p{−p^3 +4pq−8r})) ▲=(1/4)(√(p(4pq−p^3 −8r)))
$$\mathrm{It}\:\mathrm{can}\:\mathrm{be}\:\mathrm{shown}\:\mathrm{in}\:\mathrm{above}\:\mathrm{case}\:\mathrm{that} \\ $$$$\begin{cases}{\mathrm{a}+\mathrm{b}+\mathrm{c}=−\left(−\mathrm{p}\right)=\mathrm{p}\:\:}\\{\mathrm{ab}+\mathrm{bc}+\mathrm{ca}=\mathrm{q}}\\{\:\mathrm{abc}=−\left(−\mathrm{r}\right)=\mathrm{r}}\end{cases} \\ $$$$\therefore\:\mathrm{s}=\frac{\mathrm{a}+\mathrm{b}+\mathrm{c}}{\mathrm{2}}=\frac{\mathrm{p}}{\mathrm{2}} \\ $$$$\mathrm{We}\:\mathrm{know}\:\mathrm{that}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{triangle} \\ $$$$\:\:\:\:\blacktriangle=\sqrt{\mathrm{s}\left(\mathrm{s}−\mathrm{a}\right)\left(\mathrm{s}−\mathrm{b}\right)\left(\mathrm{s}−\mathrm{c}\right)} \\ $$$$\mathrm{where}\:\mathrm{a},\mathrm{b},\mathrm{c}\:\mathrm{are}\:\mathrm{sides}\:\mathrm{and}\:\mathrm{s}\:\mathrm{is}\:\mathrm{semiperimeter} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{triangle}. \\ $$$$\blacktriangle=\sqrt{\frac{\mathrm{p}}{\mathrm{2}}\left(\frac{\mathrm{p}}{\mathrm{2}}−\mathrm{a}\right)\left(\frac{\mathrm{p}}{\mathrm{2}}−\mathrm{b}\right)\left(\frac{\mathrm{p}}{\mathrm{2}}−\mathrm{c}\right)} \\ $$$$\blacktriangle=\sqrt{\frac{\mathrm{p}}{\mathrm{2}}\left(\frac{\mathrm{p}−\mathrm{2a}}{\mathrm{2}}\right)\left(\frac{\mathrm{p}−\mathrm{2b}}{\mathrm{2}}\right)\left(\frac{\mathrm{p}−\mathrm{2c}}{\mathrm{2}}\right)} \\ $$$$\blacktriangle=\frac{\mathrm{1}}{\mathrm{4}}\sqrt{\mathrm{p}\left(\mathrm{p}−\mathrm{2a}\right)\left(\mathrm{p}−\mathrm{2b}\right)\left(\mathrm{p}−\mathrm{2c}\right)} \\ $$$$\blacktriangle=\frac{\mathrm{1}}{\mathrm{4}}\sqrt{\mathrm{p}\left\{\mathrm{p}^{\mathrm{3}} −\mathrm{2ap}^{\mathrm{2}} −\mathrm{2bp}^{\mathrm{2}} −\mathrm{2cp}^{\mathrm{2}} +\mathrm{4abp}+\mathrm{4bcp}+\mathrm{4cap}−\mathrm{8abc}\right\}} \\ $$$$\blacktriangle=\frac{\mathrm{1}}{\mathrm{4}}\sqrt{\mathrm{p}\left\{\mathrm{p}^{\mathrm{3}} −\mathrm{2p}^{\mathrm{2}} \left(\mathrm{a}+\mathrm{b}+\mathrm{c}\right)+\mathrm{4p}\left(\mathrm{ab}+\mathrm{bc}+\mathrm{ca}\right)−\mathrm{8}\left(\mathrm{abc}\right)\right\}} \\ $$$$\blacktriangle=\frac{\mathrm{1}}{\mathrm{4}}\sqrt{\mathrm{p}\left\{\mathrm{p}^{\mathrm{3}} −\mathrm{2p}^{\mathrm{2}} \left(\mathrm{p}\right)+\mathrm{4p}\left(\mathrm{q}\right)−\mathrm{8}\left(\mathrm{r}\right)\right\}} \\ $$$$\blacktriangle=\frac{\mathrm{1}}{\mathrm{4}}\sqrt{\mathrm{p}\left\{\mathrm{p}^{\mathrm{3}} −\mathrm{2p}^{\mathrm{3}} +\mathrm{4pq}−\mathrm{8r}\right\}} \\ $$$$\blacktriangle=\frac{\mathrm{1}}{\mathrm{4}}\sqrt{\mathrm{p}\left\{−\mathrm{p}^{\mathrm{3}} +\mathrm{4pq}−\mathrm{8r}\right\}} \\ $$$$\blacktriangle=\frac{\mathrm{1}}{\mathrm{4}}\sqrt{\mathrm{p}\left(\mathrm{4pq}−\mathrm{p}^{\mathrm{3}} −\mathrm{8r}\right)} \\ $$
Commented by Tinkutara last updated on 15/Jun/17
Thanks Sir!
$$\mathrm{Thanks}\:\mathrm{Sir}! \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *