Question Number 82018 by TawaTawa last updated on 17/Feb/20
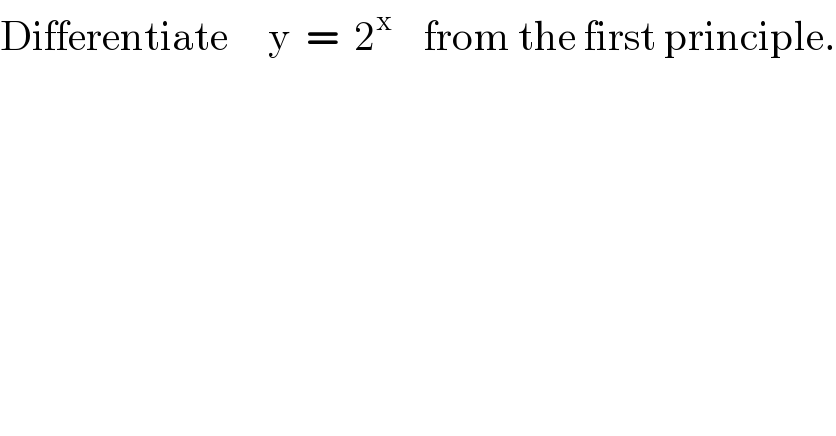
$$\mathrm{Differentiate}\:\:\:\:\:\mathrm{y}\:\:=\:\:\mathrm{2}^{\mathrm{x}} \:\:\:\:\mathrm{from}\:\mathrm{the}\:\mathrm{first}\:\mathrm{principle}. \\ $$
Commented by john santu last updated on 17/Feb/20
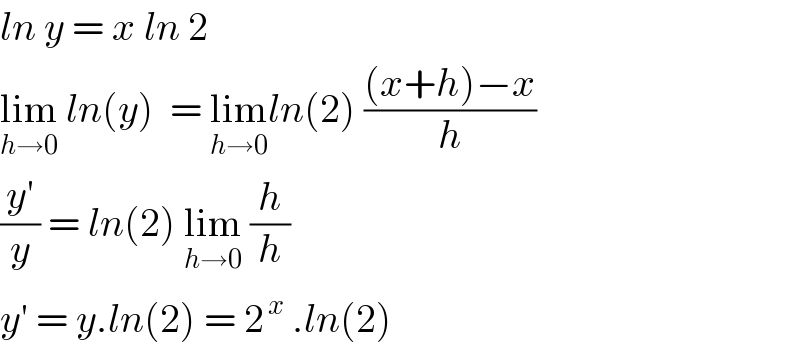
$${ln}\:{y}\:=\:{x}\:{ln}\:\mathrm{2} \\ $$$$\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:{ln}\left({y}\right)\:\:=\:\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}{ln}\left(\mathrm{2}\right)\:\frac{\left({x}+{h}\right)−{x}}{{h}} \\ $$$$\frac{{y}'}{{y}}\:=\:{ln}\left(\mathrm{2}\right)\:\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{h}}{{h}} \\ $$$${y}'\:=\:{y}.{ln}\left(\mathrm{2}\right)\:=\:\mathrm{2}^{\:{x}} \:.{ln}\left(\mathrm{2}\right) \\ $$
Commented by TawaTawa last updated on 17/Feb/20
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Answered by TANMAY PANACEA last updated on 17/Feb/20
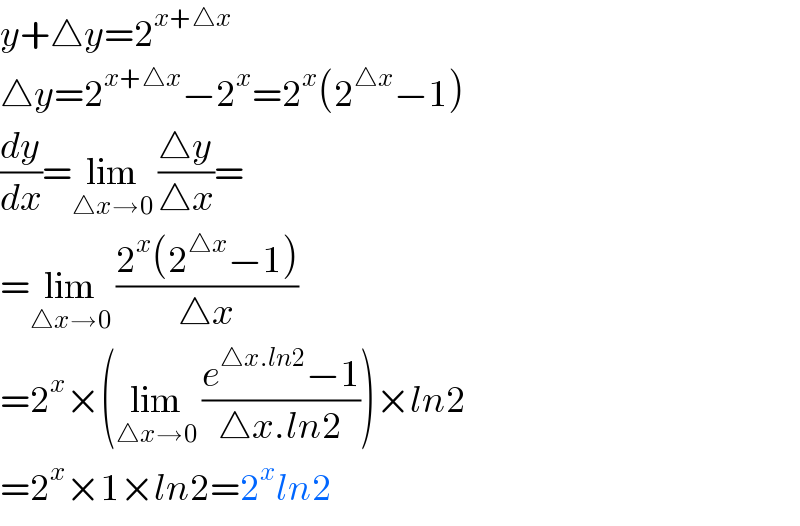
$${y}+\bigtriangleup{y}=\mathrm{2}^{{x}+\bigtriangleup{x}} \\ $$$$\bigtriangleup{y}=\mathrm{2}^{{x}+\bigtriangleup{x}} −\mathrm{2}^{{x}} =\mathrm{2}^{{x}} \left(\mathrm{2}^{\bigtriangleup{x}} −\mathrm{1}\right) \\ $$$$\frac{{dy}}{{dx}}=\underset{\bigtriangleup{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\bigtriangleup{y}}{\bigtriangleup{x}}= \\ $$$$=\underset{\bigtriangleup{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{2}^{{x}} \left(\mathrm{2}^{\bigtriangleup{x}} −\mathrm{1}\right)}{\bigtriangleup{x}} \\ $$$$=\mathrm{2}^{{x}} ×\left(\underset{\bigtriangleup{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{e}^{\bigtriangleup{x}.{ln}\mathrm{2}} −\mathrm{1}}{\bigtriangleup{x}.{ln}\mathrm{2}}\right)×{ln}\mathrm{2} \\ $$$$=\mathrm{2}^{{x}} ×\mathrm{1}×{ln}\mathrm{2}=\mathrm{2}^{{x}} {ln}\mathrm{2} \\ $$
Commented by TawaTawa last updated on 17/Feb/20
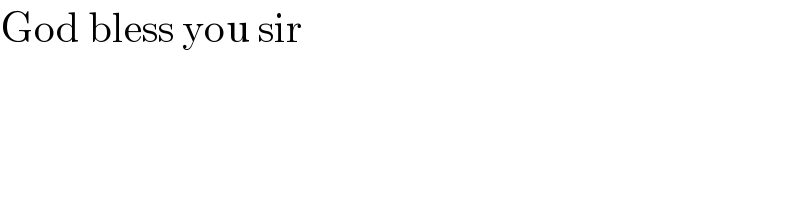
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
Answered by mr W last updated on 17/Feb/20
![y′=lim_(h→0) ((2^(x+h) −2^x )/h) =2^x lim_(h→0) ((2^h −1)/h) =2^x lim_(h→0) ((e^(hln 2) −1)/h) =2^x lim_(h→0) (([1+hln 2+(((hln 2)^2 )/(2!))+(((hln 2)^3 )/(3!))+...]−1)/h) =2^x lim_(h→0) [ln 2+((h(ln 2)^2 )/(2!))+((h^2 (ln 2)^3 )/(3!))+...] =2^x ln 2](https://www.tinkutara.com/question/Q82033.png)
$${y}'=\underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{2}^{{x}+{h}} −\mathrm{2}^{{x}} }{{h}} \\ $$$$=\mathrm{2}^{{x}} \underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{2}^{{h}} −\mathrm{1}}{{h}} \\ $$$$=\mathrm{2}^{{x}} \underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{{h}\mathrm{ln}\:\mathrm{2}} −\mathrm{1}}{{h}} \\ $$$$=\mathrm{2}^{{x}} \underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left[\mathrm{1}+{h}\mathrm{ln}\:\mathrm{2}+\frac{\left({h}\mathrm{ln}\:\mathrm{2}\right)^{\mathrm{2}} }{\mathrm{2}!}+\frac{\left({h}\mathrm{ln}\:\mathrm{2}\right)^{\mathrm{3}} }{\mathrm{3}!}+…\right]−\mathrm{1}}{{h}} \\ $$$$=\mathrm{2}^{{x}} \underset{{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\left[\mathrm{ln}\:\mathrm{2}+\frac{{h}\left(\mathrm{ln}\:\mathrm{2}\right)^{\mathrm{2}} }{\mathrm{2}!}+\frac{{h}^{\mathrm{2}} \left(\mathrm{ln}\:\mathrm{2}\right)^{\mathrm{3}} }{\mathrm{3}!}+…\right] \\ $$$$=\mathrm{2}^{{x}} \mathrm{ln}\:\mathrm{2} \\ $$
Commented by TawaTawa last updated on 17/Feb/20
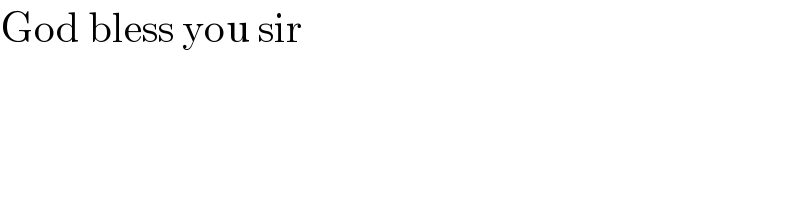
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
