Question Number 148364 by mathdanisur last updated on 27/Jul/21

Commented by dumitrel last updated on 27/Jul/21
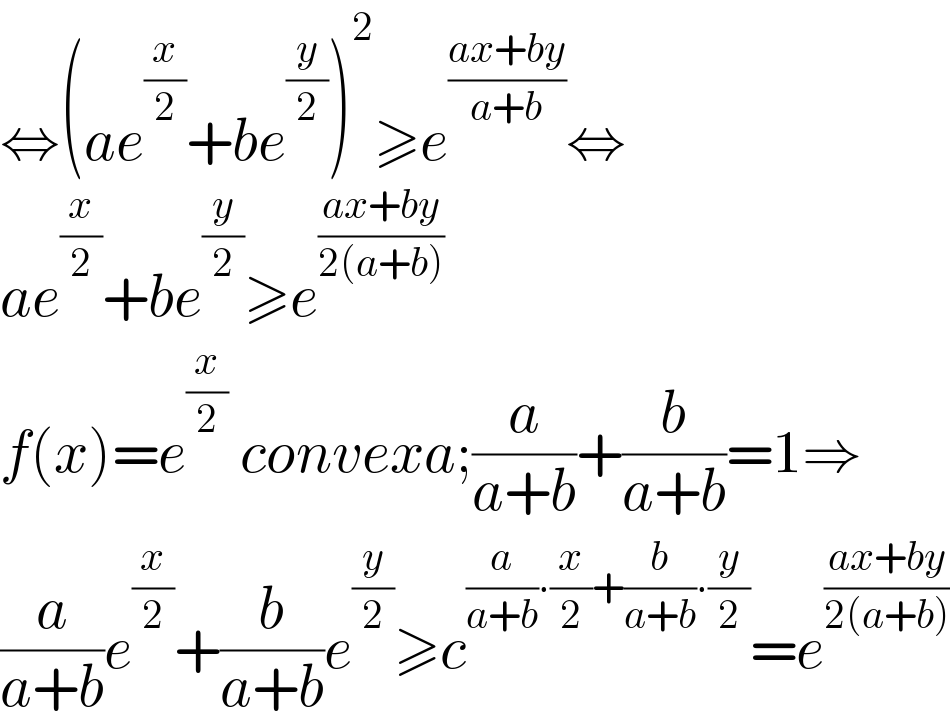
$$\Leftrightarrow\left({ae}^{\frac{{x}}{\mathrm{2}}} +{be}^{\frac{{y}}{\mathrm{2}}} \right)^{\mathrm{2}} \geqslant{e}^{\frac{{ax}+{by}}{{a}+{b}}} \Leftrightarrow \\ $$$${ae}^{\frac{{x}}{\mathrm{2}}} +{be}^{\frac{{y}}{\mathrm{2}}} \geqslant{e}^{\frac{{ax}+{by}}{\mathrm{2}\left({a}+{b}\right)}} \\ $$$${f}\left({x}\right)={e}^{\frac{{x}}{\mathrm{2}}} \:{convexa};\frac{{a}}{{a}+{b}}+\frac{{b}}{{a}+{b}}=\mathrm{1}\Rightarrow \\ $$$$\frac{{a}}{{a}+{b}}{e}^{\frac{{x}}{\mathrm{2}}} +\frac{{b}}{{a}+{b}}{e}^{\frac{{y}}{\mathrm{2}}} \geqslant{c}^{\frac{{a}}{{a}+{b}}\centerdot\frac{{x}}{\mathrm{2}}+\frac{{b}}{{a}+{b}}\centerdot\frac{{y}}{\mathrm{2}}} ={e}^{\frac{{ax}+{by}}{\mathrm{2}\left({a}+{b}\right)}} \\ $$
Commented by mathdanisur last updated on 27/Jul/21

$${Thank}\:{you}\:{Ser} \\ $$
