Question Number 17348 by Tinkutara last updated on 04/Jul/17
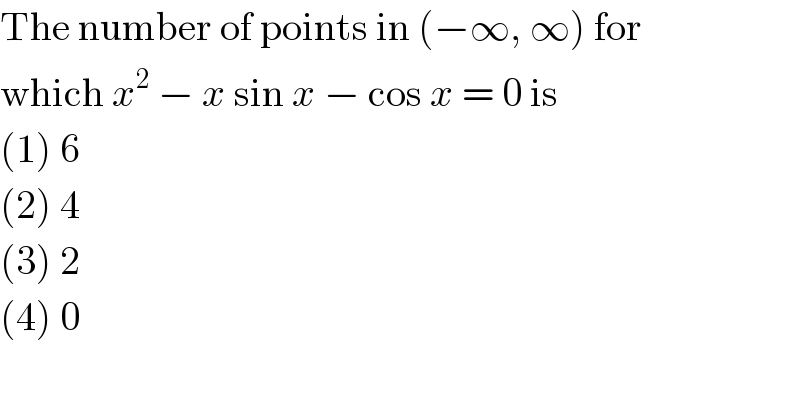
$$\mathrm{The}\:\mathrm{number}\:\mathrm{of}\:\mathrm{points}\:\mathrm{in}\:\left(−\infty,\:\infty\right)\:\mathrm{for} \\ $$$$\mathrm{which}\:{x}^{\mathrm{2}} \:−\:{x}\:\mathrm{sin}\:{x}\:−\:\mathrm{cos}\:{x}\:=\:\mathrm{0}\:\mathrm{is} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{6} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{4} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{2} \\ $$$$\left(\mathrm{4}\right)\:\mathrm{0} \\ $$
Answered by ajfour last updated on 04/Jul/17

$$\left(\mathrm{3}\right)\:\mathrm{2} \\ $$$$\:\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} −\mathrm{xsin}\:\mathrm{x}−\mathrm{cos}\:\mathrm{x} \\ $$$$\:\:\:\:\:\mathrm{f}\left(\mathrm{0}\right)=−\mathrm{1} \\ $$$$\:\:\mathrm{f}\:'\left(\mathrm{x}\right)=\mathrm{2x}−\mathrm{xcos}\:\mathrm{x} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{x}\left(\mathrm{2}−\mathrm{cos}\:\mathrm{x}\right) \\ $$$$\:\:\mathrm{as}\:\mathrm{x}\rightarrow\:−\infty\:,\:\mathrm{f}\left(\mathrm{x}\right)\rightarrow\infty \\ $$$$\:\:\mathrm{only}\:\mathrm{decreasing}\:\mathrm{for}\:\mathrm{x}<\mathrm{0} \\ $$$$\:\mathrm{stops}\:\mathrm{decreasing}\:\mathrm{when}\:\mathrm{x}=\mathrm{0} \\ $$$$\:\mathrm{and}\:\mathrm{by}\:\mathrm{this}\:\mathrm{time}\:\mathrm{f}\left(\mathrm{x}\right)=−\mathrm{1} \\ $$$$\Rightarrow\:\mathrm{crossed}\:\mathrm{x}-\mathrm{axis}\:\mathrm{once} \\ $$$$\:\:\:\:\:\:\:\mathrm{x}=\mathrm{0}\:\mathrm{is}\:\mathrm{the}\:\mathrm{minimum} \\ $$$$\:\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{starts}\:\mathrm{increasing}\:\mathrm{from}\: \\ $$$$\mathrm{f}\left(\mathrm{0}\right)=−\mathrm{1}\:\mathrm{and}\:\mathrm{keeps}\:\mathrm{increasing} \\ $$$$\:\mathrm{hereafter}\:\mathrm{so}\:\mathrm{crosses}\:\mathrm{x}-\mathrm{axis}\:\mathrm{just} \\ $$$$\mathrm{once}\:\mathrm{more}. \\ $$
Commented by Tinkutara last updated on 05/Jul/17

$$\mathrm{Thanks}\:\mathrm{Sir}! \\ $$
Answered by mrW1 last updated on 04/Jul/17

$$\mathrm{y}=\mathrm{f}\left(\mathrm{x}\right)={x}^{\mathrm{2}} \:−\:{x}\:\mathrm{sin}\:{x}\:−\:\mathrm{cos}\:{x} \\ $$$$\mathrm{f}\left(−\mathrm{x}\right)=\left(−\mathrm{x}\right)^{\mathrm{2}} −\left(−\mathrm{x}\right)\mathrm{sin}\:\left(−\mathrm{x}\right)−\mathrm{cos}\:\left(−\mathrm{x}\right) \\ $$$$=\mathrm{x}^{\mathrm{2}} −\mathrm{x}\:\mathrm{sin}\:\mathrm{x}−\mathrm{cos}\:\mathrm{x} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{is}\:\mathrm{symmetric}. \\ $$$$\mathrm{f}\left(\mathrm{0}\right)=−\mathrm{1} \\ $$$$\underset{{x}\rightarrow+\infty} {\mathrm{lim}}\:\mathrm{f}\left(\mathrm{x}\right)=+\infty \\ $$$$\Rightarrow\mathrm{there}\:\mathrm{is}\:\mathrm{at}\:\mathrm{least}\:\mathrm{one}\:\mathrm{point}\:\mathrm{for}\:\mathrm{x}>\mathrm{0}\: \\ $$$$\mathrm{and}\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{0} \\ $$$$\mathrm{f}'\left(\mathrm{x}\right)=\mathrm{2x}−\mathrm{sin}\:\mathrm{x}−\mathrm{xcos}\:\mathrm{x}+\mathrm{sin}\:\mathrm{x}=\mathrm{x}\left(\mathrm{2}−\mathrm{cos}\:\mathrm{x}\right) \\ $$$$\mathrm{for}\:\mathrm{x}>\mathrm{0}\:\Rightarrow\mathrm{f}'\left(\mathrm{x}\right)>\mathrm{0} \\ $$$$\Rightarrow\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{is}\:\mathrm{increasing}\:\mathrm{for}\:\mathrm{x}>\mathrm{0} \\ $$$$\Rightarrow\mathrm{there}\:\mathrm{is}\:\mathrm{only}\:\mathrm{one}\:\mathrm{point}\:\mathrm{for}\:\mathrm{x}\in\left(\mathrm{0},+\infty\right)\:\mathrm{with} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{0} \\ $$$$ \\ $$$$\mathrm{due}\:\mathrm{to}\:\mathrm{symmetry}\: \\ $$$$\mathrm{there}\:\mathrm{are}\:\mathrm{2}\:\mathrm{point2}\:\mathrm{for}\:\mathrm{x}\in\left(−\infty,+\infty\right)\:\mathrm{with} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{0} \\ $$$$ \\ $$$$\Rightarrow\mathrm{answer}\:\left(\mathrm{3}\right)\:\mathrm{is}\:\mathrm{right}. \\ $$
Commented by Tinkutara last updated on 05/Jul/17

$$\mathrm{Thanks}\:\mathrm{Sir}! \\ $$
Answered by b.e.h.i.8.3.4.17@gmail.com last updated on 06/Jul/17
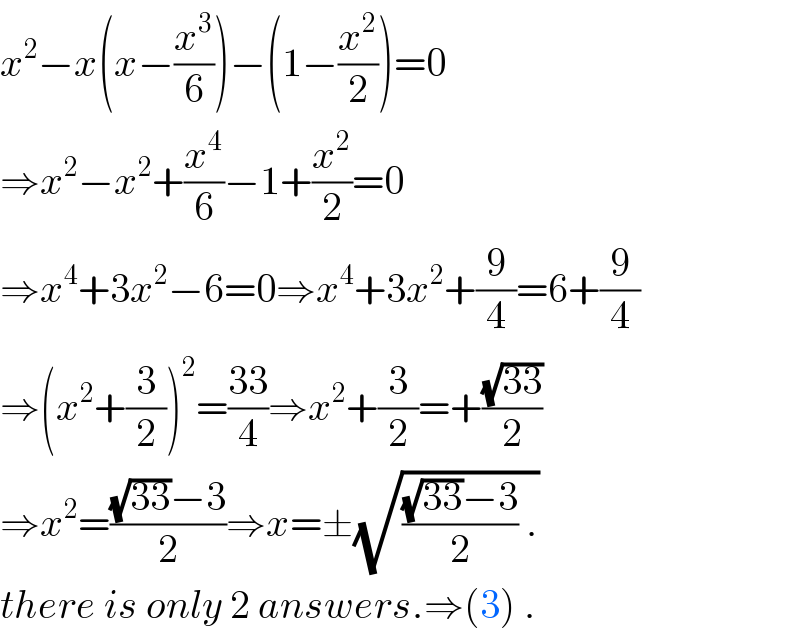
$${x}^{\mathrm{2}} −{x}\left({x}−\frac{{x}^{\mathrm{3}} }{\mathrm{6}}\right)−\left(\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{2}}\right)=\mathrm{0} \\ $$$$\Rightarrow{x}^{\mathrm{2}} −{x}^{\mathrm{2}} +\frac{{x}^{\mathrm{4}} }{\mathrm{6}}−\mathrm{1}+\frac{{x}^{\mathrm{2}} }{\mathrm{2}}=\mathrm{0} \\ $$$$\Rightarrow{x}^{\mathrm{4}} +\mathrm{3}{x}^{\mathrm{2}} −\mathrm{6}=\mathrm{0}\Rightarrow{x}^{\mathrm{4}} +\mathrm{3}{x}^{\mathrm{2}} +\frac{\mathrm{9}}{\mathrm{4}}=\mathrm{6}+\frac{\mathrm{9}}{\mathrm{4}} \\ $$$$\Rightarrow\left({x}^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{2}}\right)^{\mathrm{2}} =\frac{\mathrm{33}}{\mathrm{4}}\Rightarrow{x}^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{2}}=+\frac{\sqrt{\mathrm{33}}}{\mathrm{2}} \\ $$$$\Rightarrow{x}^{\mathrm{2}} =\frac{\sqrt{\mathrm{33}}−\mathrm{3}}{\mathrm{2}}\Rightarrow{x}=\pm\sqrt{\frac{\sqrt{\mathrm{33}}−\mathrm{3}}{\mathrm{2}}\:.} \\ $$$${there}\:{is}\:{only}\:\mathrm{2}\:{answers}.\Rightarrow\left(\mathrm{3}\right)\:. \\ $$
Commented by Tinkutara last updated on 07/Jul/17

$$\mathrm{Can}\:\mathrm{you}\:\mathrm{explain}\:\mathrm{the}\:\mathrm{first}\:\mathrm{line}? \\ $$
Commented by alex041103 last updated on 07/Jul/17

$$\mathrm{This}\:\mathrm{is}\:\mathrm{wrong}. \\ $$$$\mathrm{He}\:\mathrm{tried}\:\mathrm{to}\:\mathrm{use}\:\mathrm{the}\:\mathrm{Taylor}\:\mathrm{series} \\ $$$$\mathrm{expansion}\:\mathrm{for}\:\mathrm{sin}\:\mathrm{x}\:\mathrm{and}\:\mathrm{cos}\:\mathrm{x}.\: \\ $$$$\mathrm{They}\:\mathrm{have}\:\mathrm{infinatly}\:\mathrm{many}\:\mathrm{terms},\:\mathrm{but} \\ $$$$\mathrm{he}\:\mathrm{uses}\:\mathrm{only}\:\mathrm{two}\:\mathrm{of}\:\mathrm{them}. \\ $$$$\mathrm{This}\:\mathrm{is}\:\mathrm{an}\:\mathrm{aproximation}! \\ $$$$\mathrm{Here}\:\mathrm{are}\:\mathrm{the}\:\mathrm{expansions}\:\mathrm{for}\:\mathrm{sine}\:\mathrm{and}\:\mathrm{cosine}: \\ $$$${sin}\:{x}\:=\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(−\mathrm{1}\right)^{{n}} \:\frac{{x}^{\mathrm{2}{n}+\mathrm{1}} }{\left(\mathrm{2}{n}+\mathrm{1}\right)!} \\ $$$${cos}\:{x}\:=\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(−\mathrm{1}\right)^{{n}} \frac{{x}^{\mathrm{2}{n}} }{\left(\mathrm{2}{n}\right)!} \\ $$
